The end of a chain of length L and mass per unit length ρ, which is piled up on a horizontally platform is lifted vertically with a constant velocity u by a variable force F. Find F as a function of height x of the end above platform:
ρ(gx+2u2)
ρ(2gx+ρu2)
ρ(gx+u2)
ρ(u2−gx)
To solve the problem of finding the force F as a function of the height x of the end of the chain above the platform, we can follow these steps:
Step 1: Understand the system
We have a chain of length L and mass per unit length ρ. When we lift the chain vertically with a constant velocity u, we need to consider the forces acting on the chain.
Hint: Identify the forces acting on the chain when it is lifted.
Step 2: Identify the forces
When lifting the chain, the force F is applied upwards, while the weight of the chain acts downwards. The weight of the chain that has been lifted to a height x is given by:
Weight=mg=ρxg
where g is the acceleration due to gravity.
Hint: Write down the expression for the weight of the lifted portion of the chain.
Step 3: Consider the rate of change of momentum
Since the chain is lifted with a constant velocity u, the rate of change of momentum must also be considered. The momentum p of the chain being lifted can be expressed as:
p=mu=ρu⋅x
The rate of change of momentum dpdt when lifting the chain can be calculated as:
dpdt=ddt(ρux)=ρudxdt=ρuu=ρu2
since dxdt=u.
Hint: Calculate the rate of change of momentum for the chain being lifted.
Step 4: Apply Newton's second law
According to Newton's second law, the net force Fnet acting on the chain is given by:
Fnet=F−Weight
Thus, we can write:
F−ρxg=dpdt
Substituting the expression for dpdt:
F−ρxg=ρu2
Hint: Set up the equation using Newton's second law and substitute for the rate of change of momentum.
Step 5: Solve for the force F
Rearranging the equation gives:
F=ρxg+ρu2
This expression shows the force F as a function of the height x.
Hint: Isolate F to express it in terms of x.
Final Answer
The force F required to lift the chain as a function of the height x above the platform is:
F(x)=ρxg+ρu2
We have a chain of length L and mass per unit length ρ. When we lift the chain vertically with a constant velocity u, we need to consider the forces acting on the chain.
When lifting the chain, the force F is applied upwards, while the weight of the chain acts downwards. The weight of the chain that has been lifted to a height x is given by:
Weight=mg=ρxg
where g is the acceleration due to gravity.
Since the chain is lifted with a constant velocity u, the rate of change of momentum must also be considered. The momentum p of the chain being lifted can be expressed as:
p=mu=ρu⋅x
The rate of change of momentum dpdt when lifting the chain can be calculated as:
dpdt=ddt(ρux)=ρudxdt=ρuu=ρu2
since dxdt=u.
According to Newton's second law, the net force Fnet acting on the chain is given by:
Fnet=F−Weight
Thus, we can write:
F−ρxg=dpdt
Substituting the expression for dpdt:
F−ρxg=ρu2
Rearranging the equation gives:
F=ρxg+ρu2
This expression shows the force F as a function of the height x.
The force F required to lift the chain as a function of the height x above the platform is:
F(x)=ρxg+ρu2
Topper's Solved these Questions
- LINEAR MOMENTUM & ITS CONSERVATIONBOOK - PHYSICS GALAXY - ASHISH ARORACHAPTER - LINEAR MOMENTUM & ITS CONSERVATIONEXERCISE - Numerical MCQ s
75 Videos
- LINEAR MOMENTUM & ITS CONSERVATIONBOOK - PHYSICS GALAXY - ASHISH ARORACHAPTER - LINEAR MOMENTUM & ITS CONSERVATIONEXERCISE - Advance McQ
20 Videos
- LINEAR MOMENTUM & ITS CONSERVATIONBOOK - PHYSICS GALAXY - ASHISH ARORACHAPTER - LINEAR MOMENTUM & ITS CONSERVATIONEXERCISE - Discussio Q
28 Videos
- KINEMATICSBOOK - PHYSICS GALAXY - ASHISH ARORACHAPTER - KINEMATICSEXERCISE - Unsolved Numerical Pro.
83 Videos
- NEWTONS LAWS OF MOTIONBOOK - PHYSICS GALAXY - ASHISH ARORACHAPTER - NEWTONS LAWS OF MOTIONEXERCISE - Unsolved Numerical Problem
81 Videos
Similar Questions
The centre of mass of a non-uniform rod of length L whose mass per unit length λ is proportional to x2, where x is distance from one end
View SolutionA chain of length l and mass m lies in a pile on the floor. If its end A is raised vertically at a constant speed v0, express in terms of the length y of chain which is off the floor at any given instant.
(a) The magnitude of the force P applied to end A.
(b) Energy lost during the lifting of the chain.

View Solution
Knowledge Check
A chain of length L and mass per unit length ρ is piled on a horizontal surface. One end of the chain is lifted vertically with constant velocity by a force P.
AP as function of height x of the end above the surface will be ρ(gx+v2)Bno energy will loss in this process.Cwork done by force will be 12ρgL2+ρv2LDloss in energy 12ρgLv2A string of length L and mass M is lying on a horizontal table. A force F is applied at one of its ends. Tension in the string at a distance x from the end at which force is applied is
AZeroBF should be equal to the weight of A and BCF(L-x)/LDF(L-x)MA string of length L and mass M is lying on a horizontal table. A force F is applied at one of its ends. Tension in the string at a distance x from the end at which force is applied is
AZeroBFCF(L−y)LDF(l−y)M
A uniform rope of length L and mass m is held at one end and whirled in a horizontal circle with angular velocity ω .
Ignore gravity. Find the time required for a transverse wave to travel from one end of the rope to the other.
View SolutionA thin rod AB of length a has variable mass per unit length ρ0(1+xa) where x is the distance measured from A and ρ0 is a constant.
Find the mass M of the rod.
View SolutionA steel rope has length L area of cross-section A young's modulus Y [density =d] (i) it is pulled on a horizotnal fritionless floor with a constant horizontal force F=|dALg|/2 applied at one end find the strain at the midpoint.
(ii). If the steel rope is vertical and moving with the force acting vertically up at the upper end find the strain at distance L/3 from lowerr end.
View SolutionFigure shows a rod of length l resting on a wall and the floor. Its lower end A is pulled towards left with a constant velocity u. As a result of this, end A starts moving down along the wall. Find the velocity of the other end B downward when the rod makes an angle θ with the horizontal.

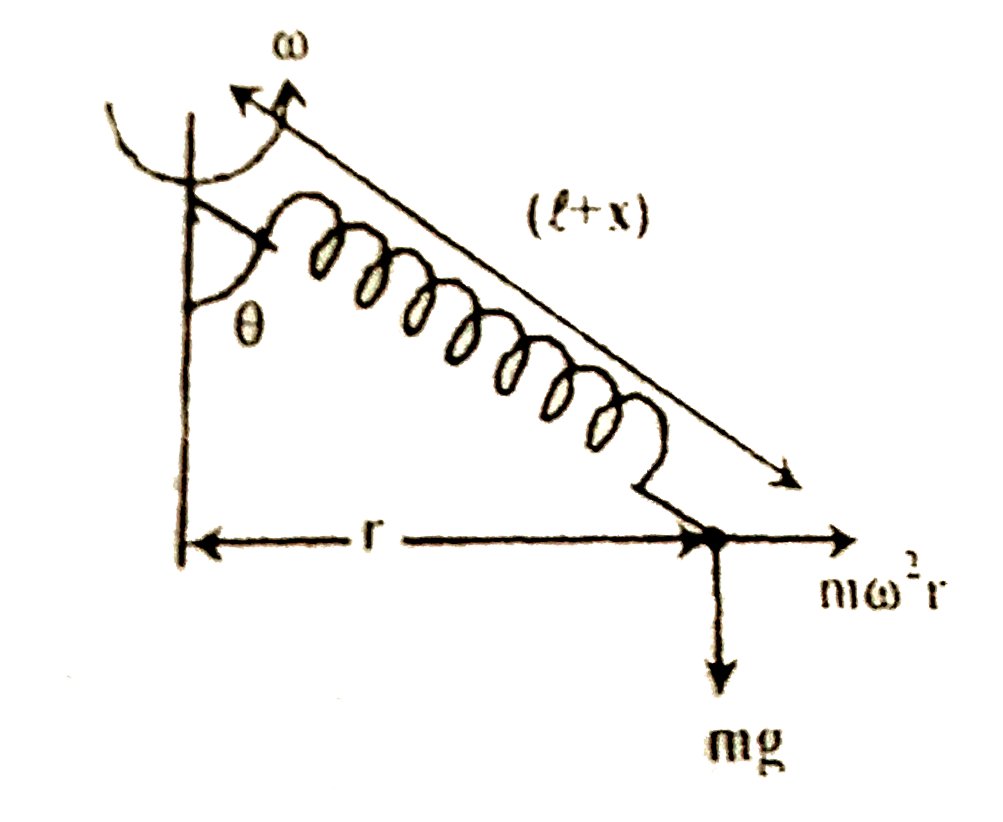
View SolutionA particle of mass m is fixed to one end of a light spring of force constant k and unstretched length l. The other end of the spring is fixed on a vertical axis which is rotaing with an angular velocity ω. The increase in length of th espring will be
View Solution
