A small sphere of mass m and radius r rolls without slipping on the insides of a large hemisphere of radius R whose axis of symmetry is vertical. Its starts at the top from rest. What is the kinetic energy of the small sphere at the bottom? What fraction is rotational and what transiational? What normal force does the small spheres exert on the hemisphere at the bottom?
[Hint: consider circular motion of center of mass at the bottom to calcualte normal reaction. For others consider conservation of energy.?
The correct Answer is:mg(R-r),2/7,5/7,17mg/7
To solve the problem, we will follow these steps:
Step 1: Determine the potential energy at the top
The small sphere starts from rest at the top of the hemisphere. The potential energy (PE) at the top is given by:
PE=mgh
where h=R−r is the height from which the sphere falls. Thus,
PE=mg(R−r)
Step 2: Apply conservation of energy
As the sphere rolls down without slipping, the potential energy at the top will convert into kinetic energy (KE) at the bottom. The total kinetic energy at the bottom consists of translational kinetic energy and rotational kinetic energy:
KE=KEtranslational+KErotational
KE=12mv2+12Iω2
Step 3: Substitute the moment of inertia and relate ω to v
For a solid sphere, the moment of inertia I is:
I=25mr2
Since the sphere rolls without slipping, we have:
ω=vr
Thus,
ω2=v2r2
Substituting this into the kinetic energy equation gives:
KE=12mv2+12(25mr2)(v2r2)
KE=12mv2+15mv2=710mv2
Step 4: Set potential energy equal to kinetic energy
Using conservation of energy:
mg(R−r)=710mv2
Cancelling m from both sides:
g(R−r)=710v2
Solving for v2:
v2=10g(R−r)7
Step 5: Calculate the total kinetic energy at the bottom
Now, substituting v2 back into the kinetic energy equation:
KE=710mv2=710m(10g(R−r)7)=mg(R−r)
Step 6: Determine the fraction of translational and rotational kinetic energy
1. Rotational Kinetic Energy:
KErotational=12Iω2=12(25mr2)(v2r2)=15mv2
Substituting v2:
KErotational=15m(10g(R−r)7)=2mg(R−r)7
2. Translational Kinetic Energy:
KEtranslational=12mv2=12m(10g(R−r)7)=5mg(R−r)7
Step 7: Calculate the normal force at the bottom
At the bottom, the forces acting on the sphere are the normal force N and the weight mg. The centripetal force required for circular motion is provided by the net force:
N−mg=mv2R−r
Substituting v2:
N−mg=m(10g(R−r)7)R−r
N−mg=10mg7
Thus,
N=mg+10mg7=17mg7
Summary of Results
- Kinetic Energy at the Bottom: KE=mg(R−r)
- Fraction of Rotational Kinetic Energy: 27
- Fraction of Translational Kinetic Energy: 57
- Normal Force at the Bottom: N=17mg7
The small sphere starts from rest at the top of the hemisphere. The potential energy (PE) at the top is given by:
PE=mgh
where h=R−r is the height from which the sphere falls. Thus,
PE=mg(R−r)
As the sphere rolls down without slipping, the potential energy at the top will convert into kinetic energy (KE) at the bottom. The total kinetic energy at the bottom consists of translational kinetic energy and rotational kinetic energy:
KE=KEtranslational+KErotational
KE=12mv2+12Iω2
For a solid sphere, the moment of inertia I is:
I=25mr2
Since the sphere rolls without slipping, we have:
ω=vr
Thus,
ω2=v2r2
Substituting this into the kinetic energy equation gives:
KE=12mv2+12(25mr2)(v2r2)
KE=12mv2+15mv2=710mv2
Using conservation of energy:
mg(R−r)=710mv2
Cancelling m from both sides:
g(R−r)=710v2
Solving for v2:
v2=10g(R−r)7
Now, substituting v2 back into the kinetic energy equation:
KE=710mv2=710m(10g(R−r)7)=mg(R−r)
1. Rotational Kinetic Energy:
KErotational=12Iω2=12(25mr2)(v2r2)=15mv2
Substituting v2:
KErotational=15m(10g(R−r)7)=2mg(R−r)7
KEtranslational=12mv2=12m(10g(R−r)7)=5mg(R−r)7
At the bottom, the forces acting on the sphere are the normal force N and the weight mg. The centripetal force required for circular motion is provided by the net force:
N−mg=mv2R−r
Substituting v2:
N−mg=m(10g(R−r)7)R−r
N−mg=10mg7
Thus,
N=mg+10mg7=17mg7
- Kinetic Energy at the Bottom: KE=mg(R−r)
- Fraction of Rotational Kinetic Energy: 27
- Fraction of Translational Kinetic Energy: 57
- Normal Force at the Bottom: N=17mg7
Topper's Solved these Questions
- ROTATIONAL DYNAMICS, MOMENT OF INERTIABOOK - NN GHOSHCHAPTER - ROTATIONAL DYNAMICS, MOMENT OF INERTIAEXERCISE - Exercises
42 Videos
- MOMENT, TORQUE, EQUILIBRIUM OF BODIESBOOK - NN GHOSHCHAPTER - MOMENT, TORQUE, EQUILIBRIUM OF BODIESEXERCISE - Exercises
33 Videos
- SATURATED AND UNSATURATED VAPOURBOOK - NN GHOSHCHAPTER - SATURATED AND UNSATURATED VAPOUREXERCISE - All Questions
1 Videos
Similar Questions
A small steel sphere of mass m and radius r rolls without slipping on the fiictionless surface of a large hemisphere of radius R{R gt gt r) whose axis of symmetry is vertical. It starts at the top from the rest, (a) What is the kinetic energy at the bottom ? (b) What fraction is the rotational kinetic energy of the total kinetic energy at the bottom? (b) What fraction is the rotational kinetic energy of the total kinetic energy? (c) What fraction is the translational kinetic energy of the total kinetic energy? (d) Calculate the normal force that the small sphere will exerton.the hemisphere at its bottom. Howthe results will be affected if r is not very sjnall as compared to R.
View Solution
A solid sphere of mass m and radius R is rolling without slipping as shown in figure. Find angular momentum of the sphere about z-axis.
View Solution
Knowledge Check
A small sphere S of radius ,r and mass m rolls without slipping, inside a large hemispherical bowl B of radius R as shown in figure. S starts from rest at the top point of the hemisphere
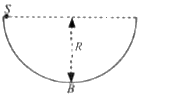 AThe fraction of translational kinetic energy at the sphere is 5/7.BThe fraction of rotational kinetic energy of the sphere is 2/7.CThe fraction of rotational kinetic energy of the sphere is 27mv2.DThe total kinetic energy of the sphere at the bottom of the bowl is 27mv2
AThe fraction of translational kinetic energy at the sphere is 5/7.BThe fraction of rotational kinetic energy of the sphere is 2/7.CThe fraction of rotational kinetic energy of the sphere is 27mv2.DThe total kinetic energy of the sphere at the bottom of the bowl is 27mv2A solid sphere of mass m and radius R rolls without slipping on a horizontal surface such that vc.m.=v0.
AThe kinetic energy of rotation is 15mv20.BThe total kinetic energy is 710mv20.CThe mechanical energy (assume the ground as reference) is mgR+710mv20.DAll options are correct.A solid sphere of mass 500 gm and radius 10 cm rolls without slipping with the velocity 20cm/s. The total kinetic energy of the sphere will be
A0.028 JB280 JC140 JD0.014 J
A solid sphere having mass m and radius r rolls down an inclined plane. Then its kinetic energy is
View SolutionA uniform ball of radius r rolls without slipping down from the top of a sphere of radius R Find the angular velocity of the ball at the moment it breaks off the sphere. The initial velocity of the ball is negligible.
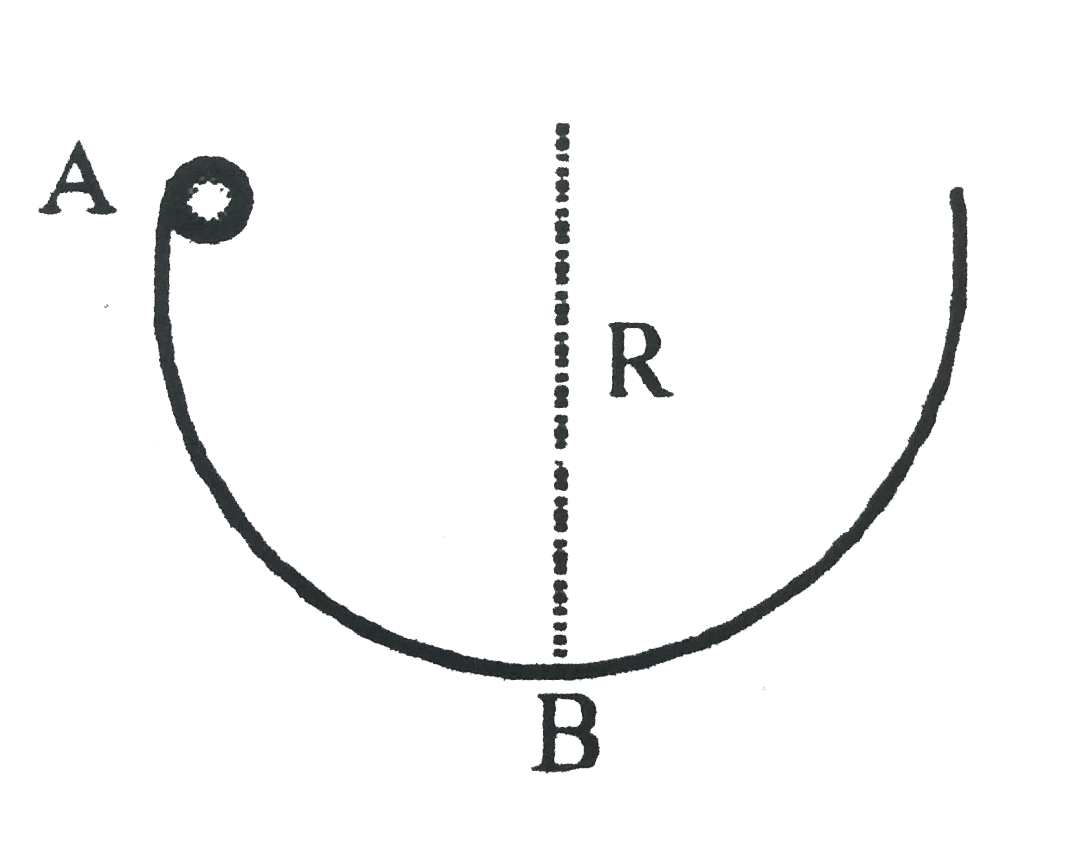
View SolutionA small sphere D of mass and radius rols without slipping inside a large fixed hemispherical radius R(>>r) as shown in figure. If the sphere starts from rest at the top point of the hemisphere normal force exerted by the small sphere on the hemisphere when its is at the bottom B of the hemisphere.
 .
.
View SolutionA small ball of radius r rolls without sliding in a big hemispherical bowl. of radius R. what would be the ratio of the translational and rotaional kinetic energies at the bottom of the bowl.
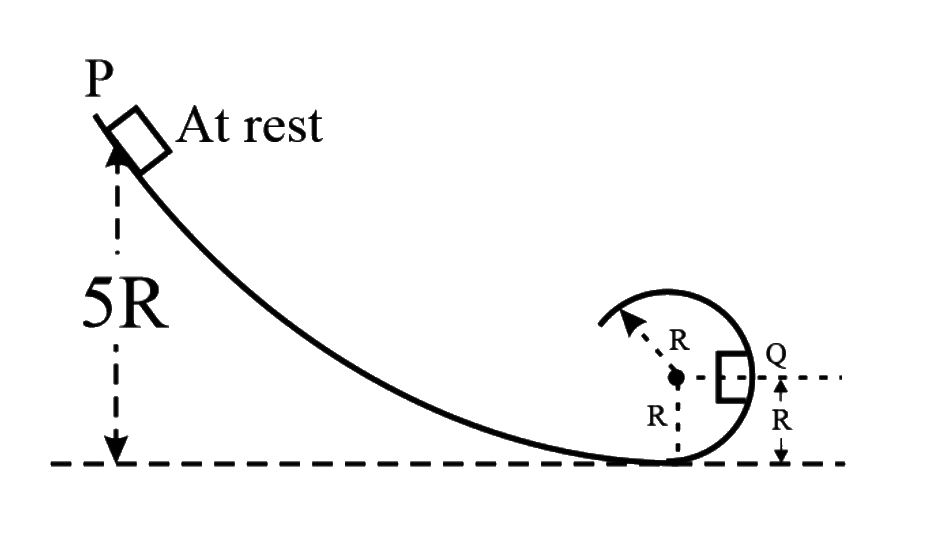
View SolutionA small block of mass m slides along a smooth frictional track as shown in the figure. If it starts from rest at P, what is the resultant force acting on it at Q?
View Solution
