A uniform circular plate of uniform thickness has a diameter of 56 cm. A circular portion of diameter 42 cm is removed from the edge of the plate. Find the position of the centre of mass of the remaining portion.
To find the position of the center of mass of the remaining portion of the circular plate after a smaller circular portion is removed, we can follow these steps:
Step 1: Determine the dimensions of the plates
- The diameter of the original circular plate is 56 cm, so its radius R1=562=28 cm.
- The diameter of the removed circular portion is 42 cm, so its radius R2=422=21 cm.
Step 2: Set the coordinate system
- Place the center of the original circular plate at the origin (0, 0).
- The center of the removed circular portion will be located at a distance of R1−R2=28−21=7 cm from the origin along the positive x-axis.
Step 3: Calculate the mass of the original plate
- The mass M1 of the original circular plate can be expressed in terms of its area and density:
M1=ρ⋅πR21=ρ⋅π(282)=ρ⋅π⋅784
Step 4: Calculate the mass of the removed portion
- The mass M2 of the removed circular portion is:
M2=ρ⋅πR22=ρ⋅π(212)=ρ⋅π⋅441
Step 5: Calculate the center of mass of the removed portion
- The center of mass of the removed portion is located at x=7 cm (since it is at the edge of the original plate).
Step 6: Apply the center of mass formula
- The new center of mass xcm of the remaining portion can be calculated using the formula:
xcm=M1⋅x1−M2⋅x2M1−M2
where x1=0 (center of the original plate) and x2=7 cm (center of the removed portion).
Step 7: Substitute the values into the formula
- Substitute M1, M2, x1, and x2:
xcm=(ρ⋅π⋅784)⋅0−(ρ⋅π⋅441)⋅7ρ⋅π⋅784−ρ⋅π⋅441
- Simplifying, we can cancel out ρ⋅π:
xcm=−441⋅7784−441
Step 8: Calculate the values
- Calculate the denominator:
784−441=343
- Calculate the numerator:
−441⋅7=−3087
- Thus, we have:
xcm=−3087343≈−9 cm
Step 9: Interpret the result
- The negative sign indicates that the center of mass of the remaining portion is located 9 cm to the left of the origin (the center of the original plate).
Final Answer
The position of the center of mass of the remaining portion is approximately −9 cm from the center of the original plate.
---
- The diameter of the original circular plate is 56 cm, so its radius R1=562=28 cm.
- The diameter of the removed circular portion is 42 cm, so its radius R2=422=21 cm.
- Place the center of the original circular plate at the origin (0, 0).
- The center of the removed circular portion will be located at a distance of R1−R2=28−21=7 cm from the origin along the positive x-axis.
- The mass M1 of the original circular plate can be expressed in terms of its area and density:
M1=ρ⋅πR21=ρ⋅π(282)=ρ⋅π⋅784
- The mass M2 of the removed circular portion is:
M2=ρ⋅πR22=ρ⋅π(212)=ρ⋅π⋅441
- The center of mass of the removed portion is located at x=7 cm (since it is at the edge of the original plate).
- The new center of mass xcm of the remaining portion can be calculated using the formula:
xcm=M1⋅x1−M2⋅x2M1−M2
where x1=0 (center of the original plate) and x2=7 cm (center of the removed portion).
- Substitute M1, M2, x1, and x2:
xcm=(ρ⋅π⋅784)⋅0−(ρ⋅π⋅441)⋅7ρ⋅π⋅784−ρ⋅π⋅441
- Simplifying, we can cancel out ρ⋅π:
xcm=−441⋅7784−441
- Calculate the denominator:
784−441=343
- Calculate the numerator:
−441⋅7=−3087
- Thus, we have:
xcm=−3087343≈−9 cm
- The negative sign indicates that the center of mass of the remaining portion is located 9 cm to the left of the origin (the center of the original plate).
The position of the center of mass of the remaining portion is approximately −9 cm from the center of the original plate.
Topper's Solved these Questions
- MOMENT, TORQUE, EQUILIBRIUM OF BODIESBOOK - NN GHOSHCHAPTER - MOMENT, TORQUE, EQUILIBRIUM OF BODIESEXERCISE - Exercises
33 Videos
- LAWS OF MOTION, FREE BODY DIAGRAMBOOK - NN GHOSHCHAPTER - LAWS OF MOTION, FREE BODY DIAGRAMEXERCISE - Exercise (B)
34 Videos
- ROTATIONAL DYNAMICS, MOMENT OF INERTIABOOK - NN GHOSHCHAPTER - ROTATIONAL DYNAMICS, MOMENT OF INERTIAEXERCISE - Exercises
42 Videos
Similar Questions
A circular plate of unifrom thickness has a diameter of 56cm. A circular portion of diameter 42cm is removed from the edge of the plate as shown in Fig. Find the position of centre of mass of the remaining portion.
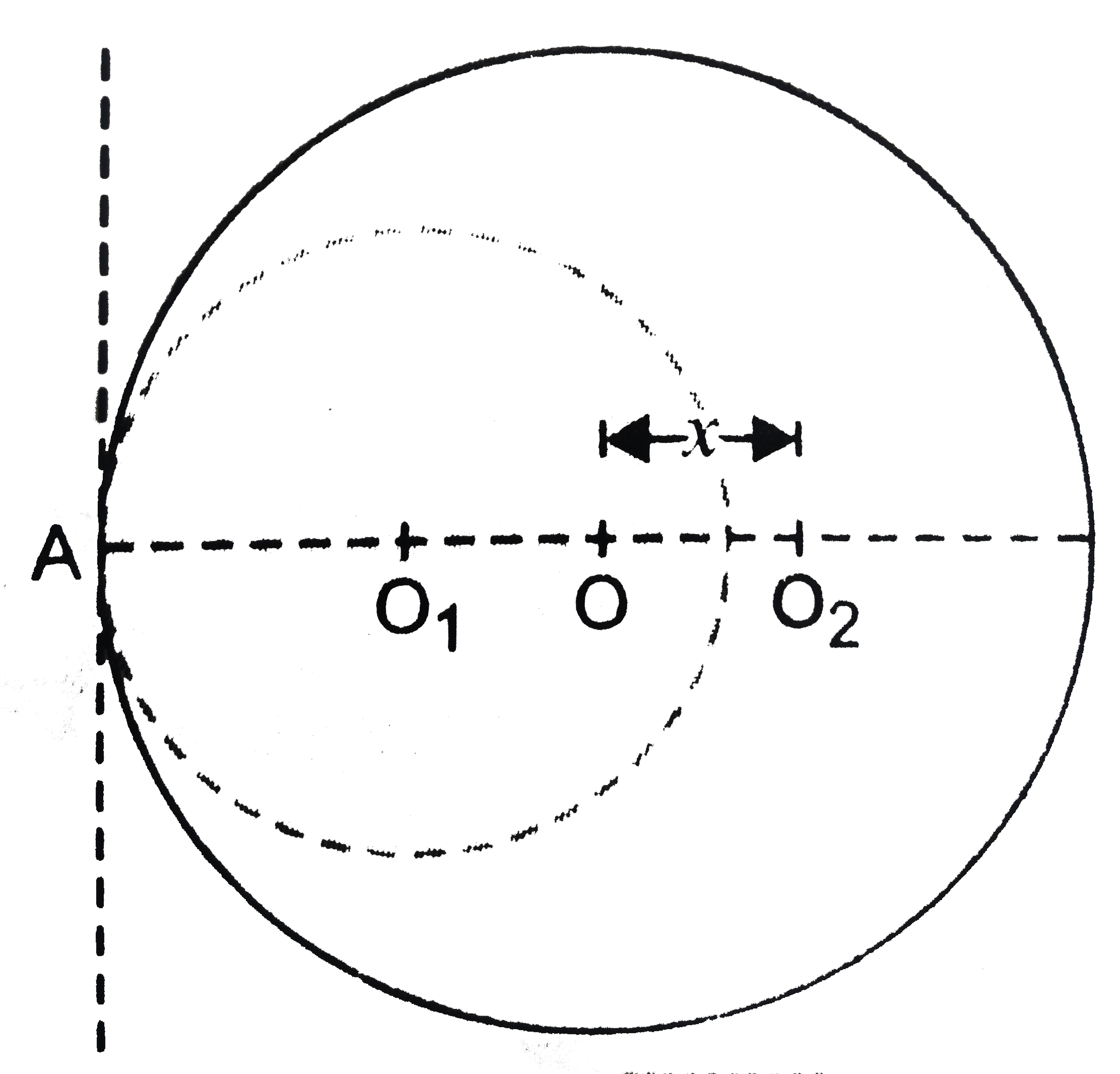
View SolutionA circular plate of unifrom thickness has a diameter of 56cm. A circular portion of diameter 42cm is removed from the edge of the plate as shown in Fig. Find the position of centre of mass of the remaining portion.

View Solution
Knowledge Check
A circular plate of uniform thickness has a diameter of 28cm. A circular portion of diameter 21cm is removed from the plate as shown. Ois the centre of mass of complete plate. The position of centre of mass of remaining portion will shift towards left from 'O' by
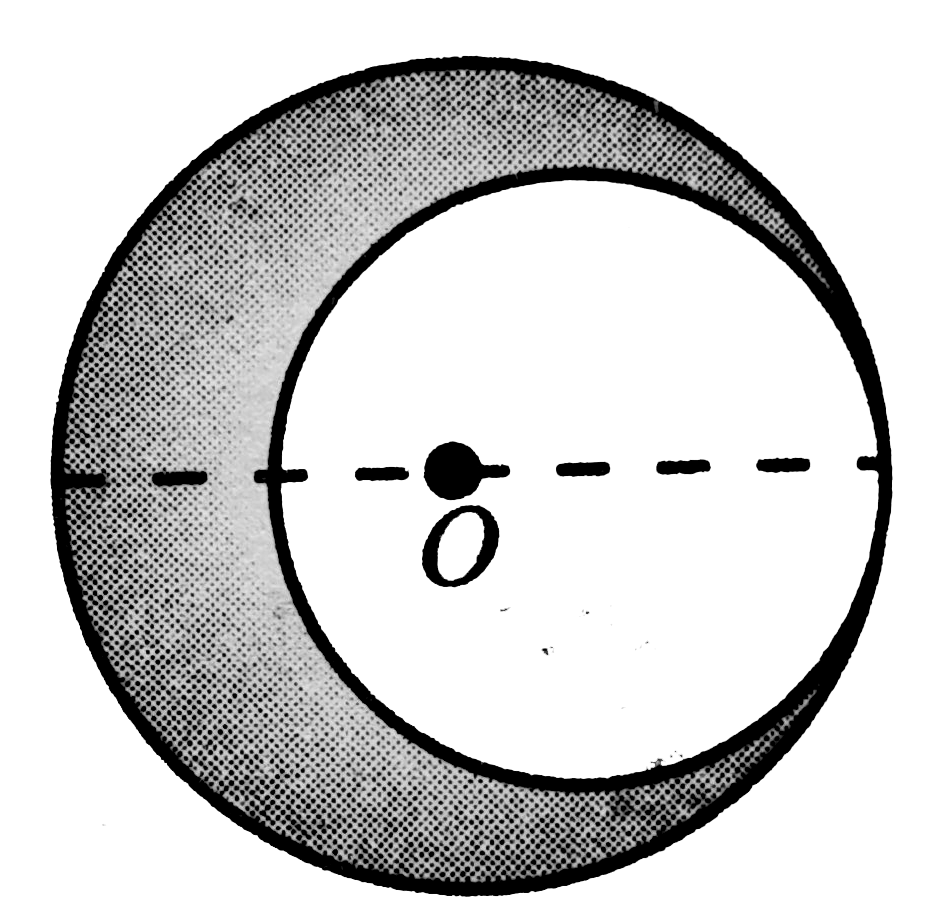 A5cmB9cmC4.5cmD5.5cm
A5cmB9cmC4.5cmD5.5cmA square plate of side 20cm has uniform thickness and density. A circular part of diameter 8cm is cut out symmetrically and shown in figure. The position of center of mass of the remaining potion is
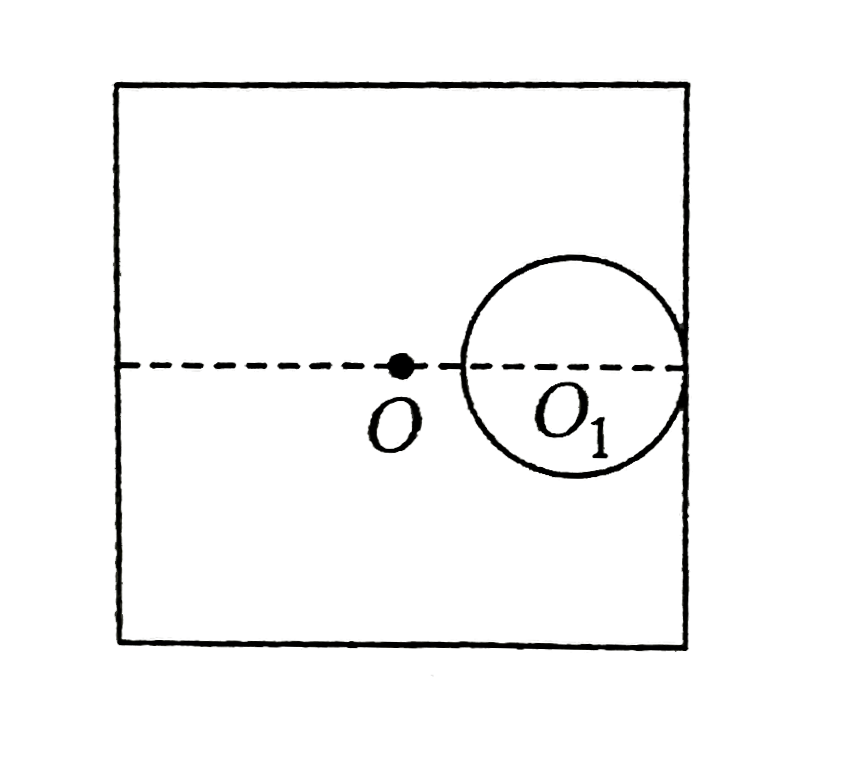 Aat O1Bat OC0.54 cm from O on the left hand sideDNone of the above
Aat O1Bat OC0.54 cm from O on the left hand sideDNone of the aboveA uniform sphere has radius R. A sphere of diameter R is cut from its edge as shown. Then the distance of centre of mass of remaining portion from the centre of mass of the original sphere is
 AR/7BR/14C2R/7DR/18
AR/7BR/14C2R/7DR/18
Fron a sqaure plate of side a,a quarter circular disc of radius a is removed as shown in figure. Find the coordinates of centre of mass of the remaining part.
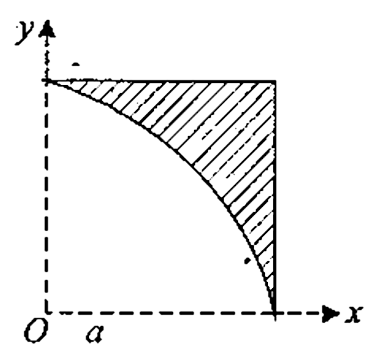
View SolutionFrom a uniform circular plate of radius R, a small circular plate of radius R/4 is cut off as shown. If O is the center of the complete plate, then the x coordinate of the new center of mass of the remaining plate will be: X o Y
View SolutionFrom a uniform circular disc of diameter d,a circular disc of diameter d/6 and having centre at a distance d/4 from the centre of the disc is scooped out. Determine the centre of mass of remaining portion.
View SolutionA circular wire of diameter 42 cm is bent in the form of a rectangle whose sides are in the ratio 6 : 5. Find the area of the rectangle.
View SolutionFrom a uniform circular disc of diameter D, a circular disc or hole of diameter D/6 and having its centre at a distance of D/4 from the centre of the disc is scopped out. Determine the centre of mass of the remaining portion.
View Solution