Miriam and Jessica are growing bacteria in a laboratory. Miriam uses the growth function f(t)=n2t to model her experiment while Jessica uses the function g(t)=(n2)4t to model her experiment. In each case, n represents the initial number of bacteria, and t is the time, in hours. If Miriam starts with 6 bacteria, how many bacteria should Jessica start with to achieve the same growth over time?
32
16
8
4
The correct Answer is:C
To solve the problem, we need to find out how many bacteria Jessica should start with in order to achieve the same growth over time as Miriam.
1. Identify Miriam's Growth Function:
Miriam's growth function is given by:
f(t)=n2t
where n is the initial number of bacteria. In this case, Miriam starts with 6 bacteria, so:
f(t)=62t
2. Rewrite Miriam's Growth Function:
We can express 62t in a different form:
62t=(62)t=36t
3. Identify Jessica's Growth Function:
Jessica's growth function is given by:
g(t)=(n2)4t
4. Set the Growth Functions Equal:
To find the initial number of bacteria n that Jessica should start with to achieve the same growth as Miriam, we set the two growth functions equal at some time t:
36t=(n2)4t
5. Simplify the Equation:
We can simplify the right side:
(n2)4t=(n424)t=n416t
Thus, we have:
36t=n416t
6. Equate the Bases:
Since the bases are equal, we can equate the terms inside the exponent:
36=n416
7. Solve for n:
Multiply both sides by 16:
36×16=n4
Calculate 36×16:
576=n4
Now take the fourth root of both sides:
n=√(4)576
We can simplify 576 as 242:
n=√(4)242=241/2=4.89898≈8
8. Conclusion:
Therefore, Jessica should start with approximately 8 bacteria to achieve the same growth over time as Miriam.
Final Answer:
Jessica should start with 8 bacteria.
Miriam's growth function is given by:
f(t)=n2t
where n is the initial number of bacteria. In this case, Miriam starts with 6 bacteria, so:
f(t)=62t
We can express 62t in a different form:
62t=(62)t=36t
Jessica's growth function is given by:
g(t)=(n2)4t
To find the initial number of bacteria n that Jessica should start with to achieve the same growth as Miriam, we set the two growth functions equal at some time t:
36t=(n2)4t
We can simplify the right side:
(n2)4t=(n424)t=n416t
Thus, we have:
36t=n416t
Since the bases are equal, we can equate the terms inside the exponent:
36=n416
Multiply both sides by 16:
36×16=n4
Calculate 36×16:
576=n4
Now take the fourth root of both sides:
n=√(4)576
We can simplify 576 as 242:
n=√(4)242=241/2=4.89898≈8
Therefore, Jessica should start with approximately 8 bacteria to achieve the same growth over time as Miriam.
Jessica should start with 8 bacteria.
Topper's Solved these Questions
- PROBLEM SOLVING AND DATA ANALYSISBook:ENGLISH SATChapter:PROBLEM SOLVING AND DATA ANALYSISExercise:Grib-In
Explore 40 Videos
- PROBLEM SOLVING AND DATA ANALYSISBook:ENGLISH SATChapter:PROBLEM SOLVING AND DATA ANALYSISExercise:Grib-In
Explore 40 Videos
- PROBLEM SOLVING AND DATA ANALYSISBook:ENGLISH SATChapter:PROBLEM SOLVING AND DATA ANALYSISExercise:EXERICSE
Explore 80 Videos
- PROBLEMS IN CONTEXT Book:ENGLISH SATChapter:PROBLEMS IN CONTEXT Exercise:PRACTICE TEST
Explore 10 Videos
Similar Questions
n=12×2t3
The number of mice in a certain colony is shown by the formula above, such that n is the number of mice and t is the time, in months, since the start of the colony. IF 2 years have passed since the start of the colony, how many mice does the colony contain now?
View SolutionGiven a starting population of 100 bacteria, the formula b(t)=100(2t) can be used to determine the number of bacteria, b, after t periods of time. If each time period is 15 minutes long, how many minutes will it take for the population of bacteria to reach 51,200?
View SolutionA scientist studies Bacteria Culture A, which grows then percent every hour. Bacteria Culture A initially contained 200 microbes, and she models the growth using the equation n=200(m)h, where n is the number of microbes and h is the number of hours.
Q. The same scientist also studies another culture, Bacteria Culture B, which grows 15% every hour. The two cultures began at the same time with the same number of microbes. After 20 hours, how many more microbes will becteria Culture B contain than Bacteria Culture A ? (Round your answer to the nearest whole number).
View SolutionA scientist studies Bacteria Culture A, which grows then percent every hour. Bacteria Culture A initially contained 200 microbes, and she models the growth using the equation n=200(m)h, where n is the number of microbes and h is the number of hours.
Q. What is the value of m in the equation ?
View SolutionA biologist places a colony consisting of 5000 bacteria into a petri dish. After the intial placement of the bacteria at time t = 0, the biologist measures and estimates the number of bacteria present every half hour. This data was then fitted by an exponential curve of the form y=c.2kt where c and k are constants, t is measured in hours, and y is measured in thousands of bacteria. The scatterplot together with the exponential curve are shown below.
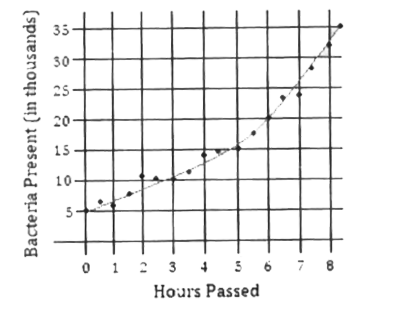
Suppose that the data was fitted with a quadratic function of the form t2+bt+c instead of an exponential function. Assume that the quadratic funtion agrees with the scatterplot at times t = 0 and t = 6. What is the t - coordinate of the vertex of the graph of the quadratic function?
View SolutionA biologist places a colony consisting of 5000 bacteria into a petri dish. After the intial placement of the bacteria at time t = 0, the biologist measures and estimates the number of bacteria present every half hour. This data was then fitted by an exponential curve of the form y=c.2kt where c and k are constants, t is measured in hours, and y is measured in thousands of bacteria. The scatterplot together with the exponential curve are shown below.
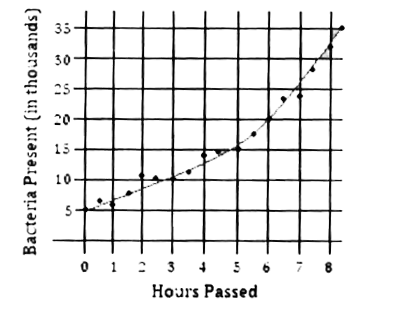
According to the scatterplot, the biologist'smeasurements indicate that the number of bacteria present quadrupled in 6 hours, and the exponential curve passes through the corresponding data point at time t = 6. The exponential function also agrees with the initial number of bacteria. Compute ck.
View SolutionA student performs an experiment for determination of g[=4π2LT2],L≈1m , and he commits an error of ΔL. For T he takes the time of n oscillations with the stop watch of least count ΔT . For which of the following data , the measurement of g will be most accurate ?
View SolutionIf in Bohr's model, for unielectronic atom, time period of revolution is represented as Tn,z where n represents shell no. and Z represents atomic number then the value of T1,2:T2,1 , will be :
View SolutionA pulse is started at a time t=0 along the +xdirection on a long, taut string. The shape of the pulse at t=0 is given by function f(x) with
here f and x are in centimeters. The linear mass density of the string is 50g/m and it is under a tension of 5N.
The transverse velocity of the particle at x=13cm and t=0.015s will be
View SolutionA 2kg mass is attached to a spring of force constant 600N/m and rests on a smooth horizontal surface. A second mass of 1kg slides along the surface toward the first at 6m/s.
(a) Find the amplitude of oscillation if the masses make a perfectly inelastic collision and remain together on the spring. what is the period of oscillation ?
(b) Find the amplitude and period of oscillation if the collision is perfectly elastic.
( c) For each case , write down the position x as a function of time t for the mass attached to the spring, assuming that the collision occurs at time t=0. What is the impulse given to the 2kg mass in each case ?
View Solution
