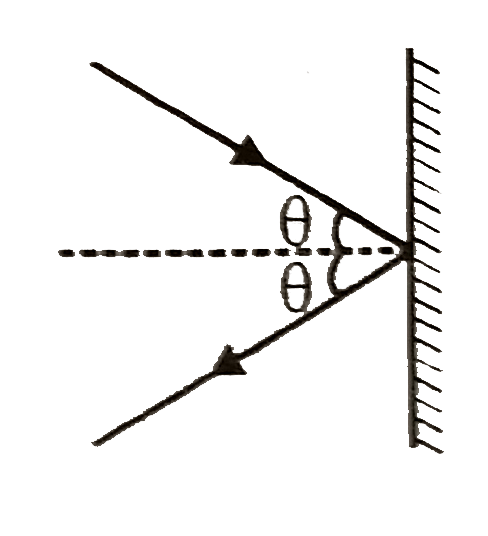
A stream of particles each of mass m moving with velocity v with number of particles per unit volume n strike against a wall at an angle θ to the normal elastically. Find the pressure exerted by the exerted by the stream on the wall.
The correct Answer is:2mvnv2cos2θ
Those partcles whose distance from the wall is less than v dt will striking the wall in time dt. Assuming the wall area to be A, volume of the part will be A (vdt) cosθ
∴ No. of molecules (dn)=n(dv)=n(Av dt)cosθ
change in momentum of each particle dp1=2mvcosθ
∴F=dpdt=(dn)(dp1)dt=[n(A vdtcosθ)]2mvcosθdt=2A n mv2cos2θ
⇒pressure=FA=2mnv2cos2θ
Topper's Solved these Questions
- CENTRE OF MASS & MOMENTUM CONSERVATIONBOOK - BANSALCHAPTER - CENTRE OF MASS & MOMENTUM CONSERVATIONEXERCISE - EXERCISE-4 (SECTION-A) (JEE-ADVANCED Previous Year Questions)
11 Videos
- CENTRE OF MASS & MOMENTUM CONSERVATIONBOOK - BANSALCHAPTER - CENTRE OF MASS & MOMENTUM CONSERVATIONEXERCISE - EXERCISE-4 (SECTION-A) (Comprehension)
3 Videos
- CENTRE OF MASS & MOMENTUM CONSERVATIONBOOK - BANSALCHAPTER - CENTRE OF MASS & MOMENTUM CONSERVATIONEXERCISE - EXERCISE-2[MATRIX TYPE]
2 Videos
- GRAVITATIONBOOK - BANSALCHAPTER - GRAVITATIONEXERCISE - EXERCISE -4 Section - B
6 Videos
Similar Questions
A parallel beam of molecules moving with velocity v impinges on a wall at an angle θ to its normal. Find the pressure exerted by the beam on the wall assuming perfect elastic collisions. The concentration of the molecules in the beam is n.
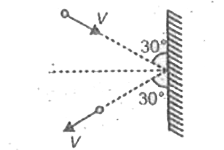
View SolutionA particle of mass m strikes a wall with speed v at an angle 30∘ with the wall elastically as shown in the figure. The magnitude of impulse imparted to the ball by the wall is
View Solution
Knowledge Check
A parallel beam of particles of mass m moving with velocity v impinges on a wall at an angle θ to its normal. The number of particles per unit volume in the beam is n. If the collision of particles with the wall is elastic, then the pressure exerted by this beam on the wall is
A2mnv2cosθB2mnv2cos2θC2mnvcosθD2mnvcos2θA molecule of mass m moving at a velocity v impinges elastically on the wall at an angle a with the wall. Then
Athe impulsive reaction of the wall is 2mvcosαBthe impulsive reaction of the wall is 2mvsinαCthe impulsive reaction of the wall is nonzeroDgiven data is insufficient to calculate impulsive reaction of the wallA particle of mass 2m moving with velocity v strikes a stationary particle of mass 3m and sticks to it . The speed of the system will be
A0.8vB0.2vC0.6vD0.4v
A parallel beam of nitrogen molecules moving with velocity v=400ms−1 imprings on a wall at an angle θ=30∘ to its normal. The concentration of molecules in the beam is n=9×1018cm−3. Find the pressure exerted by the beam on the wall, assuming that collisions are perfectly elastic.
View SolutionA particle of mass 2m moving with velocity v strikes a stationary particle of mass 3m and sticks to it. The speed of the system will be
View SolutionA particle of mass m1 moves with velocity v1 and collides with another particle at rest of equal mass. The velocity of the second particle after the elastic collision is
View SolutionA particle of mass m moving with velocity v strikes a stationary particle of mass 2m and sticks to it. The speed of the system will be.
View Solutionn bullets per sec each of mass m moving with velocity v strike normally on a wall. The collision is elastic then force on wall is-
View Solution