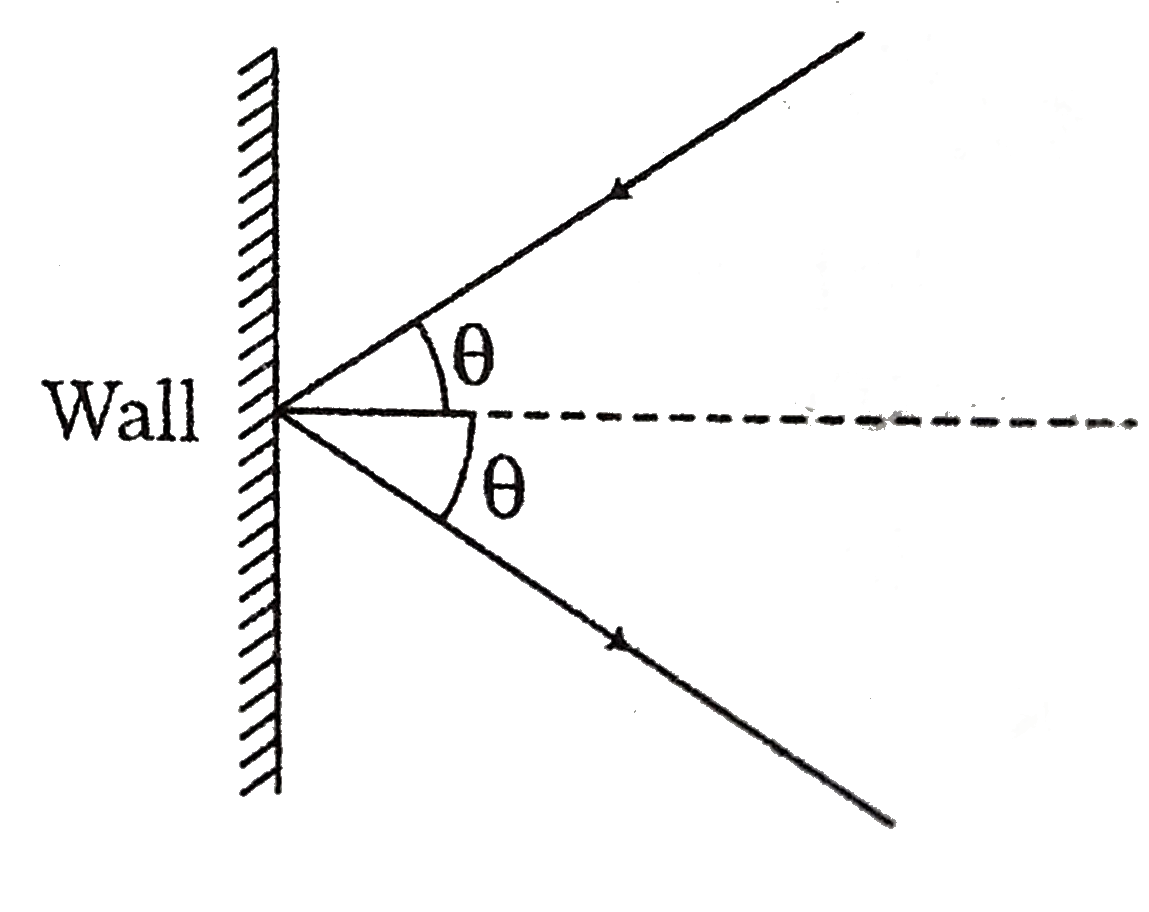
A parallel beam of nitrogen molecules moving with velocity $v = 400 m s^{- 1}$ imprings on a wall at an angle $θ = 30^{\circ}$ to its normal. The concentration of molecules in the beam is $n = 9 \times 10^{18} c m^{- 3}$ . Find the pressure exerted by the beam on the wall, assuming that collisions are perfectly elastic.

Video Solution

Text Solution

Verified by Experts

All the molecules contained in the cylinder of length v and inclined on the wall at an angle $θ$ to the normal will strike the wall every second. Let $Δ$ S be the base area of the cylinder.
The volume of the cylinder $= Δ S v cos θ$ .
Therefore, the number of molecules striking $Δ S$ every second $= (Δ S v cos θ \times n)$ .
Change of momentum per molecule $= 2 m v cos θ$ .
$∴ P r e s s u r e = \frac{F or c e}{A r e a} = \frac{(2 m v cos θ) (Δ S v cos θ \times n)}{(Δ S {cos}^{2} θ)} = 2 m v^{2} n$
$∴ p = 2 \times (\frac{28}{6.0 \times 10^{26}}) (9 \times 10^{24}) \times 400^{2} \times {cos}^{2} 30^{\circ}$
$= 1.00 \times 10^{5} N m^{- 2} = 1$ atm

Updated on:21/07/2023

A parallel beam of particles of mass $m$ moving with velocity $v$ impinges on a wall at an angle $θ$ to its normal. The number of particles per unit volume in the beam is $n$ . If the collision of particles with the wall is elastic, then the pressure exerted by this beam on the wall is
A $2 m n v^{2} cos θ$
B $2 m n v^{2} {cos}^{2} θ$
C $2 m n v cos θ$
D $2 m n v {cos}^{2} θ$
A molecule of mass m moving with a velocity v makes 5 elastic collisions with a wall of the container per second. The change in its momentum per second will be
Amv
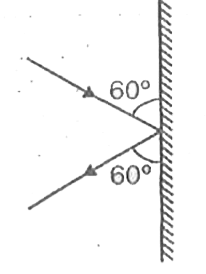
B5 mv
C $\frac{m v}{10}$
D10 mv
$10^{23}$ molecules of a gas, each having a mass of $3 \times 10^{- 27}$ kg strike per second per sq. cm. of a rigid will at an angle of $60^{\circ}$ with the normal and rebound with a velocity of $500 m / s$ . What is the pressure exerted by the gas molecules on the wall?
A $500 N / m^{2}$
B $1000 N / m^{2}$
C $1500 N / m^{2}$
D $2000 N / m^{2}$