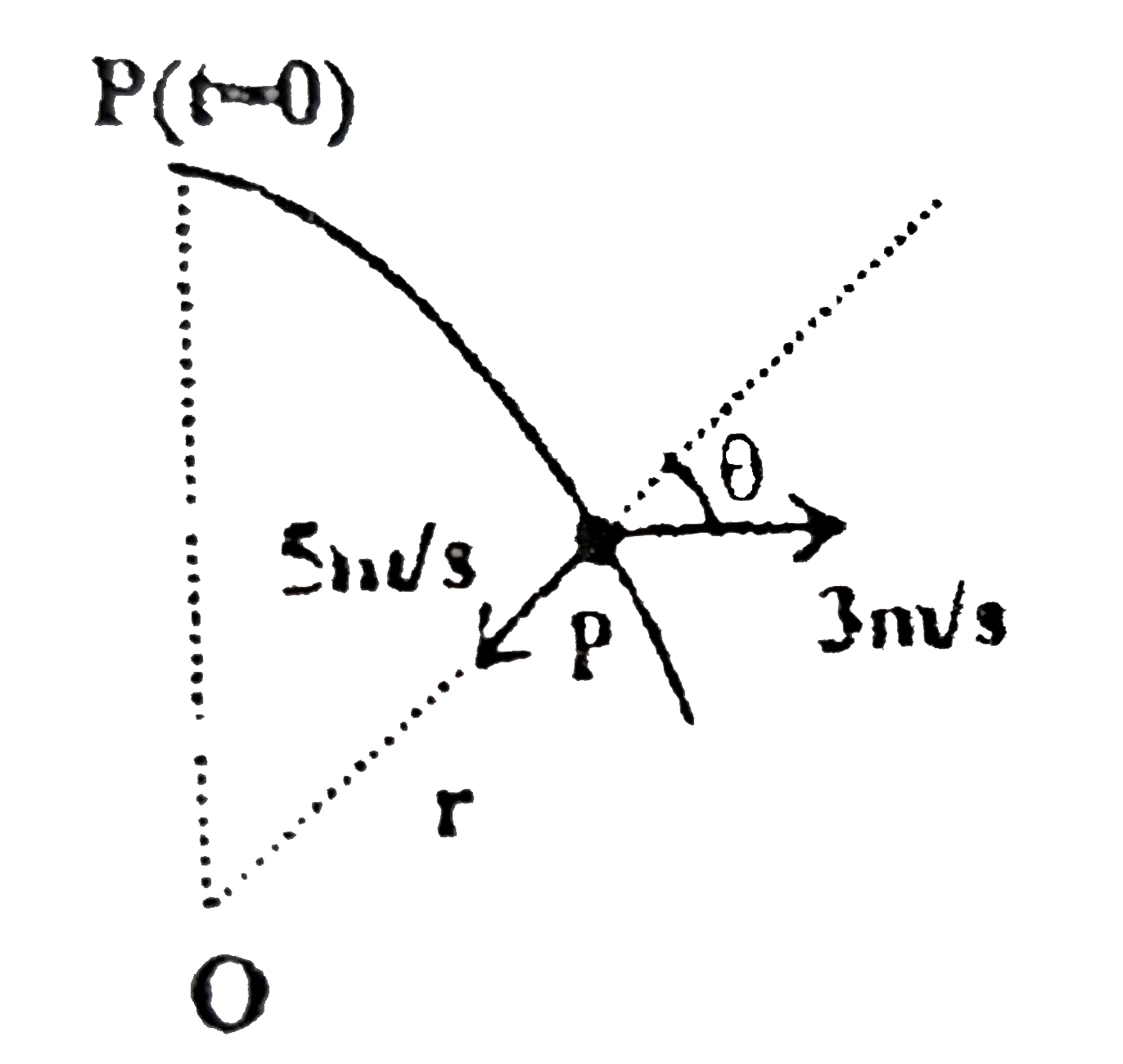
A particle P is initially at a distance c'=16m from a fixed point O. T

Two particles are initially located at points A and B distant d apart. They start moving at time t=0 such that the velocity $\to u$ of B is always along the horizontal and velocity $\to v$ of A is contiunously aimed at B. At $t = 0, \to u$ is perpendicu lar to $\to v$ The particles will meet after time:
A $\frac{v d}{v^{2} - u^{2}}$
B $\frac{v^{2} + u^{2}}{u d}$
C $\frac{v^{2} - u^{2}}{v d}$
D $\frac{u d}{(v^{2} - u^{2})}$
A particle $P$ is moving with a constant speed of $6 m / s$ in a direction $2 ˆ i - ˆ j + 2 ˆ k$ . When $t = 0, P$ is at a point whose position vectors is $3 ˆ i + 4 ˆ j - 7 ˆ k$ . Find the position vector of the particle $P$ after $4$ seconds.
A $18 ˆ i - 4 ˆ j - 23 ˆ k$
B $19 ˆ i - 4 ˆ j - 23 ˆ k$
C $19 ˆ i + 4 ˆ j - 23 ˆ k$
D $19 ˆ i - 4 ˆ j + 23 ˆ k$
A particle is projected with a velocity $\to v = 8 ˆ i + 6 ˆ j m / s$ . The time after which it will starts moving perpendicular to its initial direction of motions is
A $0.5 s$
B $1.25 s$
C $1 s$
D $5 / 3 s$

$- \frac{d r}{d t} = 5 - 3 cos θ$
$\int_{16}^{0} - d r = \int_{0}^{t} (5 - 3 cos θ) d t$
$16 = 5 t - 3 \int_{0}^{t} cos θ d t$
$\int_{0}^{t} (3 - 5 cos θ) d t = 0 \Rightarrow \int_{0}^{t} cos θ d t = \frac{3 t}{5}$
$16 = 5 t - \frac{9 t}{5}$
$t = 5 sec$ .