A capacitor initially given a charge Q0 is connected across a resistor R at t=0. The separation between the plates changes according to d=d0(1+t)(0≤t<1). A small bulb is connected across the plates of the capacitor which lights when potential difference across the plates of the capacitor reaches V0. Find
(a) the variation of charge with time
(b) time when the bulb will light.
Capacitance at t=0, C0=ε0Ad0, C=C0(1+t)
Using Kirchoff's law
qC−Ri=0
(i) qC0(1+t)+Rdqdt=0
dqq=−1RC0dt(1+t)
lnq∣∣qQ0=−1RC0ln(1+t)∣∣∣t0
ln(q/Q0)=−1RC0ln(1+t)
ln(q/Q0)=ln(1+t)1RC0
q=Q0(1+t)1RC0
V=qC=Q0(1+t)−1RCC0(1+t)=Q0C0(1+t)−(1RC+1)
which gives t=⎧⎨⎩(Q0C0V)(RCRC+1)−1⎫⎬⎭
Topper's Solved these Questions
- GMP ASSESMENTBOOK - FIITJEECHAPTER - GMP ASSESMENTEXERCISE - Objective problems
215 Videos
- GMP ASSESMENTBOOK - FIITJEECHAPTER - GMP ASSESMENTEXERCISE - Assertion & Reason
15 Videos
- GENERAL PHYSICSBOOK - FIITJEECHAPTER - GENERAL PHYSICSEXERCISE - MATRIX MATCH TYPE
3 Videos
- GRAVITATIONBOOK - FIITJEECHAPTER - GRAVITATIONEXERCISE - Numerical based Question
2 Videos
Similar Questions
A capacitor initially given a charge Qo is connected across a resistor R at t = 0. The separation between the plates changes according to d=do1+t(o≤t<1)A small bulb is connected across the plates of the capacitor which lights when potential difference across the plates of the capacitor reaches V_(o). Find
(a) the variation of charge with time
(b) till when the bulb will light
View SolutionIf A is connerted with D and B is connected with C find the potential difference a across each capacitor and the final charge in each capacitor.
View Solution
Knowledge Check
A capacitor is charged to a potential difference of 100 V and is then connected across a resistor. The potential difference across the capacitor decays exponentially with respect to time. After 1 sec, the P.D. between the plates of the capacitor is 80 V. what will be the potential difference between the plates after 2 sec ?
A32 VB48 VC64 VD70 VA capacitor is connected to a variable source of potential. Current flowing in the circuit and potential across the plates of capacitor at time t are shown in figure.

Capacity of the capacitor isA1 FB2 FC3 FD4 F
When a parallel plate capacitor is connected across a d.c. battery. Explain briefly how the capacitor gets charged .
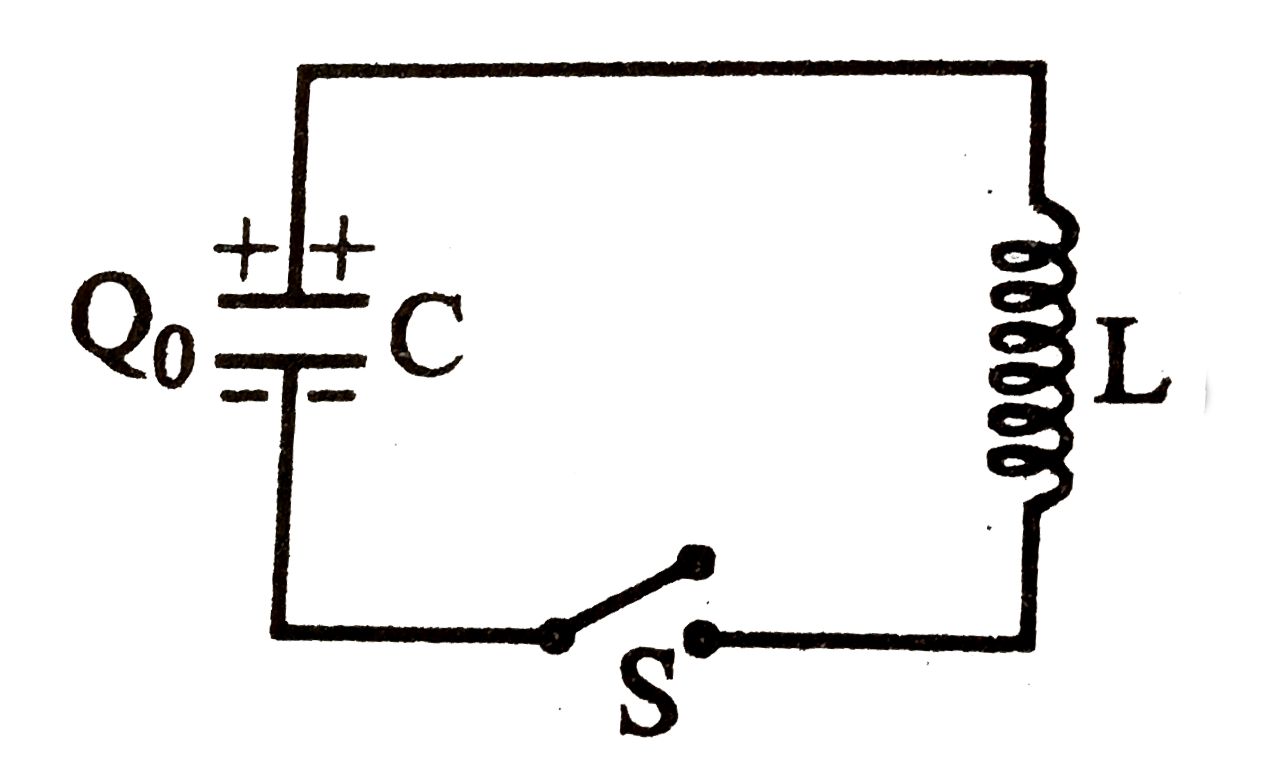
View SolutionA capacitor C with a charge Q0 is connected across an inductor through a switch S. If at t=0, the switch is closed, then find the instantaneous charge q on the upper plate of capacitor.
View SolutionA capacitor with an initial potential difference of 80.0 V is discharged through a resistor when a switch between them is closed at t=0. At t= 10.0s, the potential difference across the capacitor is 1.00V (a) What is time constant of the circuit? (b) What is the potential difference across the capacilor
View SolutionA capacitor is charged to a potential difference of 100V and then it is connected across a resistor.After one second,the potential drop across the plates of the capacitor becomes 80V .Then after 2sec the potential difference
View SolutionThe plates of capacitor are charged to a potential difference of 100V and then connected across a resistor.When the potential difference between the plates of the capacitor is 60V ,what is the fraction of the stored energy which has been dissipated?
View SolutionThe plates of parallel capaitor are given charges +4Q and −2Q. The capacitor is then connected across an uncharged capacitor of same capacitances first one (=C). Find the final potential difference between the plates of the first capacitor.
View Solution
