Two identical weightless rods are hinged to each other and to a horizontal beam (Fig.). The rigidity of each rod is k0, and the angle between them is 2α. Determine the rigidity k of the system of rods relative to the vertical displacement of a hinge A under the action of a certain force F, assuming that displacements are small in comparison with the length of the rods.

Step by step video & image solution for Two identical weightless rods are hinged to each other and to a horizontal beam (Fig.). The rigidity of each rod is k_(0), and the angle between them is 2alpha. Determine the rigidity k of the system of rods relative to the vertical displacement of a hinge A under the action of a certain force F, assuming that displacements are small in comparison with the length of the rods. by Physics experts to help you in doubts & scoring excellent marks in Class 12 exams.
Updated on:21/07/2023Topper's Solved these Questions
Similar Questions
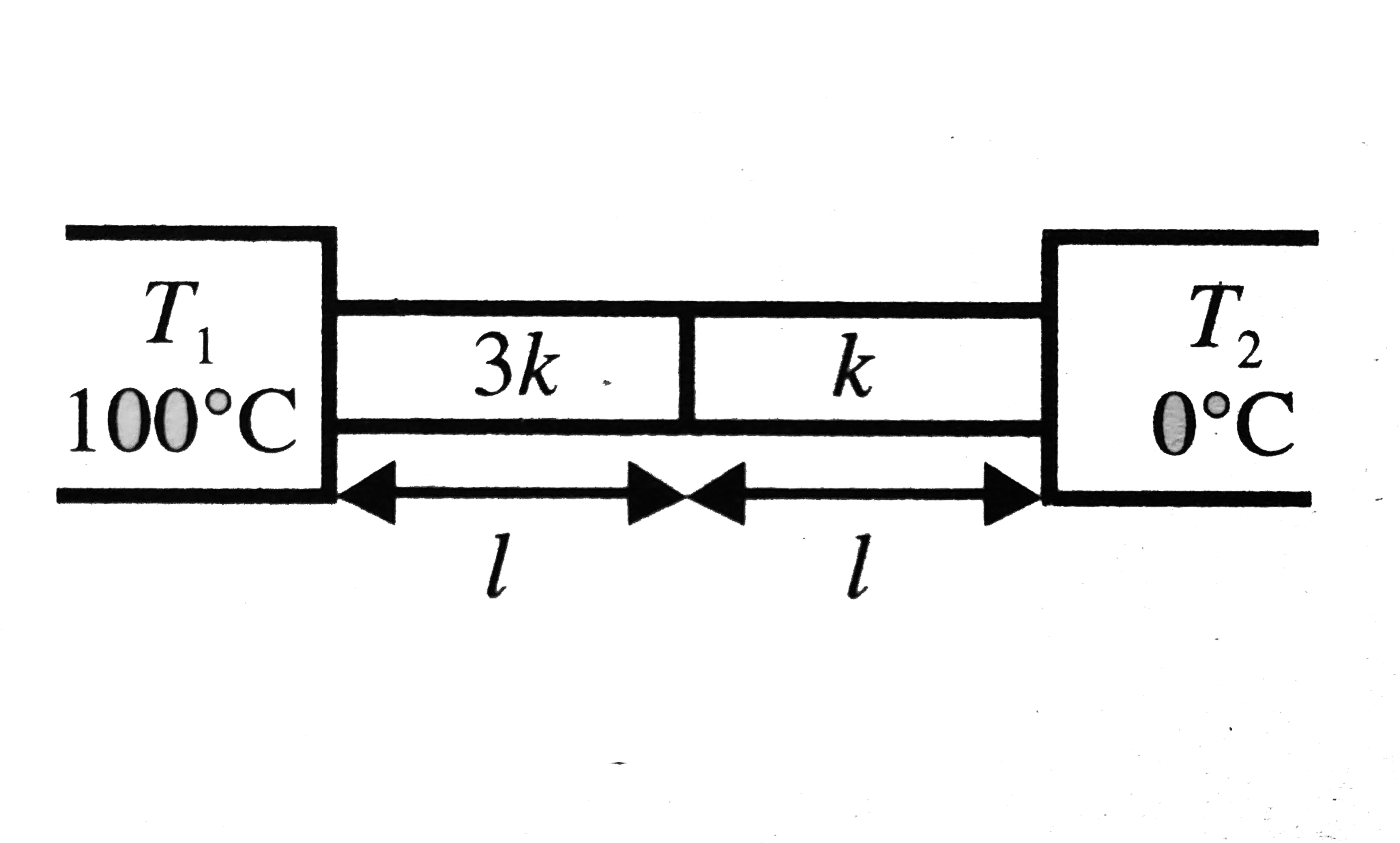
Two rods A and B of same length and cross-sectional area are connected in series and a temperature difference of 100∘C is maintained across the combination as shoen in Fig. If the thermal conductivity of the rod A is 3 k and that of rod B is k, Then
i.Determine the thermal resistance of each rod.
ii. determine the heat current flowing through each rod.
iii. determine the heat current flowing through each rod.
iv. plot the variation of temperature along the length of the rod.
View SolutionTwo rods of the same proper length l0 move toward each other parallel to a common horizontal axis. In the reference frame fixed to one of the rods the time interval between the moments, when the right and left ends of the rods coincide, is equal to Δt. What is the velocity of one rod relative to the other?
View Solution
Knowledge Check
A weightless rigid rod AB of length / connects two equal masses m one particle is fixed at the end B and the other at the middle ofthe rod as shown in the figure-5.131. The rod can rotate in the vertical plane freely around the hinge point/4. Choose the correct option(s)
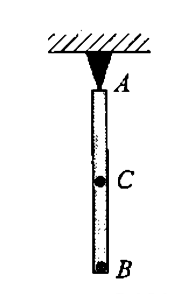 AThe minimum horizontal velocity required to be given to the particle B so as to make the rod go around in the complete vertical circleisBThe minimum horizontal velocity required to begivento the particle B so as to make the rod go around in the complete vertical circle isCThe ratio of compressive force in the rods AC and BC is 2 : 1 when the masses are at highest point.DThe ratio of compressive force in the rodsA
AThe minimum horizontal velocity required to be given to the particle B so as to make the rod go around in the complete vertical circleisBThe minimum horizontal velocity required to begivento the particle B so as to make the rod go around in the complete vertical circle isCThe ratio of compressive force in the rods AC and BC is 2 : 1 when the masses are at highest point.DThe ratio of compressive force in the rodsAA system of two identical rods (L-shaped) of mass m and length l are resting on a peg P as shown in the figure. If the system is displaced in its plane by a small angle θ,find the period of oscillations:
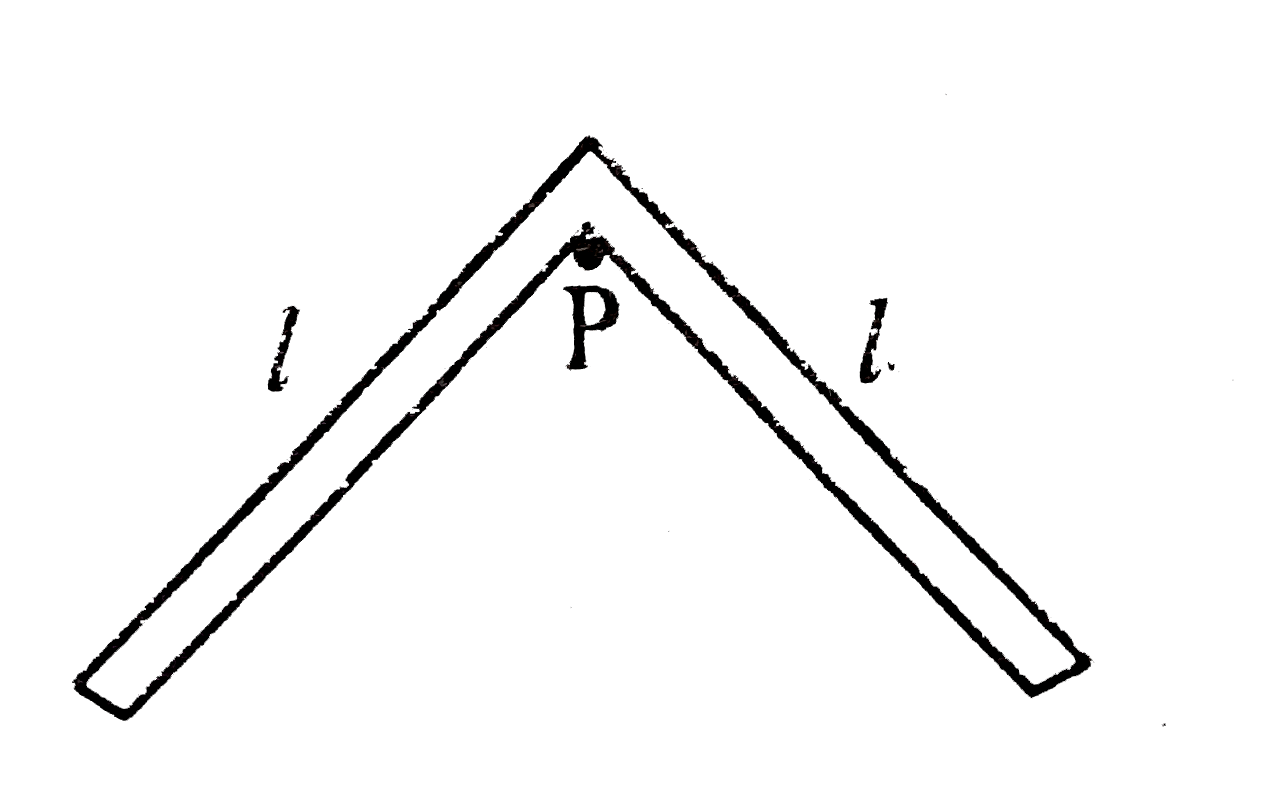 A2π√√2l3gB2π√2√2l3gC2π√2l3gD3π√l3g
A2π√√2l3gB2π√2√2l3gC2π√2l3gD3π√l3gA rod of mass m and length L is kept on a horizontal smooth surface. The rod is hinged at its one end A. There are two springs, perpendicular to the rod, kept in the same horizontal plane. The spring of spring constant k is rigidly attached to the lower end of rod and spring of spring constant 12k just touches the rod at its midpoint C, as shown in the figure. If the rod is slightly displaced leftward and released then, the time period for small osciallation of the rod will be
 Aπ√m3kB3π2√m3kCπ[√m3k+√3g2L]D3π2[√m3k+4√3g2L]
Aπ√m3kB3π2√m3kCπ[√m3k+√3g2L]D3π2[√m3k+4√3g2L]
Three weightless rods of length 1 each are hinged at points A and B lying on the same horizontal and joint through hinges at points C and D (Fig. ). The length AB=2l. A load of mass m is suspended at the hinge C. Determine the minimum force Fmin aplied to the hinge D for which the middle rod remains horizontal.

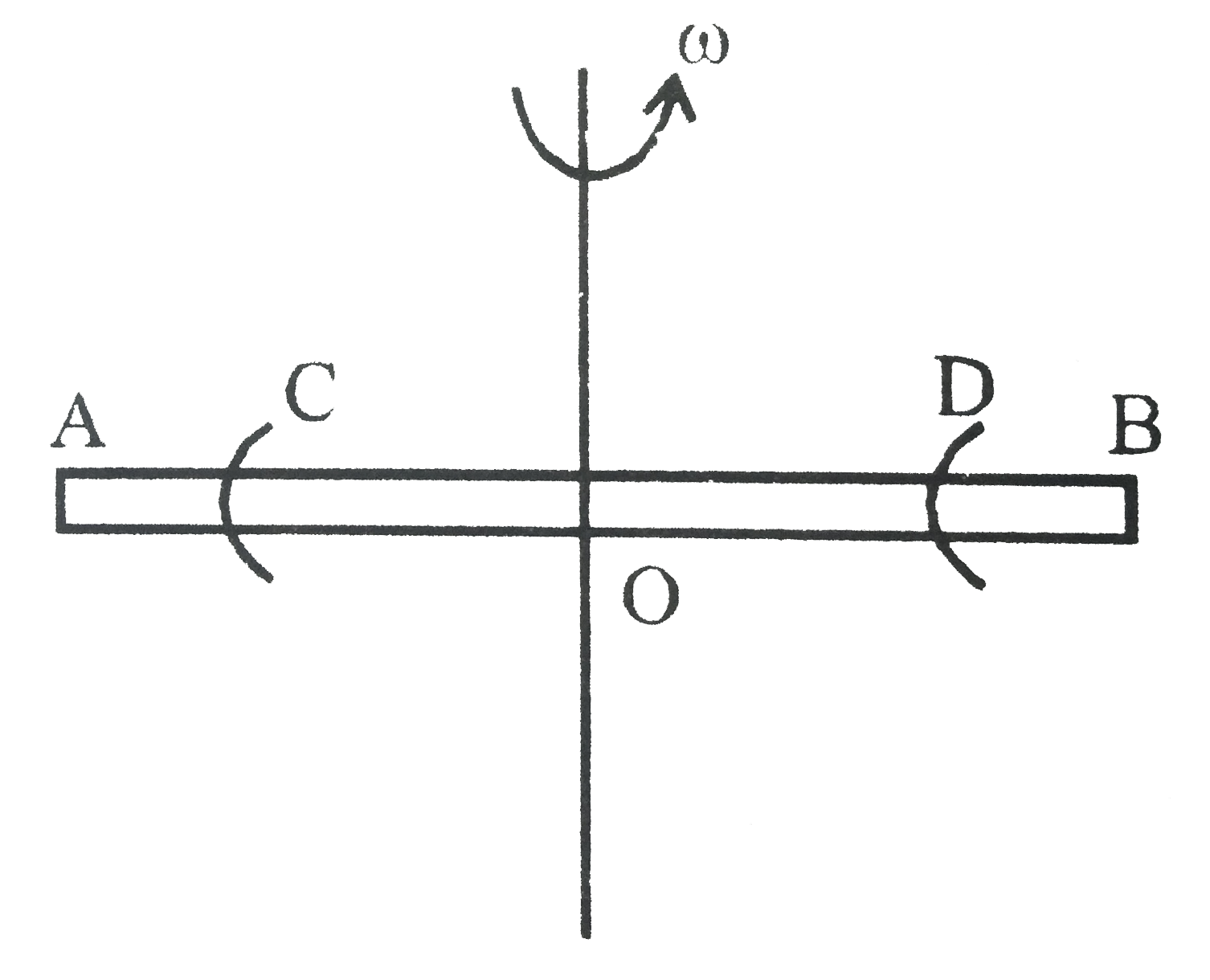
View SolutionA rigid horizontal smooth rod AB of mass 0.75kg and length 40cm can roate freely about a fixed vertical axis through its mid point O. Two rings each of mass 1kg are initially at rest at a distance of 10cm from O on either side of the rod. The rod is set in rotatiob with an angular velocity of 30 radians per second. Find the velocity of each ring along the length of the rod in m/s when they reach the ends of the rod.
 .
.
View SolutionTwo long conducting rods suspended by means of two insulating threads as shown in Fig. are connected at one end to a charged capacitor through a switch S, which initially open. At the other end , they are connected by a loose wire. The capacitor has charge Q and mass per unit length of the rod is λ. The effective resistance of the circuit after closing the switch is R. Find the velocity of each rod when the capacitor is discharged after closing the switch. (Assume that the displacement of rods during the discharging time is negligible.)

View SolutionA uniform rod of mass M and length L is hinged at its lower end so as to rotate freely in the vertical plane of the fig. There is a small tight fitting the hinged end. A small mass less pin welded to the rod supports the bead. The system is released from the vertical position shown. It was observed that the bead just begins to slide on the rod when the rod becomes horizontal.
(a) Find the normal contact force between the rod and the bead when the rod gets horizontal. What is the direction of this force?
(b) Find the coefficient of friction between the bead and rod.
View Solution
A weightless rigid rod with a small iron bob at the end is hinged at point A to the wall so that it can rotate in all directions. The rod is kept in the horizontal position by a vertical inextensible string of length 20cm, fixed at its midpoint. The bob is displaced slightly perpenducular to the plane of the rod and string. Find period of small oscillations of the system in the form πX10s and fill the value of X.
View Solution