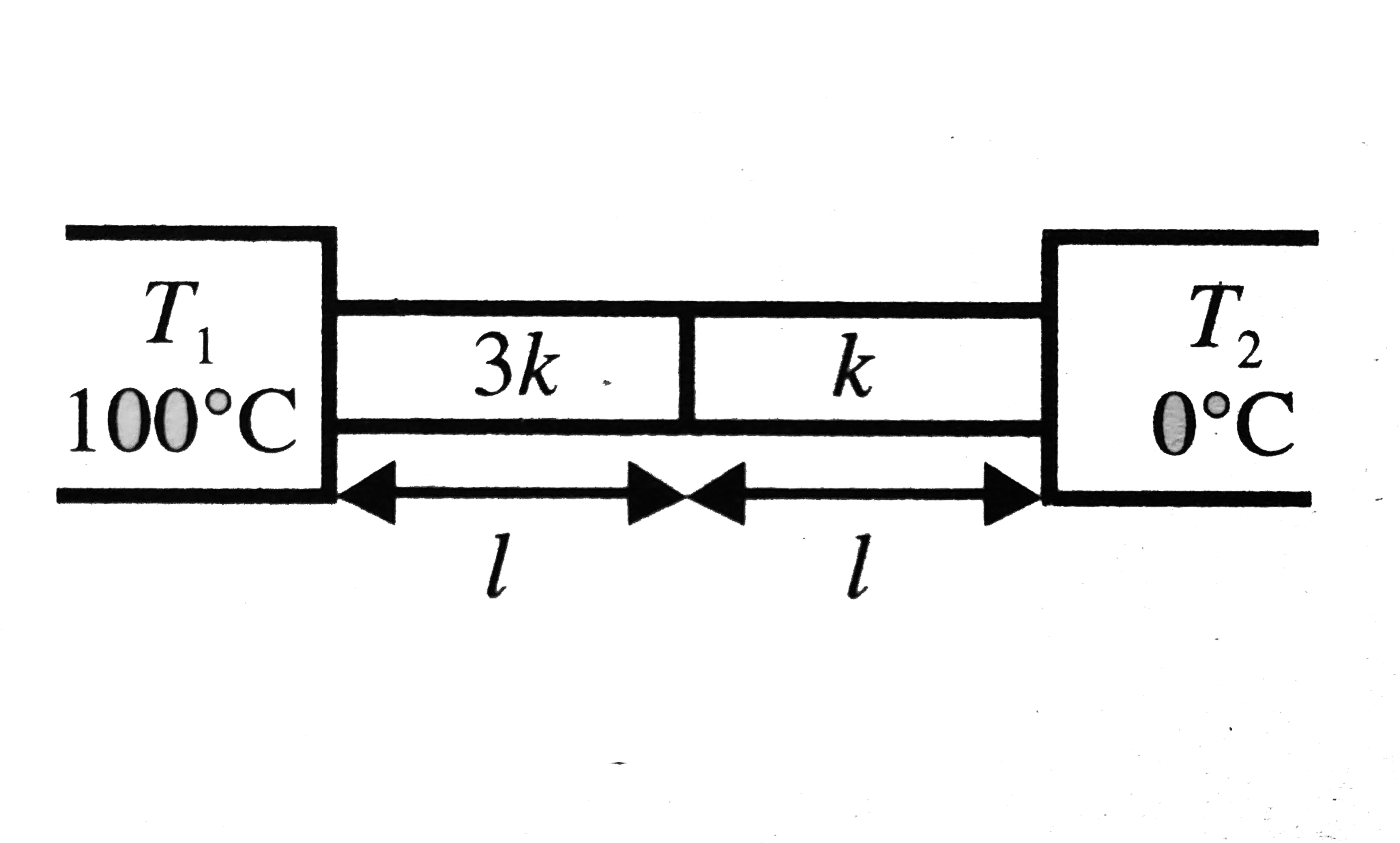
Two rods A and B of same length and cross-sectional area are connected in series and a temperature difference of 100∘C is maintained across the combination as shoen in Fig. If the thermal conductivity of the rod A is 3 k and that of rod B is k, Then
i.Determine the thermal resistance of each rod.
ii. determine the heat current flowing through each rod.
iii. determine the heat current flowing through each rod.
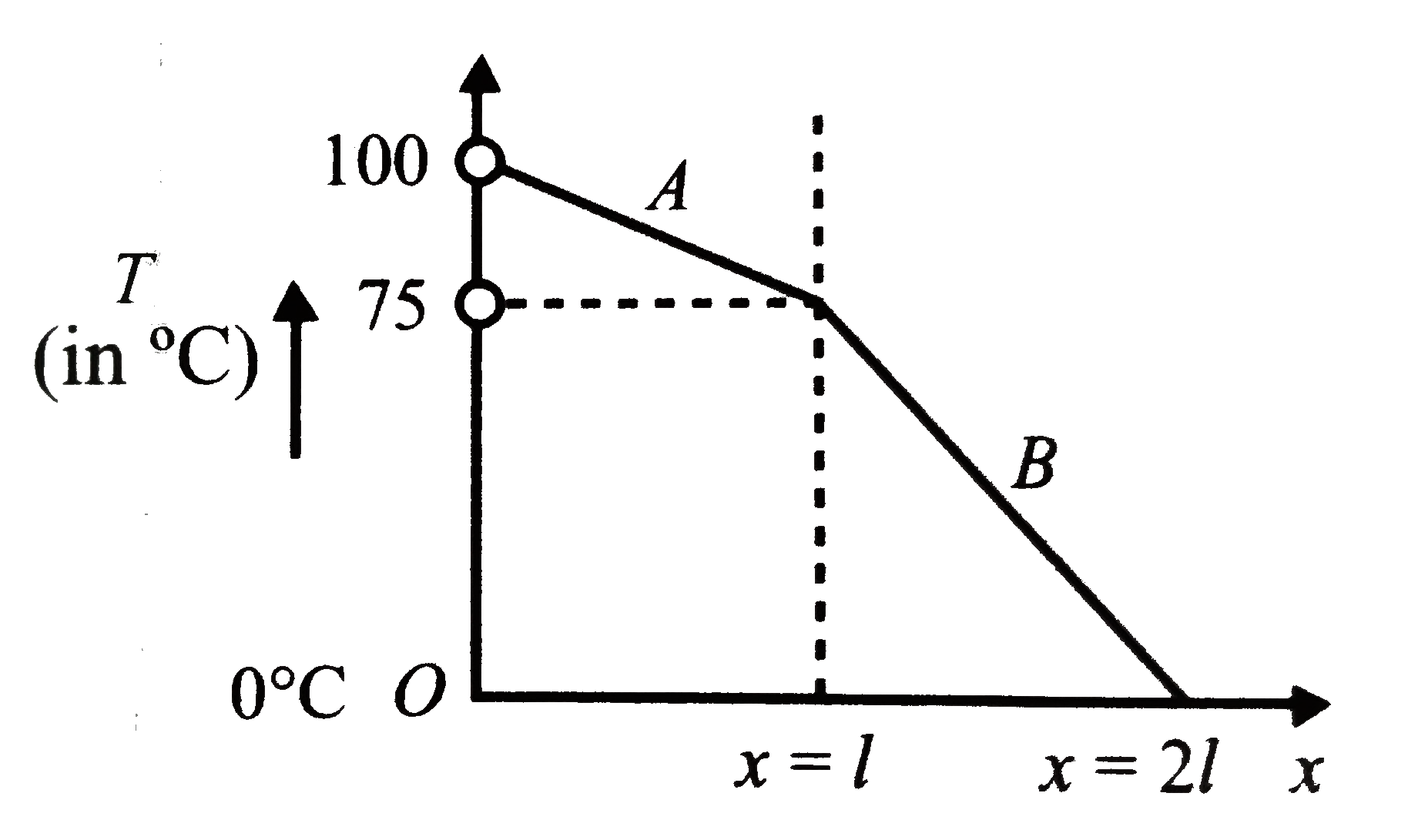
iv. plot the variation of temperature along the length of the rod.
i. Thermal resistance is defined as R=l/kA
For rod A RA=lkAA=l3kA
For rod B RB=lkBA=lkA
ii. Using electrical analogy, the given problem may be modified as The equivalent resistance is R=RA+RB=43lkA
The heat current is given by
I=T1−T2R=100−043lkA=73(kAl)
iii. The temperature of the junction is given by
T=T1−IRA=100−75kAl(l3kA)=75∘C
iv. The variation of temperature along the length of the conductor is shown in Fig.
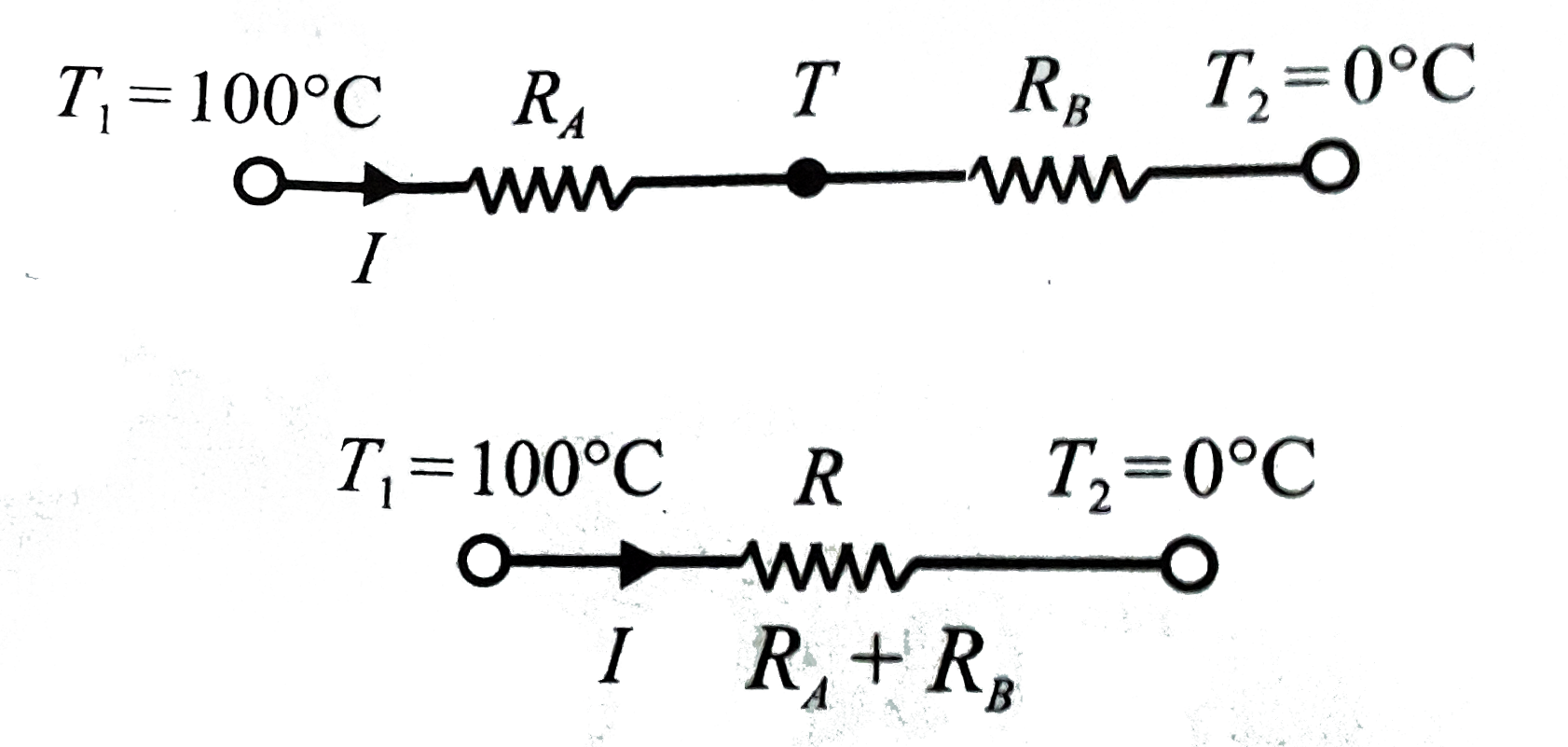
Topper's Solved these Questions
- CALORIMETRYBOOK - CENGAGE PHYSICSCHAPTER - CALORIMETRYEXERCISE - Subjective
25 Videos
- CALORIMETRYBOOK - CENGAGE PHYSICSCHAPTER - CALORIMETRYEXERCISE - Single Correct
148 Videos
- CALORIMETRYBOOK - CENGAGE PHYSICSCHAPTER - CALORIMETRYEXERCISE - Exercise 1.2
22 Videos
- BASIC MATHEMATICSBOOK - CENGAGE PHYSICSCHAPTER - BASIC MATHEMATICSEXERCISE - Exercise 2.6
20 Videos
- CENTRE OF MASSBOOK - CENGAGE PHYSICSCHAPTER - CENTRE OF MASSEXERCISE - INTEGER_TYPE
1 Videos
Similar Questions
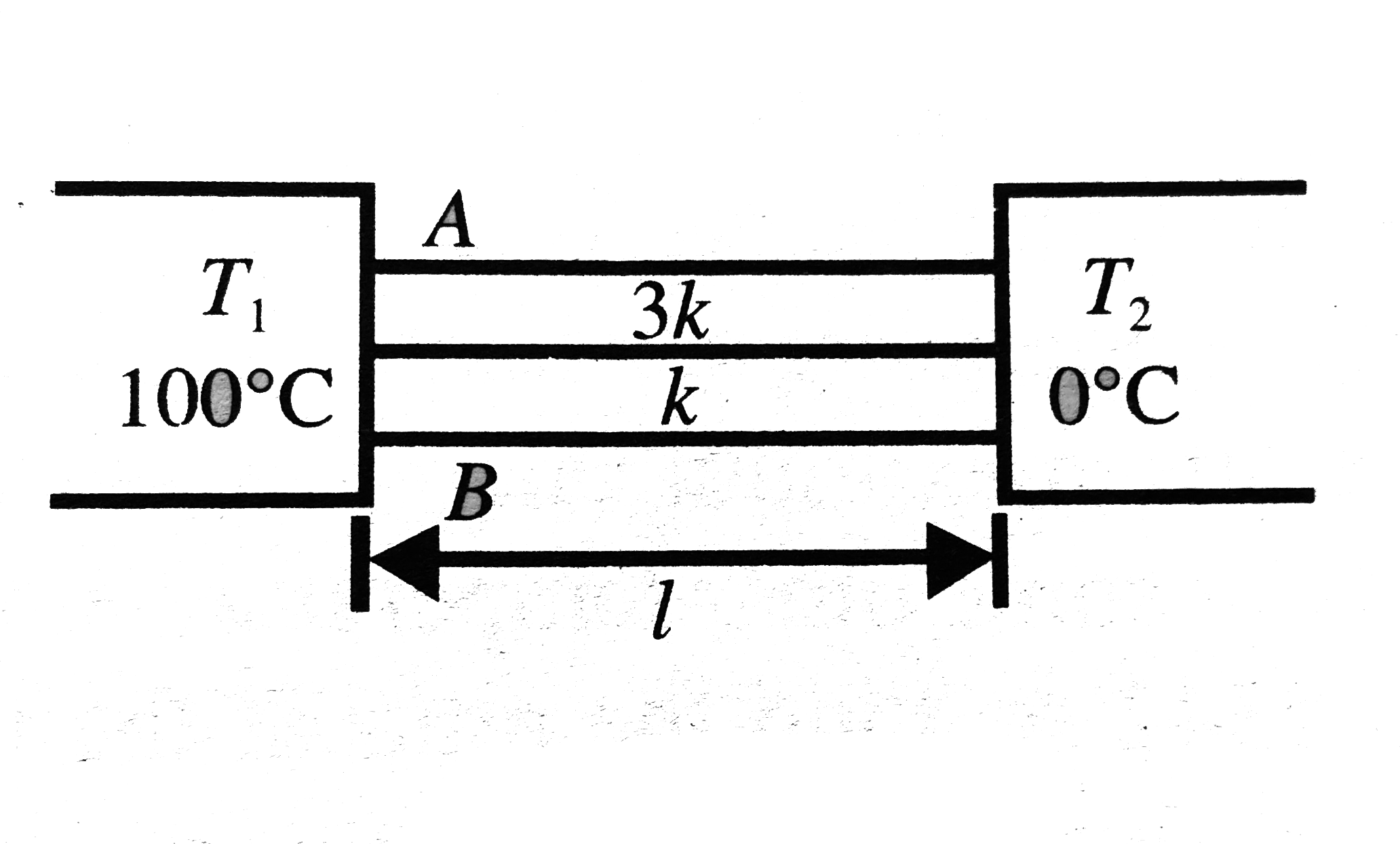
Two conductors A and B given in previous problem are connected in parallel as shown in Fig.
i. Determine the equivalent thermal resistance.
ii. Determine the heat current in each rod.
View SolutionA rod of length1m having cross sections area 0.75m^(2), conducts heat at 6000J/sec.Then the temperature difference across the rod isK=200Wm^(-1)k^(-1)
View Solution
Knowledge Check
Two conducting rods A and B of same length and cross-sectional area are connected (i) In series (ii) In parallel as shown. In both combination a temperature difference of 100°C is maintained. If thermal conductivity of A is 3 K and that of B is K then the ratio of heat current flowing in parallel combination to that flowing in series combination is
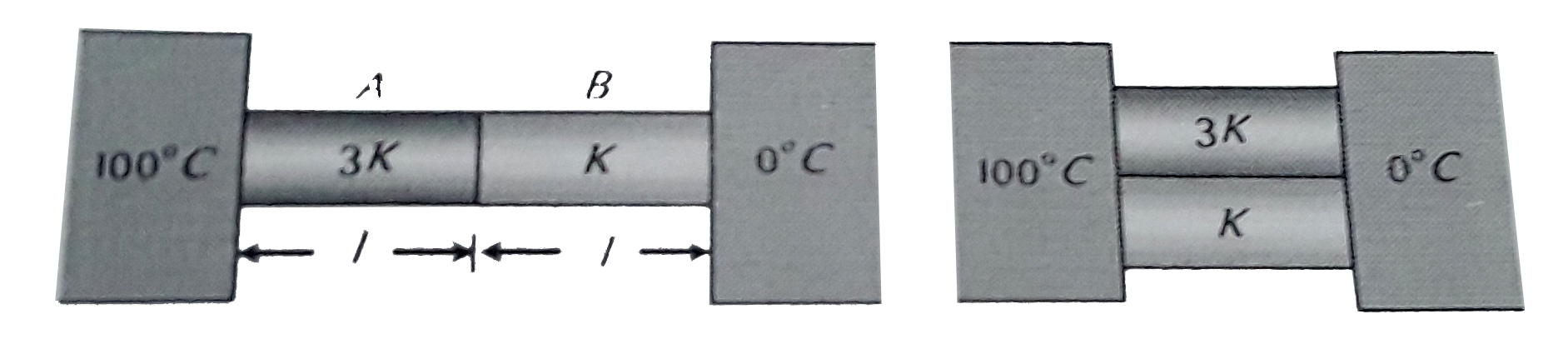 A163B316C11D13
A163B316C11D13Three rods A,B and C of the same length and cross- sectional area are joined in series as shown. The temperature at the junction of A and B, in equilibriu, is
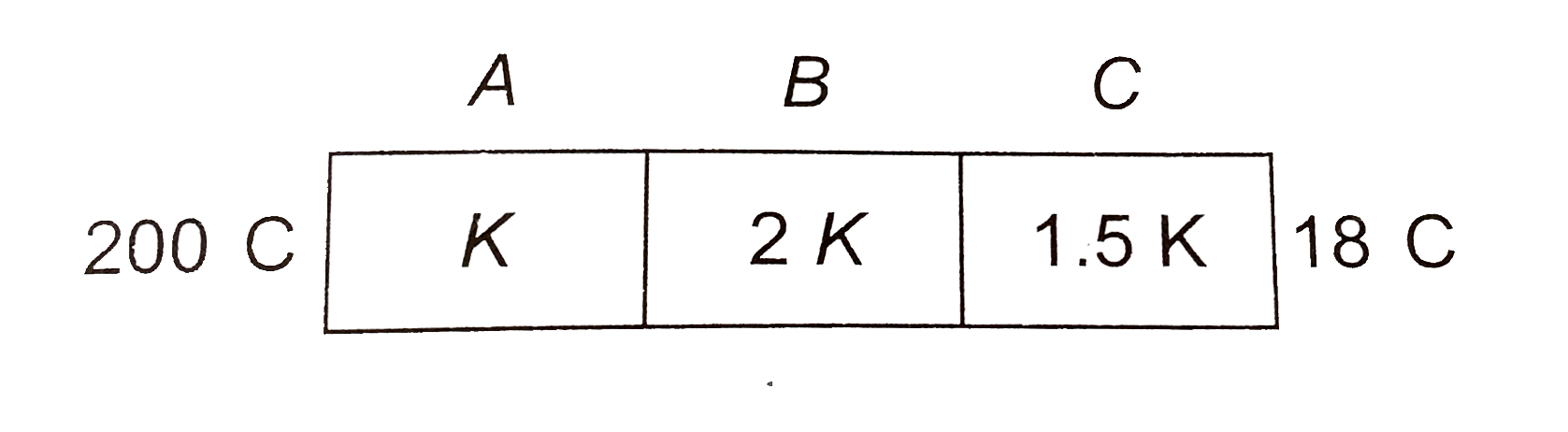 A74∘CB116∘CC156∘CD148∘C
A74∘CB116∘CC156∘CD148∘CThree metal rod A, B and C of same length and cross-section are placed end to end and a temperature difference is maintained between the free ends of A and C. If the thermal conductivity of B(KB) is twice that of C(KC) and half that of A(KA), then the effective thermal conductivity of the system will be
AKA7B6KB7C7KB3D7KC
Two rods A and B of same cross-sectional area A and length l are connected in series between a source (T1=100∘C) and a sink (T2−0∘C) as shown in figure. The rod is laterally insulated.
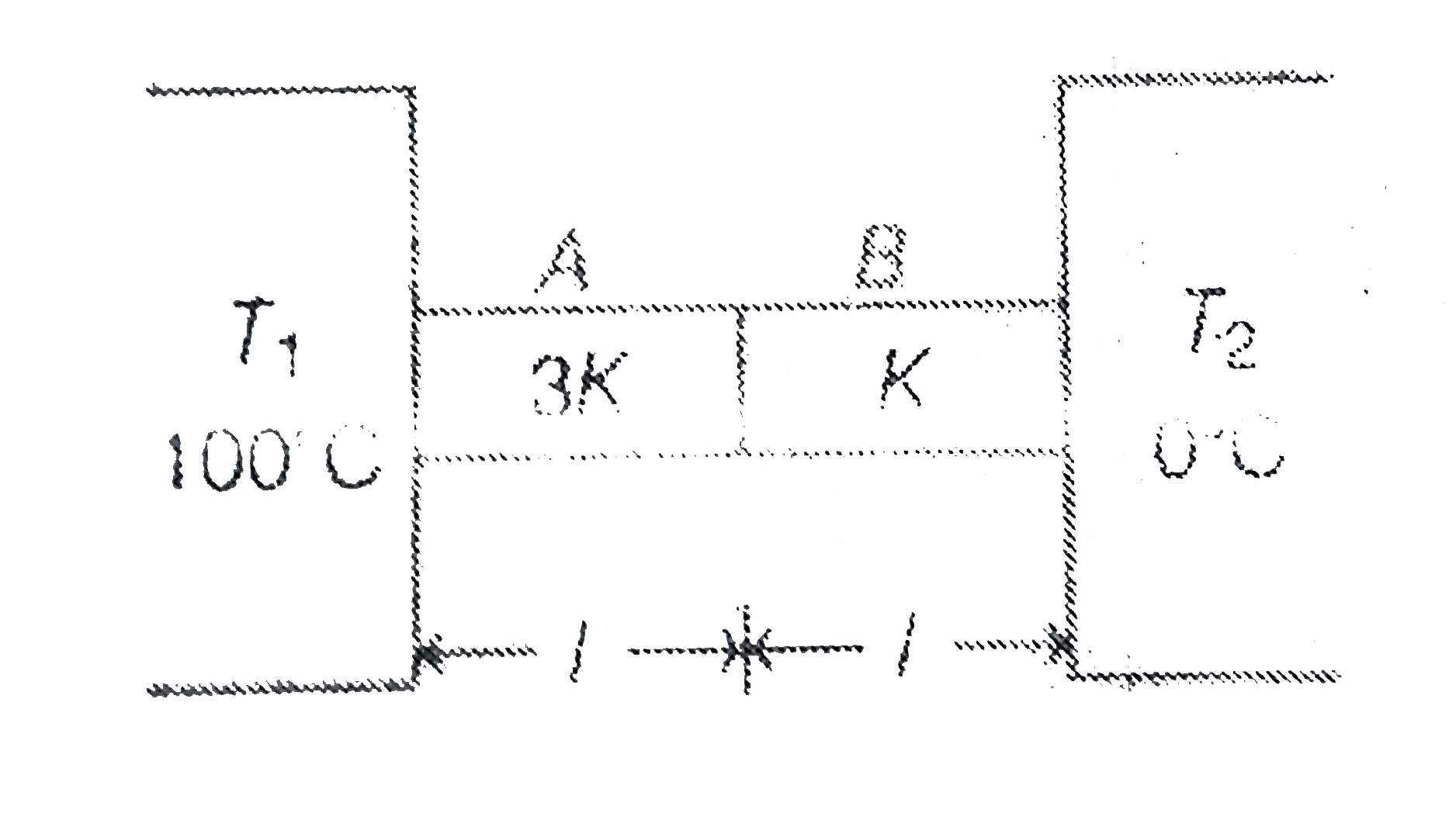
The ratio of the thermal resistance of the rods is
View SolutionTwo rods A and B of same cross sectional are A and length l connected in series between a source
(T1=100∘C) and a sink (T2=0∘C) as shown in The rod is laterally insulated

The ratio of thermal resistance of the rod is .
View SolutionAccording to the experiment of Ingen Hausz the relation between the thermal conductivity of a metal rod is K and the length of the rod whenever the wax melts is
View SolutionThree identical rods A, B and C are placed end to end. A temperature difference is maintained between the free ends of A and C. The thermal conductitvity of B is thrice that of C and half that of A. The effective thermal conductivity of rod A)
View SolutionAccording to the experiment of Ingen Hauze the relation between the thermal conductivity of a metal rod is K and the length of the rod whenever the wax melt is
View Solution
