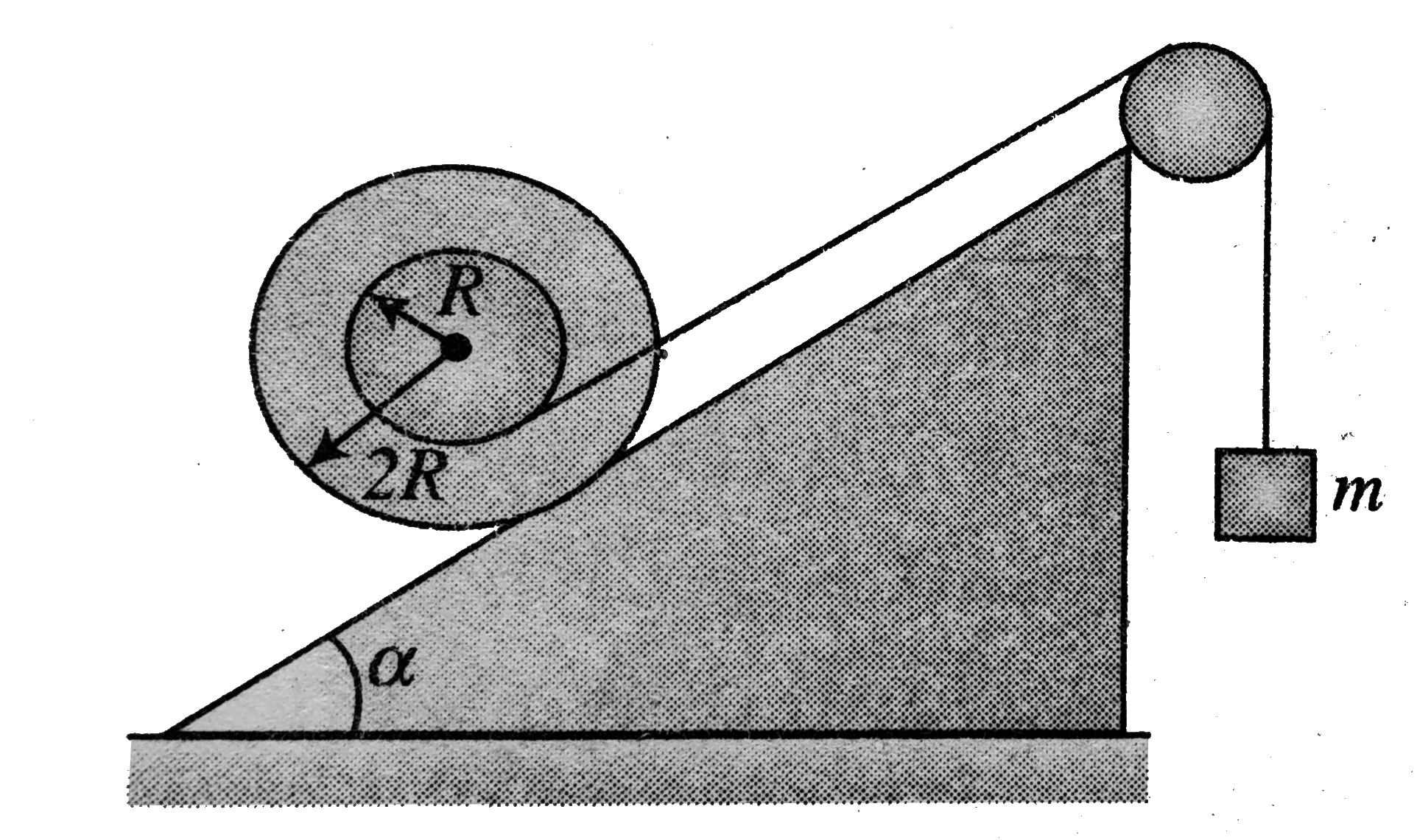
A hollow cylindrical pipe of mass M and radius R has a thin rod of mass m welded inside it, along its length. A light thread is tightly wound on the surface of the pipe. A mass m0 is attached to the end of the thread as shown in figure. The system stays in equilibrium when the cylinder is placed such that α=30∘ The pulley shown in figure is a disc of mass M2
(a) Find the direction and magnitude of friction force acting on the cylinder.
(b) Express mass of the rod ‘m’ in terms of m0
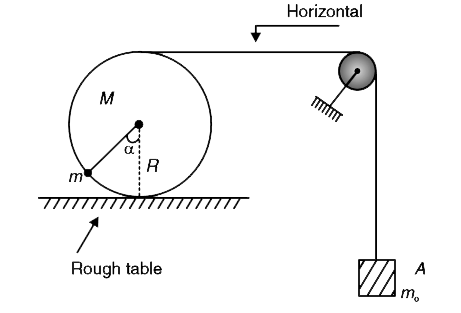
The correct Answer is:(a) f=m0g towards left
(b) m=4m0
Step by step video, text & image solution for A hollow cylindrical pipe of mass M and radius R has a thin rod of mass m welded inside it, along its length. A light thread is tightly wound on the surface of the pipe. A mass m_(0) is attached to the end of the thread as shown in figure. The system stays in equilibrium when the cylinder is placed such that alpha = 30^(@) The pulley shown in figure is a disc of mass (M)/(2) (a) Find the direction and magnitude of friction force acting on the cylinder. (b) Express mass of the rod ‘m’ in terms of m_(0) by Physics experts to help you in doubts & scoring excellent marks in Class 11 exams.
Updated on:21/07/2023Topper's Solved these Questions
Similar Questions
Knowledge Check
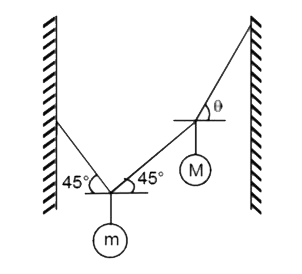
Two masses m and M are attached to the strings as shown in the figure. If the system is in equilibrium, then
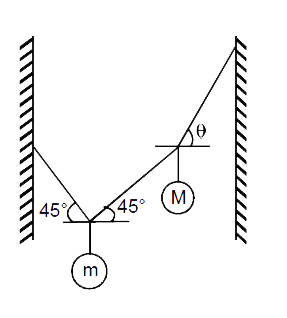 Atanθ=1+2MmBtanθ=1+2mMCcotθ=1+2MmDcotθ=1+2mM
Atanθ=1+2MmBtanθ=1+2mMCcotθ=1+2MmDcotθ=1+2mMA mass m is placed inside a hollow sphere of mass M as shown in figure. The gravitaional force on mass m is
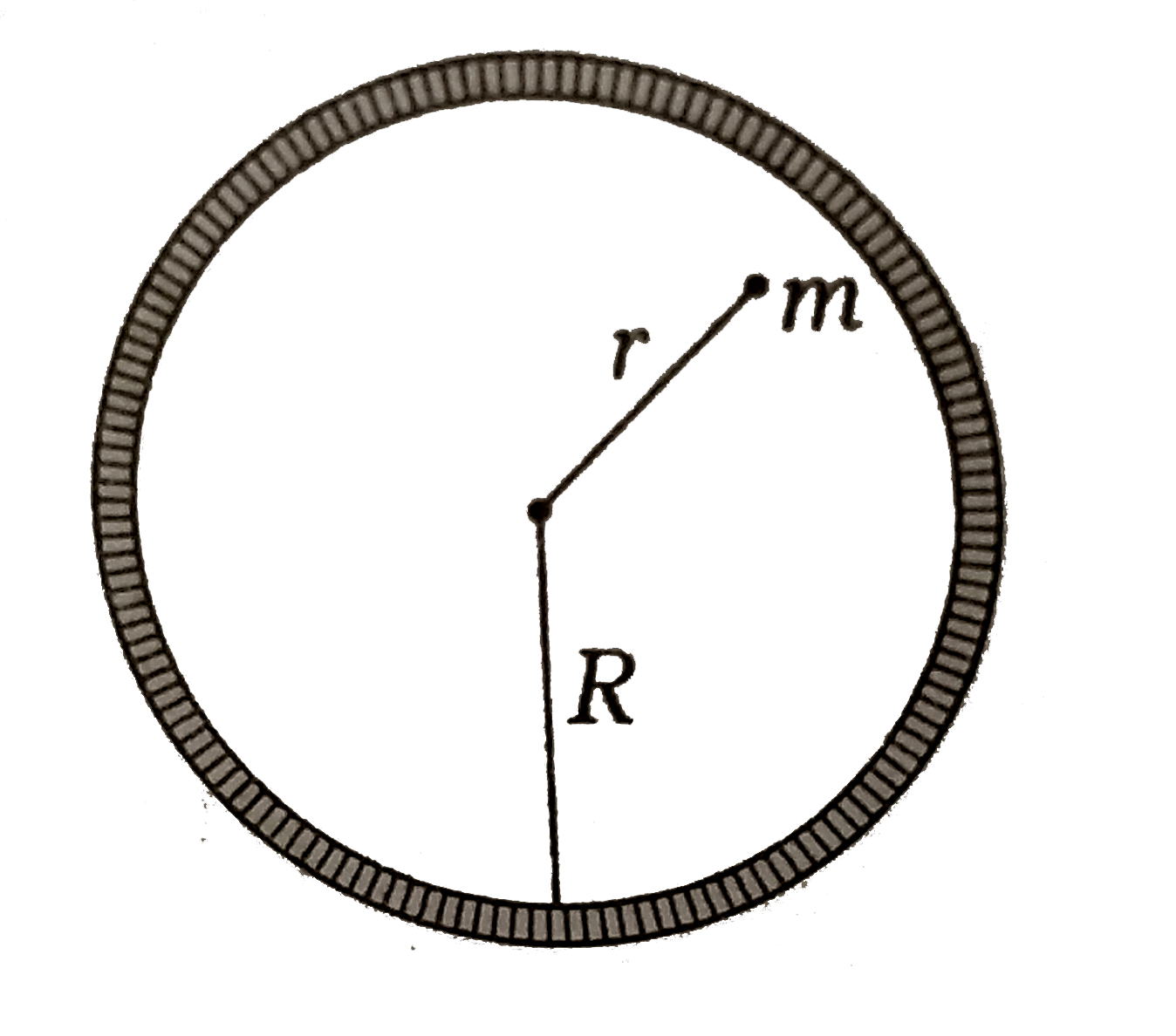 AGMmR2BGMmr2CGMm(R−r)2Dzero
AGMmR2BGMmr2CGMm(R−r)2DzeroA mass m is placed in the cavity inside hollow sphere of mass M as shown in the figure. The gravitational force on m is
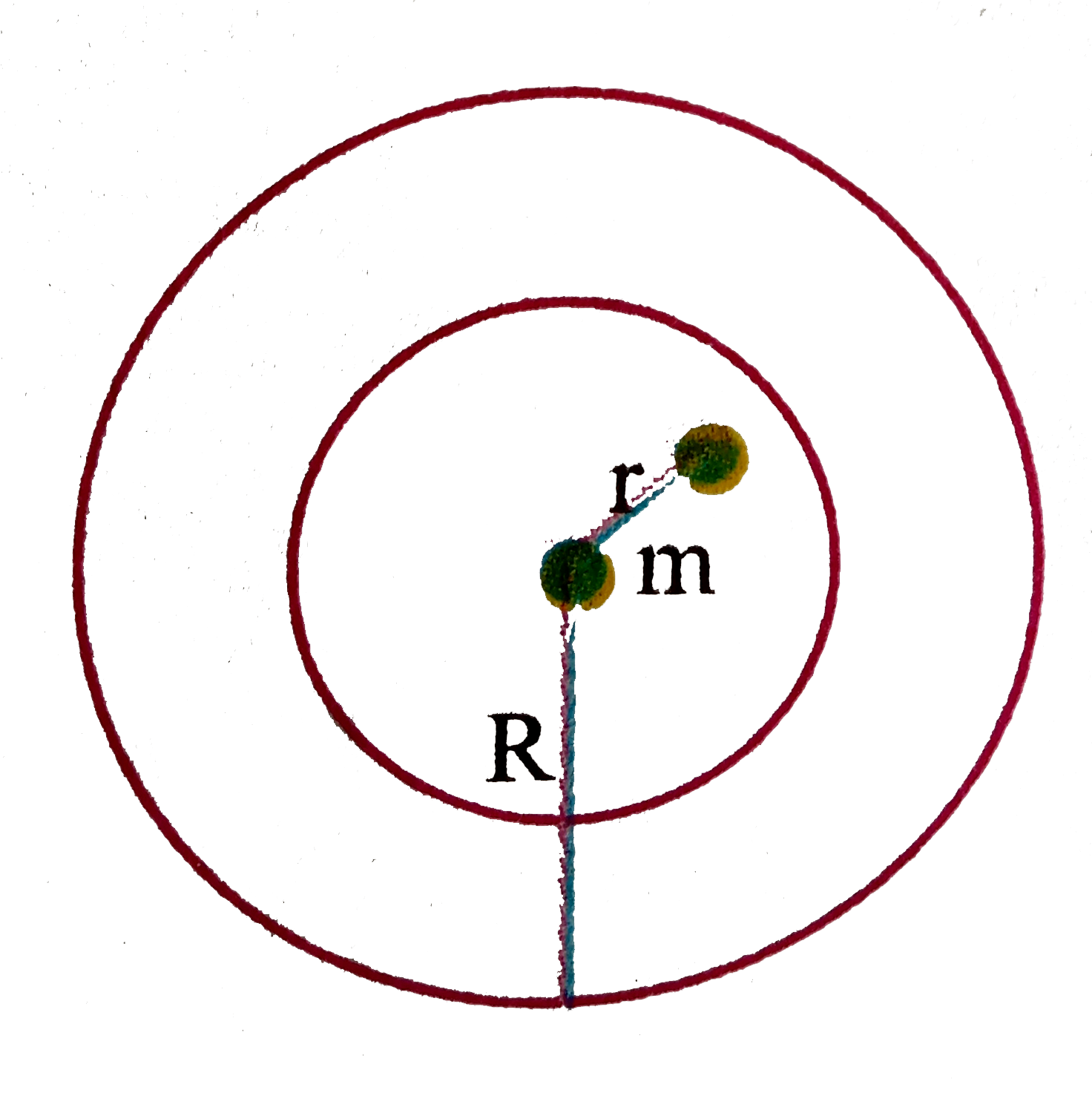 AGMmR2BGMmr2CGMm(R−r)2Dzero
AGMmR2BGMmr2CGMm(R−r)2Dzero
Thread would around cylinder of mass M and radius R. It is allowed to fall as shown. Find its acceleration.
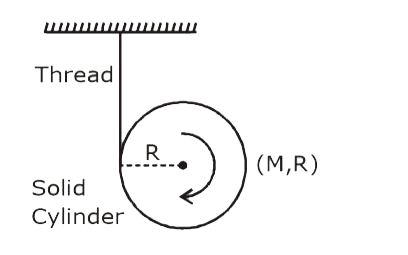
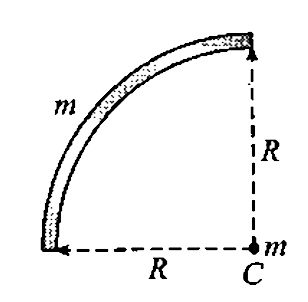
View SolutionFind the force of attraction on a particle of mass m placed at the centre of a quarter ring of mass mand radius R as shown in figure.
View SolutionThree solid sphere of mass M and radius R are placed in contact as shown in figure. Find the potential energy of the system ?
View SolutionThree solid spheres of mass M and radius R are placed in contact as shown in figure. Find the potential energy of the system ?
View SolutionA spool of mass M and radius 2R lies on an inclined plane as shown in the figure. A light thread is wound around the connecting tube of the spool and its free end carries a weight of mass m. The value of m so that system is in equilibrium is
View Solution