A solid body A of mass m and specific heat capacity ‘s’ has temperature T1=400K. It is placed, at time t=0, in atmosphere having temperature T0=300K. It cools, following Newton’s law of cooling and its temperature was found to be T2=350Kat time t0. At time t0, the body A is connected to a large water bath maintained at atmospheric temperature T0, using a conducting rod of length L, cross section A and thermal conductivity k. The cross sectional area A of the connecting rod is small compared to the overall surface area of body A. Find the temperature of A at time t=2t0.
The correct Answer is:T=300+50e−[In2t0+kAmsL]t0
Step by step video, text & image solution for A solid body A of mass m and specific heat capacity ‘s’ has temperature T_(1) = 400 K. It is placed, at time t = 0, in atmosphere having temperature T_(0) = 300 K. It cools, following Newton’s law of cooling and its temperature was found to be T_(2) = 350 K at time t_(0). At time t_(0), the body A is connected to a large water bath maintained at atmospheric temperature T_(0), using a conducting rod of length L, cross section A and thermal conductivity k. The cross sectional area A of the connecting rod is small compared to the overall surface area of body A. Find the temperature of A at time t = 2t_(0). by Physics experts to help you in doubts & scoring excellent marks in Class 11 exams.
Updated on:21/07/2023Topper's Solved these Questions
Similar Questions
A solid body X of heat capacity C is kept in an atmosphere whose temperature is TA=300K. At time t=0 the temperature of X is T0=400K. It cools according to Newton's law of cooling. At time t1, its temperature is found to be 350K. At this time (t1), the body X is connected to a large box Y at atmospheric temperature is T4, through a conducting rod of length L, cross-sectional area A and thermal conductivity K. The heat capacity Y is so large that any variation in its temperature may be neglected. The cross-sectional area A of hte connecting rod is small compared to the surface area of X. Find the temperature of X at time t=3t1.
View SolutionWhen two bodies ofmasses m1 and m2 with specific heats s1 and s2 at absolute temperatures T10 and T20(T10>T20) are connected by a rod of length l and cross sectional area A with thermal conductivity k. Find the temperature difference of the bodies after time t. Neglect any heat loss due to radiation at any surface..

View Solution
Knowledge Check
A perfectly black body emits radiation at temperature T1K. If is to radiate at 16 times this power, its temperature T2K should be
AT2=4T1BT2=8T1CT2=2T1DT2=16T1A liquid of mass M and specific heat S is at a temperature 2T. Another liquid of thermal capacity 1.5× the first liquid at a temperature T3 is added to it. The resultant temperature of the mixture will be
A2T3BT2CTD4T3If the temperature of the body θ and time of cooling 't' and room temperature of the surrounding θ0. The cooling curve is
A B
B C
C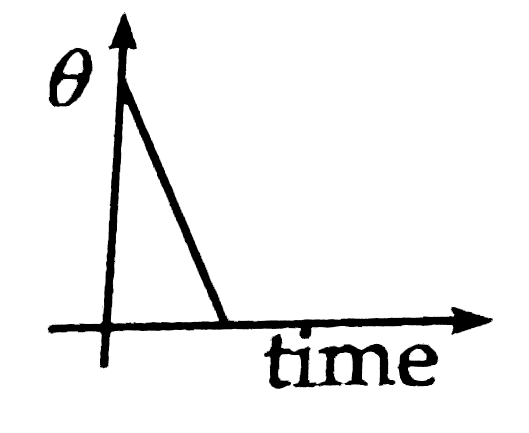 D
D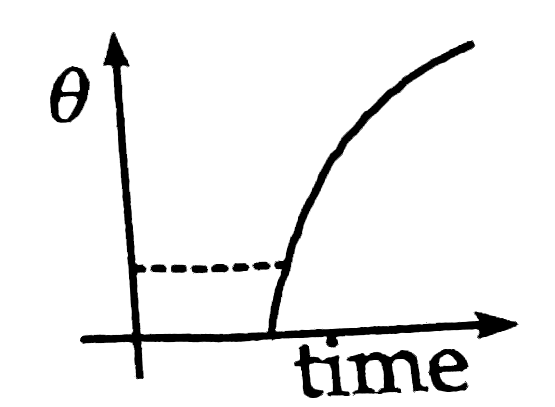
Figure-4.13 shows a water tank at a constant temperature. T0 and a small bodyof mass m, and specific heat S at a temperature T1 . Given that T1<T0. A metal rod of length L, cross-sectional area A whose thermal conductivity is K is placed between the tank and the body to connect than. Find the temperature of body as a function of time. Given that the heat capacity of rod is negligible.
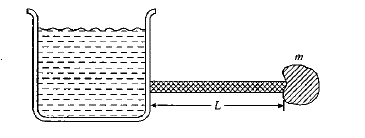
View SolutionKinetic energy of a charged particle is zero at time t0. K at time 2 t0. 2K at time 3 t0. It remains constant (i.e.2K) upto 4 t0. Choose the correct option.
View SolutionA liquid in a beaker has temperature θ(t) at time t and θ0 is temperature of surroundings, then according to Newton's law of cooling the correct graph between loge(θ−θ0) and t is :
View SolutionA body cools from a temperature 3T to 2T in 10 minutes. The room temperature is T. Assume that Newton's law of cooling is applicable. The temperature of the body at the end of next 10 minutes will be
View SolutionA body with an initial temperature θ1 is allowed to cool in a surrounding which is at a constant temperature of θ0(θ<θ1) Assume that Newton's law of cooling is obeyed Let k= constant The temperature of the body after time t is best experssed by .
View Solution