A Carnot cycle based ideal heat engine operates between two tanks each having same mass m of water. The source tank has an initial temperature of T1=361K and the sink tank has an initial temperature of T2=289K. Assume that the two tanks are isolated from the surrounding and exchange heat with the engine only. Specific heat of water is s.
(a) Find the final common temperature of the two tanks.
(b) Find the total work that the engine will be able to deliver by the time the two tanks reach common temperature.
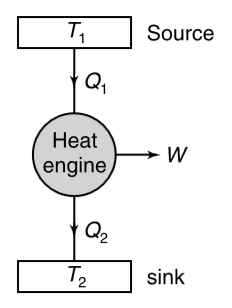
The correct Answer is:(a) T0=√T1T2=323K
(b) W=ms (T1+T2−2√T1T2=4ms
Step by step video, text & image solution for A Carnot cycle based ideal heat engine operates between two tanks each having same mass m of water. The source tank has an initial temperature of T_(1) = 361 K and the sink tank has an initial temperature of T_(2) = 289 K. Assume that the two tanks are isolated from the surrounding and exchange heat with the engine only. Specific heat of water is s. (a) Find the final common temperature of the two tanks. (b) Find the total work that the engine will be able to deliver by the time the two tanks reach common temperature. by Physics experts to help you in doubts & scoring excellent marks in Class 11 exams.
Updated on:21/07/2023Topper's Solved these Questions
- FIRST LAW OF THERMODYNAMICSBook:ARIHANTChapter:FIRST LAW OF THERMODYNAMICSExercise:Level 3
Explore 6 Videos
- FIRST LAW OF THERMODYNAMICSBook:ARIHANTChapter:FIRST LAW OF THERMODYNAMICSExercise:Level 3
Explore 6 Videos
- ELASTICITYBook:ARIHANTChapter:ELASTICITYExercise:Level 3
Explore 1 Video
- FLUIDSBook:ARIHANTChapter:FLUIDSExercise:All Questions
Explore 70 Videos
Similar Questions
An adiabatic cylinder of cross section A is fitted with a mass less conducting piston of thickness d and thermal conductivity K. Initially, a monatomic gas at temperature T0 and pressure P0 occupies a volume V0 in the cylinder. The atmospheric pressure is P0 and the atmospheric temperature is T1(>T0). Find
(a) the temperature of the gas as a function of time
(b) the height raised by the piston as a function of time.
Neglect friction and heat capacities of the container and the piston.
View SolutionAn adiabatic cylinder of cross section A is fitted with a mass less conducting piston of thickness d and thermal conductivity K. Initially, a monatomic gas at temperature T0 and pressure P0 occupies a volume V0 in the cylinder. The atmospheric pressure is P0 and the atmospheric temperature is T1(>T0). Find
(a) the temperature of the gas as a function of time
(b) the height raised by the piston as a function of time.
Neglect friction and heat capacities of the container and the piston.
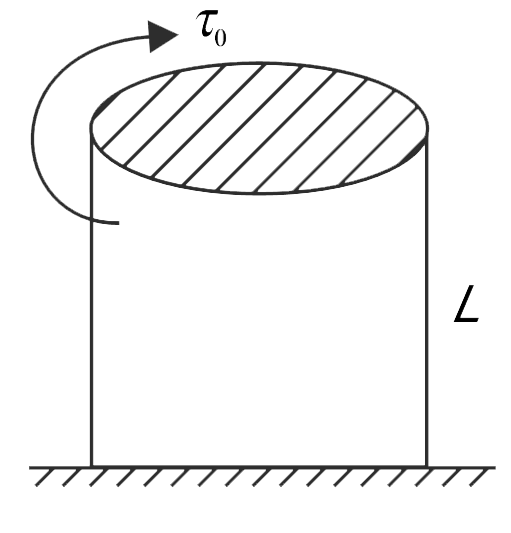
View SolutionA metal cylinder of length L and radius R is fixed rigidly to ground with its axis vertical. A twisting torque τ0 is applied along the circumference at the top of the cylinder. This causes an angular twist of θ0 (rad) in the top surface. Calculate the shear modulus of elasticity (η) of the material of the cylinder.
View SolutionWe know that the atmospheric pressure on the surface of the earth is because of weight, of the air. The radius of the earth is 6400 km and atmospheric pressure on the sruface of earth is 1×105N/m2. Estimate the mass of the earth's atmosphere assuming that acceleration due to gravity remains constant at 10m/s2 over the entrie height of the atmospehre.
View SolutionAn ideal heat engine working between temperature T_(1) and T_(2) has an efficiency eta , the new efficiency if both the source and sink temperature are doubled, will be
View SolutionAn ideal heat engine working between temperature T_(H) and T_(L) has efficiency eta . If both the temperature are raised by 100 K each the new efficiency of heat engine will be
View SolutionTwo Carnot engines are operated in succession. The first engine receives heat from a source at T=800K and rejects to sink at T2K. The second engine receives heat rejected by the first engine and rejects to another sink at T3=300K. If work outputs of the two engines are equal, then find the value of T2.
View SolutionTwo Carnot engines A and B are operated in series. The engine A receives heat from the source at temperature T1 and rejects the heat to the sink at temperature T. The second engine B receives the heat at temperature T and rejects to its sink at temperature T2. For what value of T the efficiencies of the two engines are equal?
View SolutionThe efficiency of carnot's heat engine is 0.5 when the temperature of the source is T1 and that of sink is T2 .The efficiency of another carnot's heat engine is also 0.5.the temperature of source and sink of the second engine are respectively
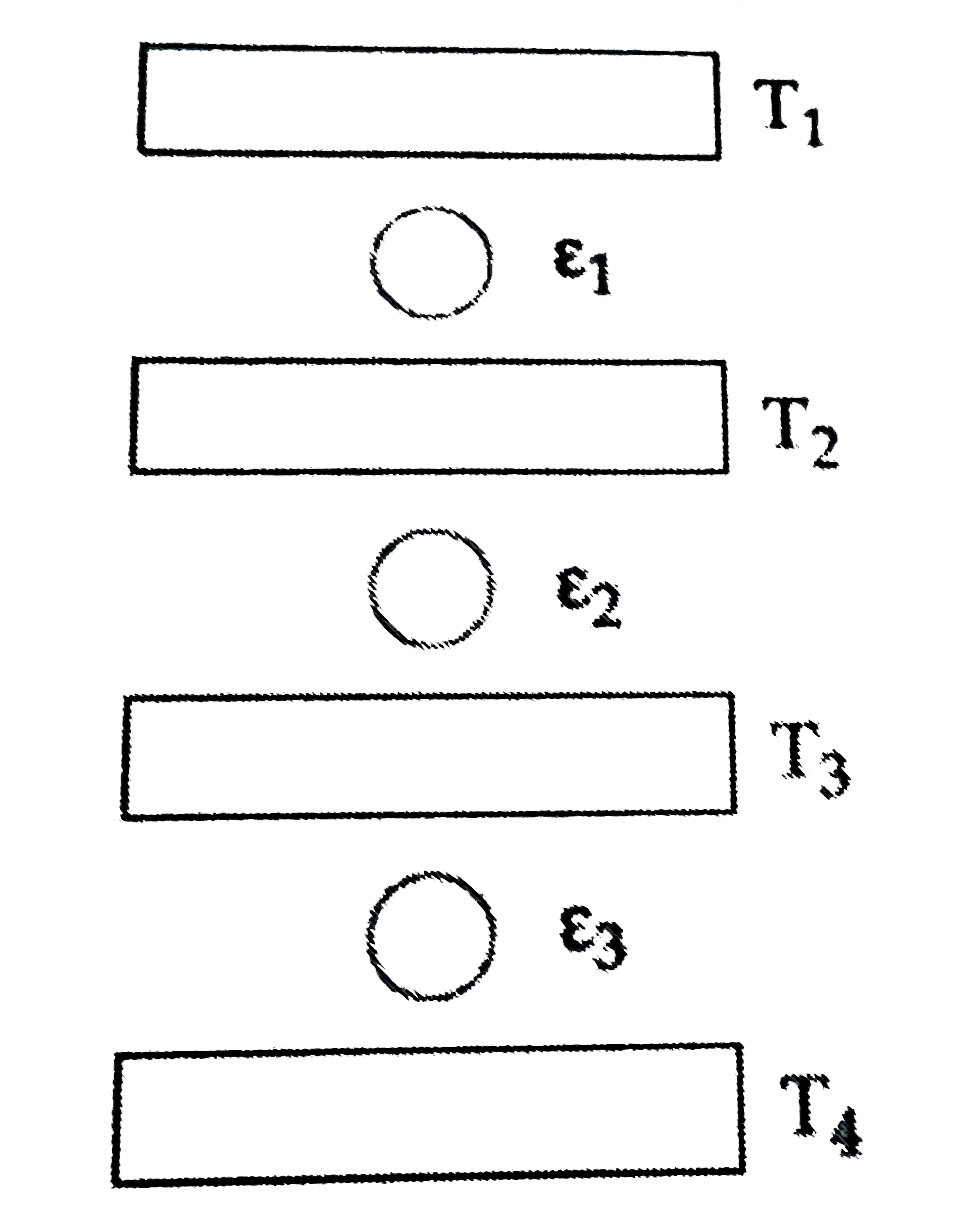
View SolutionThree Carnot engines operate in series between a heat source at a temperature T1 and a heat sink at temperature T4 (see figure). There are two other reservoirs at temperature T2andT3, as shown, with T1>T2>T3>T4. The three engines are equally efficient if :
View Solutionएक कार्नो इंजन 627∘ ताप वाले स्रोत से 3000 किलोकैलोरी ऊष्मा लेता हैं तथा 27∘ ताप वाले कुण्ड को ऊष्मा निष्काषित करता हैं । ज्ञात कीजिए - (A) इंजन की दक्षता , (B) कुण्ड को निष्काषित ऊष्मा , (C) कृत कार्य जूल में ।
View Solutionएक अनुत्क्रमणीय ऊष्मा इंजन T1 K पर ऊष्मा स्त्रोत से ऊष्मा लेता हैं तथा T2 K पर ऊष्मा सिंक को दे देता हैं । इसकी दक्षता n होगी -
View SolutionTwo Carnot engines A and B are operated in series. The first one, A, receives heat at T_(1) (=600 K) and rejects to a reservoir at temperature T_2 . The second engine and, in turn, rejects to a heat reservoir at T_(3) (=400 K) Calculate the temperature T_(2) (in K) if the work outputs of the two engines are equal.
View SolutionTwo ideal Carnot engines operate in cascade (all heat given up by one engine is used by the other engine to produce work) between temperatures, T1andT2. The temperature of the hot reservoir of the first engine is T1 and the temperature of the cold reservoir of the second engine is T2. T is temperature of the sink of first engine which is also the source for the second engine. How is T related to T1andT2, if both the engines perform equal amount of work?
View SolutionTwo Carnot engines A and B are operated in series. The engine A receives heat from the source at temperature T_(1) and rejects the heat to the sink at temperature T. The second engine B receives the heat at temperature T and rejects to its sink at temperature T_(2) . For what value of T the efficiencies of the two engines are equal.
View Solution