A room air conditioner is a Carnot cycle based heat engine run is reverse. An amount of heat Q2 is absorbed from the room at a temperature T2 into coils having a working gas (these gases are not good for environment!). The gas is compressed adiabatically to the outside temperature T1. Then the gas is compressed isothermally in the unit outside the room, giving off an amount of heat Q1. The gas expands adiabatically back to the temperatureT2 and the cycle is repeated. The electric motor electric consumes power P.
(i) Find the maximum rate at which heat can be removed from the room.
(i) Heat flows into the room at a constant rate of kΔT where k is a constant and ΔT is temperature difference between the outside and inside of the room. Find the smallest possible room temperature in terms of T1,k and P.
The correct Answer is:(i)P(T2T1−T2)
(ii)T1−Pk[√1+(4kT1)−1
Step by step video, text & image solution for A room air conditioner is a Carnot cycle based heat engine run is reverse. An amount of heat Q_(2) is absorbed from the room at a temperature T_(2) into coils having a working gas (these gases are not good for environment!). The gas is compressed adiabatically to the outside temperature T_(1). Then the gas is compressed isothermally in the unit outside the room, giving off an amount of heat Q_(1). The gas expands adiabatically back to the temperatureT_(2) and the cycle is repeated. The electric motor electric consumes power P. (i) Find the maximum rate at which heat can be removed from the room. (i) Heat flows into the room at a constant rate of k DeltaT where k is a constant and Delta T is temperature difference between the outside and inside of the room. Find the smallest possible room temperature in terms of T_(1), k and P. by Physics experts to help you in doubts & scoring excellent marks in Class 11 exams.
Updated on:21/07/2023Topper's Solved these Questions
- FIRST LAW OF THERMODYNAMICSBOOK - ARIHANTCHAPTER - FIRST LAW OF THERMODYNAMICSEXERCISE - Level 3
6 Videos
- FIRST LAW OF THERMODYNAMICSBOOK - ARIHANTCHAPTER - FIRST LAW OF THERMODYNAMICSEXERCISE - Level 3
6 Videos
- ELASTICITYBOOK - ARIHANTCHAPTER - ELASTICITYEXERCISE - Level 3
1 Videos
- FLUIDSBOOK - ARIHANTCHAPTER - FLUIDSEXERCISE - All Questions
70 Videos
Similar Questions
An adiabatic cylinder of cross section A is fitted with a mass less conducting piston of thickness d and thermal conductivity K. Initially, a monatomic gas at temperature T0 and pressure P0 occupies a volume V0 in the cylinder. The atmospheric pressure is P0 and the atmospheric temperature is T1(>T0). Find
(a) the temperature of the gas as a function of time
(b) the height raised by the piston as a function of time.
Neglect friction and heat capacities of the container and the piston.
View SolutionAn adiabatic cylinder of cross section A is fitted with a mass less conducting piston of thickness d and thermal conductivity K. Initially, a monatomic gas at temperature T0 and pressure P0 occupies a volume V0 in the cylinder. The atmospheric pressure is P0 and the atmospheric temperature is T1(>T0). Find
(a) the temperature of the gas as a function of time
(b) the height raised by the piston as a function of time.
Neglect friction and heat capacities of the container and the piston.
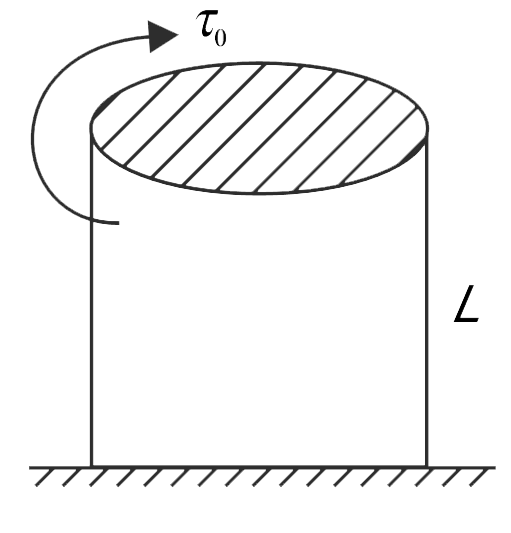
View SolutionA metal cylinder of length L and radius R is fixed rigidly to ground with its axis vertical. A twisting torque τ0 is applied along the circumference at the top of the cylinder. This causes an angular twist of θ0 (rad) in the top surface. Calculate the shear modulus of elasticity (η) of the material of the cylinder.
View SolutionWe know that the atmospheric pressure on the surface of the earth is because of weight, of the air. The radius of the earth is 6400 km and atmospheric pressure on the sruface of earth is 1×105N/m2. Estimate the mass of the earth's atmosphere assuming that acceleration due to gravity remains constant at 10m/s2 over the entrie height of the atmospehre.
View SolutionA heat engine absorbs heat Q_(1) at temperature T_(1) and Q_(2) at temperature T_(2) . Work done by the engine is (Q_(1)+Q_(1)) . This data:
View SolutionThe efficiency of a heat engine is defined as the ratio of the mechanical work done by the engine in one cycle to the heat absorbed from the high temperature source . eta = (W)/(Q_(1)) = (Q_(1) - Q_(2))/(Q_(1)) Cornot devised an ideal engine which is based on a reversible cycle of four operations in succession: isothermal expansion , adiabatic expansion. isothermal compression and adiabatic compression. For carnot cycle (Q_(1))/(T_(1)) = (Q_(2))/(T_(2)) . Thus eta = (Q_(1) - Q_(2))/(Q_(1)) = (T_(1) - T_(2))/(T_(1)) According to carnot theorem "No irreversible engine can have efficiency greater than carnot reversible engine working between same hot and cold reservoirs". A carnot engine whose low temperature reservoir is at 7^(@)C has an efficiency of 50% . It is desired to increase the efficiency to 70% . By how many degrees should the temperature of the high temperature reservoir be increased?
View SolutionThe efficiency of a heat engine is defined as the ratio of the mechanical work done by the engine in one cycle to the heat absorbed from the high temperature source . eta = (W)/(Q_(1)) = (Q_(1) - Q_(2))/(Q_(1)) Cornot devised an ideal engine which is based on a reversible cycle of four operations in succession: isothermal expansion , adiabatic expansion. isothermal compression and adiabatic compression. For carnot cycle (Q_(1))/(T_(1)) = (Q_(2))/(T_(2)) . Thus eta = (Q_(1) - Q_(2))/(Q_(1)) = (T_(1) - T_(2))/(T_(1)) According to carnot theorem "No irreversible engine can have efficiency greater than carnot reversible engine working between same hot and cold reservoirs". An inventor claims to have developed an engine working between 600K and 300K capable of having an efficiency of 52% , then -
View SolutionThe efficiency of a heat engine is defined as the ratio of the mechanical work done by the engine in one cycle to the heat absorbed from the high temperature source . η=WQ1=Q1−Q2Q1 Cornot devised an ideal engine which is based on a reversible cycle of four operations in succession: isothermal expansion , adiabatic expansion. isothermal compression and adiabatic compression.
_E01_238_Q01.png)
For carnot cycle Q1T1=Q2T2. Thus η=Q1−Q2Q1=T1−T2T1 According to carnot theorem "No irreversible engine can have efficiency greater than carnot reversible engine working between same hot and cold reservoirs"._E01_238_Q02.png)
Efficiency of a carnot's cycle change from 16 to 13 when source temperature is raised by 100K. The temperature of the sink is-
View SolutionA heat engine absorbs heat Q_(1) at temperature T_(1) and heat Q_(2) at temperature T_(2) . Work done by the engine is (Q_(1)+Q_(2)) . This data
View Solutionकिसी गैस के रुद्धोष्म संपीडन में गैस को बाहर से कोई ऊष्मा नहीं दी जाती फिर भी गैस का ताप बढ़ जाता है । सम्बन्धित नियम अथवा समीकरण देते हुए इसका कारण कीजिए ।
View SolutionA heat engine absorbs heat Q1 at temperature T1 and heat Q2 at temperature T2, work done by the engine is J (Q1+Q2) this data
View SolutionThe temperature of a gas rises during an adiabatic compression, although no heat is given to the gas from outside. Why?
View SolutionA heat engine absorbs heat q1 from a source at temperature T1 and heat q2 from a source at temperature T2. Work done is found to be J(q1+q2). This is in accordance with
View Solutionएक ऊष्मा इंजन एक स्रोत से ताप T1 पर q1 ऊष्मा अवशोषित करता है तथा एक अन्य स्रोत से तापा T2 पर q2 ऊष्मा अवशोषित करता है। किया गया कार्य (q1+q2)J पाया जाता है। यह किस नियम के अनुसार होता है?
View SolutionThe temperature of a gas rises during an adiabatic compression, although no heat is given to the gas from outside. Why?
View Solution
