A thin ring of radius R is made of a wire of density ρ and Young’s modulus Y. It is spun in its own plane, about an axis through its centre, with angular velocity w. Determine the amount (assumed small) by which its circumference increases.
The correct Answer is:2πρR3ω2Y
Step by step video, text & image solution for A thin ring of radius R is made of a wire of density rho and Young’s modulus Y. It is spun in its own plane, about an axis through its centre, with angular velocity w. Determine the amount (assumed small) by which its circumference increases. by Physics experts to help you in doubts & scoring excellent marks in Class 11 exams.
Updated on:21/07/2023Topper's Solved these Questions
- ELASTICITYBook:ARIHANTChapter:ELASTICITYExercise:Level 3
Explore 1 Video
- ELASTICITYBook:ARIHANTChapter:ELASTICITYExercise:Level 3
Explore 1 Video
- CALORIMETRYBook:ARIHANTChapter:CALORIMETRYExercise:Level 3
Explore 1 Video
- FIRST LAW OF THERMODYNAMICSBook:ARIHANTChapter:FIRST LAW OF THERMODYNAMICSExercise:Level 3
Explore 6 Videos
Similar Questions
A thin ring of radius R is made of a material of density ρand Young's modulus Y. If the ring is rotated about its centre in its own plane with angular velocity omega , find the small increases in its radius.
View SolutionA thin ring of radius R is made of a material of density ρ is Young's modulus Y. If the ring is rotated about its centre in its own plane with anglular velocity ω, if the small increase in its radius is 2ρω3R3λY then find λ.
View Solution
Knowledge Check
Very thin ring of radius R is rotated about its centre. Its radius will
AincreaseBdecreaseCchange depends on materialDnone of the aboveRadius of gyration of a ring about a transverse axis passing through its centre is
A0.5× diameter of ringBdiameter of ringC2× diameter of ringD(diameter of ring)2A ring of radius R is made of a thin wire of material of density ρ, having cross-section area a and Young's modulus y. The ring rotates about an axis perpendicular to its plane and through its centre. Angular frequency of rotation is ω.
The tension in the ring will beAaρR2ω22BaρR2ω2C2aρR2ω2DaρR2ω24
A solid sphere of radius r and mass m rotates about an axis passing through its centre with angular velocity ω. Its K.E. Is
View SolutionA ring of radius R is made of a thin wire of material of density ρ, having cross-section area a and Young's modulus y. The ring rotates about an axis perpendicular to its plane and through its centre. Angular frequency of rotation is ω.
The ratio of kinetic energy to potential energy is
View SolutionThe M.I. of the solid sphere of density 'rho' and radius 'R' about an axis passing through its centre is given by
View SolutionMoment of inertia of a circular ring about an axis through its centre and perpendicular to its plane is
View SolutionA ring of radius R is made of a thin wire of material of density ρ having cross section area a. The ring rotates with angular velocity ω about an axis passing through its centre and perpendicular to the plane. If we consider a small element of the ring,it rotates in a circle. The required centripetal force is provided by the component of tensions on the element towards the centre. A small element of length dl of angular width dθ is shown in the figure.
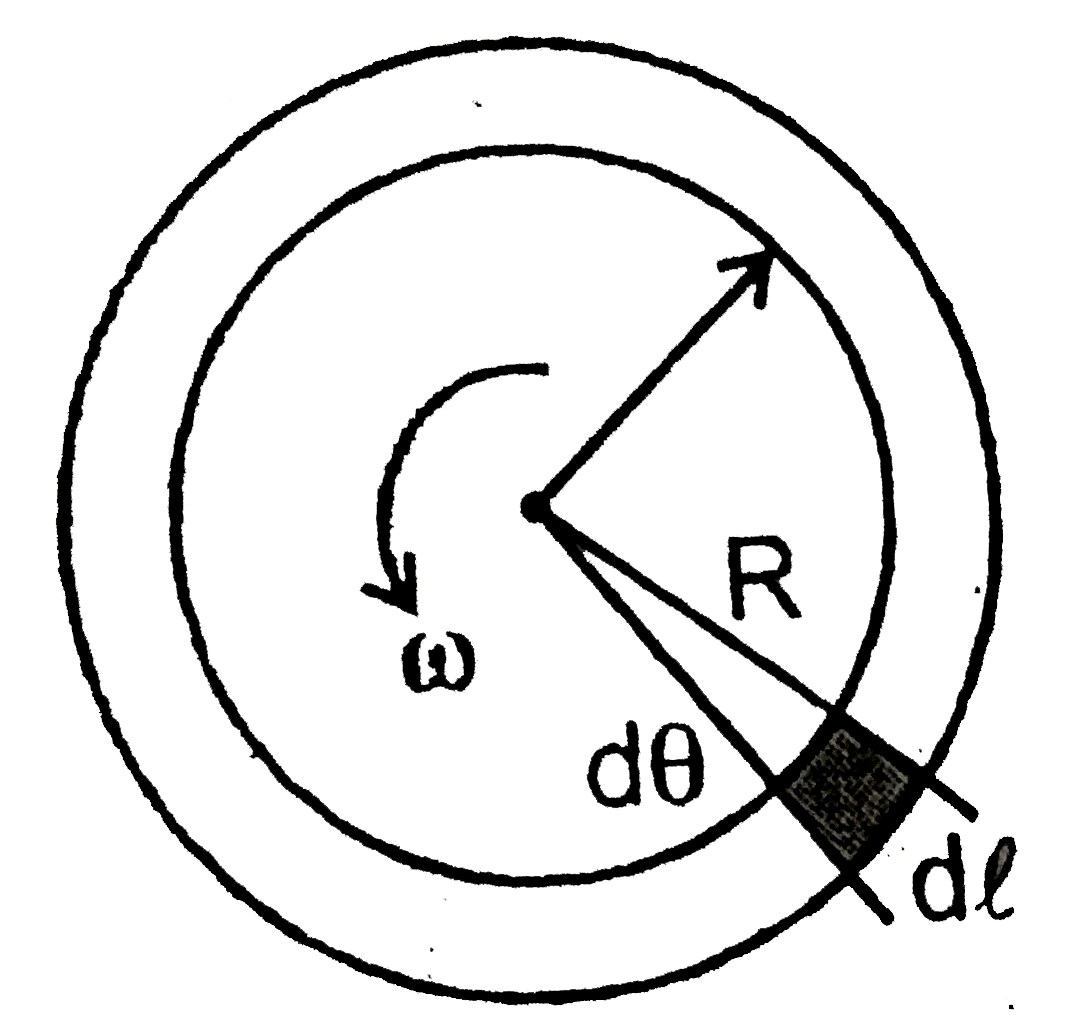
If T is the tension in the ring, then
View Solution