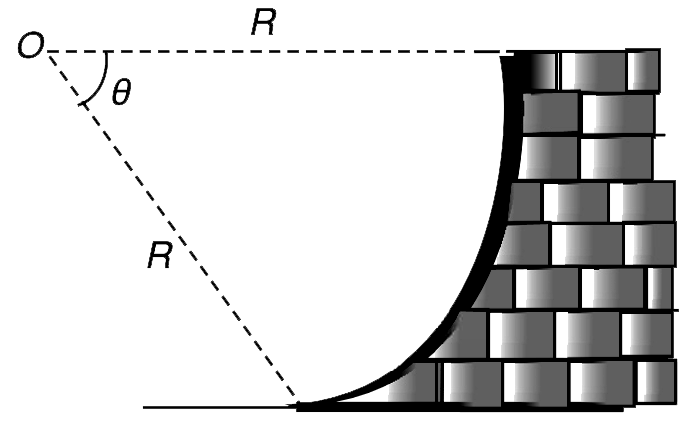
(i) In the figure shown, the heavy cylinder (radius R) resting on a smooth surface separates two liquids of densities $2 ρ$ and $3 ρ$ . Find the height 'h' for the equilibrium of cylinder.

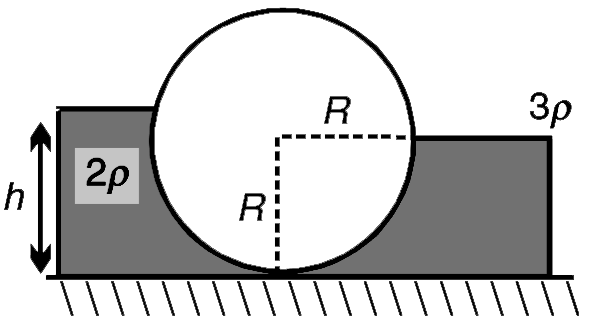
(ii) The cross section of a dam wall is an arc of a circle of radius R=20m subtending on angle of $θ = 60^{\circ}$ at the centre of the circle. the centre (O) of the circle lies in the water surface. The width of the dam (i.e., dimension perpendicular to the figure) is b=10m. Neglect atmospheric pressure is following calculations.

(a) Calculate the vertical component of force $(F_{x})$ applied by water on the curved dam wall.
(b) Calculate the horizontal component of force $(F_{H})$ applied by water on the curved dam wall.
(c) Calculate the resultant force applied by the water on the curved dam wall.

Video Solution

Text Solution

Verified by Experts

The correct Answer is:(i) $\sqrt{\frac{3}{2}} R$
(ii) (a) $F_{v} = 1.2 \times 10^{7} N$
(b) $F_{H} = 1.5 \times 10^{7} N$
(c) $F = 1.92 \times 10^{7} N$

Updated on:21/07/2023

Answer

Step by step video, text & image solution for (i) In the figure shown, the heavy cylinder (radius R) resting on a smooth surface separates two liquids of densities 2rho and 3rho. Find the height 'h' for the equilibrium of cylinder. (ii) The cross section of a dam wall is an arc of a circle of radius R=20m subtending on angle of theta=60^(@) at the centre of the circle. the centre (O) of the circle lies in the water surface. The width of the dam (i.e., dimension perpendicular to the figure) is b=10m. Neglect atmospheric pressure is following calculations. (a) Calculate the vertical component of force (F_(x)) applied by water on the curved dam wall. (b) Calculate the horizontal component of force (F_(H)) applied by water on the curved dam wall. (c) Calculate the resultant force applied by the water on the curved dam wall. by Physics experts to help you in doubts & scoring excellent marks in Class 11 exams.

Updated on:21/07/2023

Class 11 PHYSICS FLUID MECHANICS

Topper's Solved these Questions

Knowledge Check

In the figure shown, the heavy cylinder (radius R) reasting on a smooth surface separates two liquids of densities $2 ρ$ and $3 ρ$ . The height h for the equilibrium of cylinder must be

A $3 R / 2$
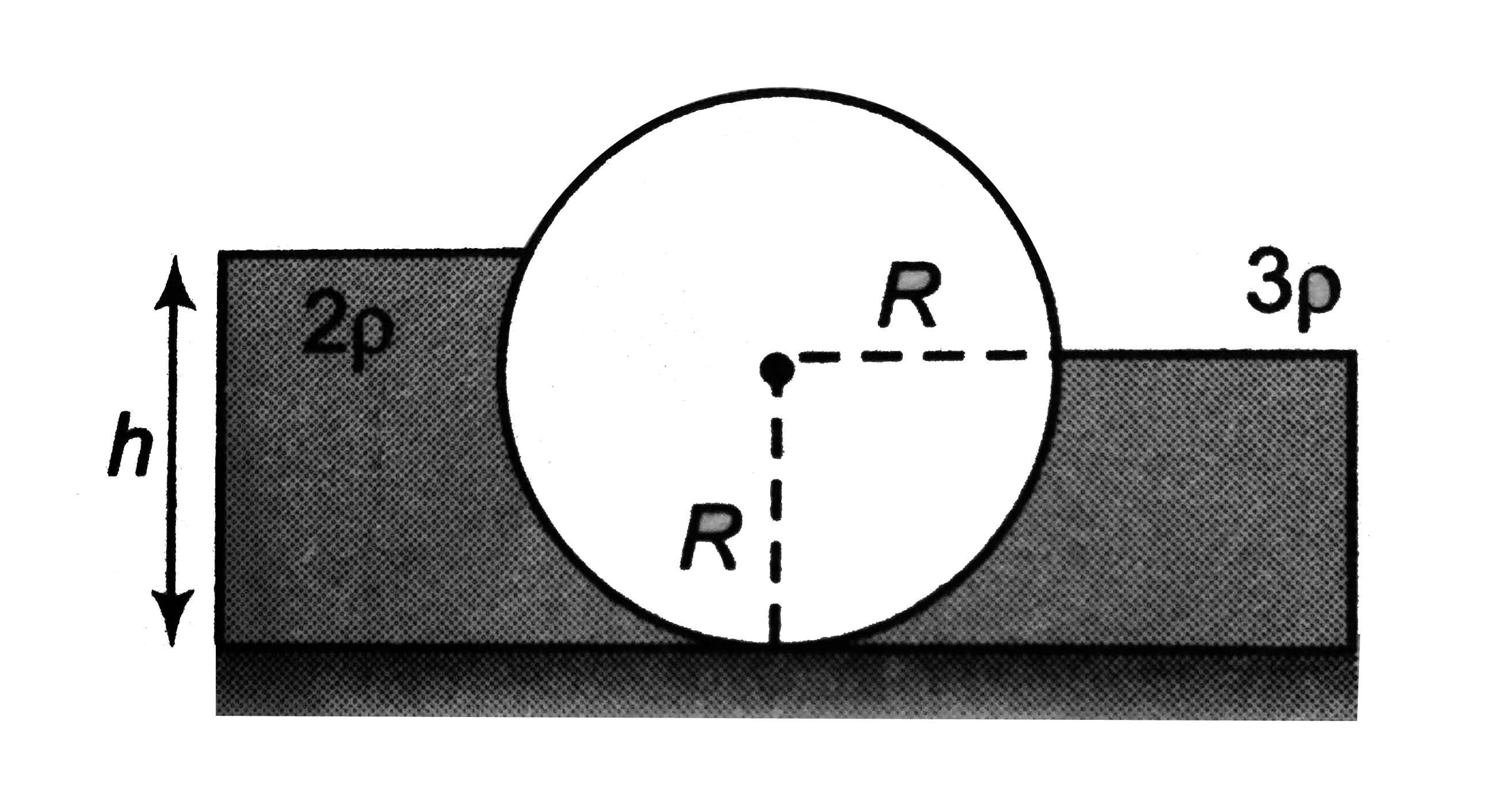B $R \sqrt{\frac{3}{2}}$
C $R \sqrt{2}$
DNone of these
In a circle of radius 21 cm, an arc subtends an angle of $60^{\circ}$ at the centre. The length of the arc is
A21 cm
B22 cm
C18.16 cm
D23.5cm
In a circle of radius r cm, an arc subtends an angle of $60^{\circ}$ at the centre. The length of the arc will be $:$
A $\frac{π r}{3} c m$
B $\frac{2 π r}{3} c m^{2}$
C $\frac{π r^{2}}{3} c m^{2}$
D $\frac{π r}{3} c m^{2}$

ARIHANT-FLUIDS-All Questions

Home
Ask Doubt
Profile

The correct Answer is:(i) √32R (ii) (a) Fv=1.2×107N (b) FH=1.5×107N (c) F=1.92×107N

Topper's Solved these Questions

Similar Questions

Knowledge Check

In the figure shown, the heavy cylinder (radius R) reasting on a smooth surface separates two liquids of densities 2ρ and 3ρ. The height h for the equilibrium of cylinder must be

In a circle of radius 21 cm, an arc subtends an angle of 60∘ at the centre. The length of the arc is

In a circle of radius r cm, an arc subtends an angle of 60∘ at the centre. The length of the arc will be :

ARIHANT-FLUIDS-All Questions

The correct Answer is:(i) $\sqrt{\frac{3}{2}} R$
(ii) (a) $F_{v} = 1.2 \times 10^{7} N$
(b) $F_{H} = 1.5 \times 10^{7} N$
(c) $F = 1.92 \times 10^{7} N$

In the figure shown, the heavy cylinder (radius R) reasting on a smooth surface separates two liquids of densities $2 ρ$ and $3 ρ$ . The height h for the equilibrium of cylinder must be

In a circle of radius 21 cm, an arc subtends an angle of $60^{\circ}$ at the centre. The length of the arc is

In a circle of radius r cm, an arc subtends an angle of $60^{\circ}$ at the centre. The length of the arc will be $:$