A semicircular ring of radius R is fixed on a smooth horizontal table. A small block is projected with speed u so as to enter the ring at end A. Initial velocity of the block is along tangent to the ring at A and it moves on the table remaining in contact with the inner wall of the ring. The coefficient of friction between the block and the ring is .
(a) Find the time after which the block will exit the ring at B.
(b) With what speed will the block leave the ring at B.
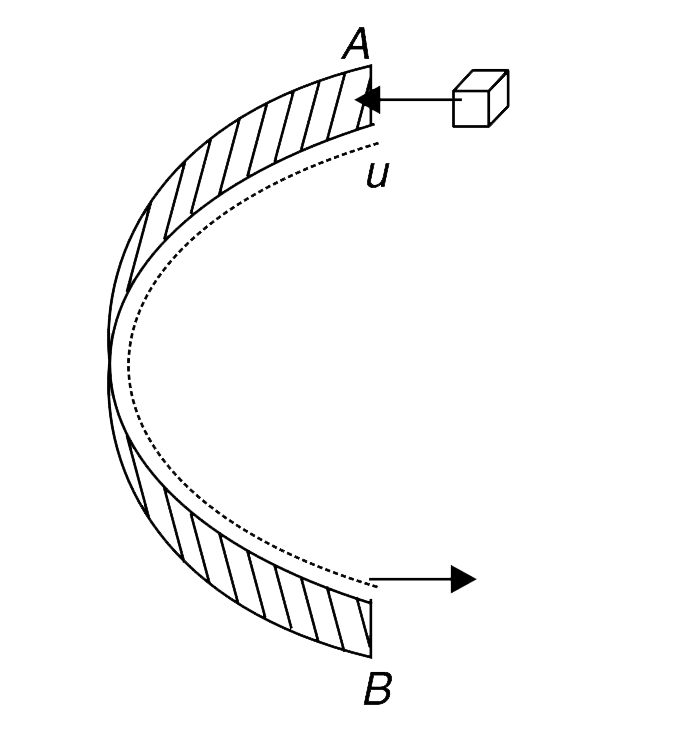
The correct Answer is:(a)
(b)
Step by step video, text & image solution for A semicircular ring of radius R is fixed on a smooth horizontal table. A small block is projected with speed u so as to enter the ring at end A. Initial velocity of the block is along tangent to the ring at A and it moves on the table remaining in contact with the inner wall of the ring. The coefficient of friction between the block and the ring is mu. (a) Find the time after which the block will exit the ring at B. (b) With what speed will the block leave the ring at B. by Physics experts to help you in doubts & scoring excellent marks in Class 11 exams.
Updated on:21/07/2023Topper's Solved these Questions
Similar Questions
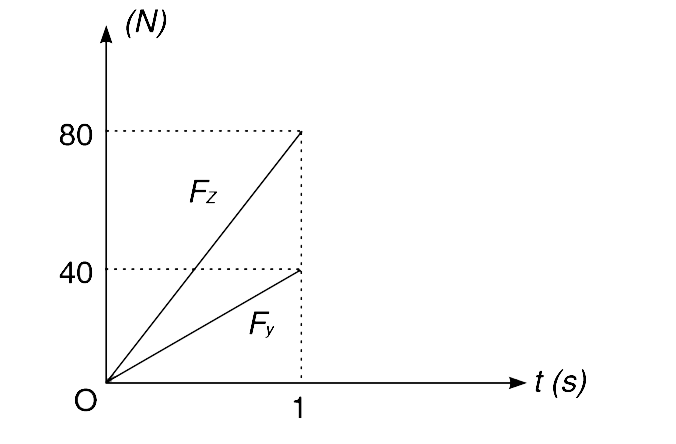
A particle is acted upon by a force for 1 second whose X component remains constant at Fx=30N but y and z components vary with time as shown in the graph. Calculate the magnitude of change in momentum of the particle in 1 s. What angle does the change in momentum (Δ→P) make with X axis ?
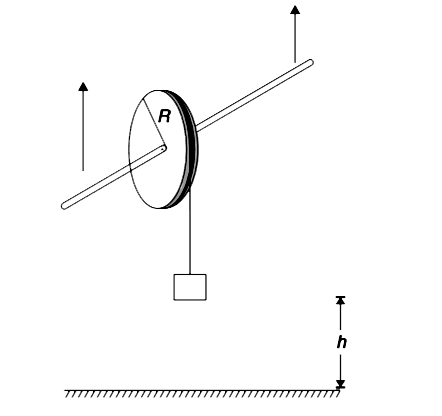
View SolutionThe pulley of radius R can rotate freely about its axle as shown in the figure. A thread is tightly wrapped around the pulley and its free end carries a block of mass m. When the block is at a height h above the ground the system is released (i.e., the pulley is made free to rotate & the block is allowed to fall) and at the same instant the axle is moved up keeping it horizontal all the time. When the block hits the floor the axle has gone up by a distance 2h. Find the angle by which the pulley must have rotated by this time.
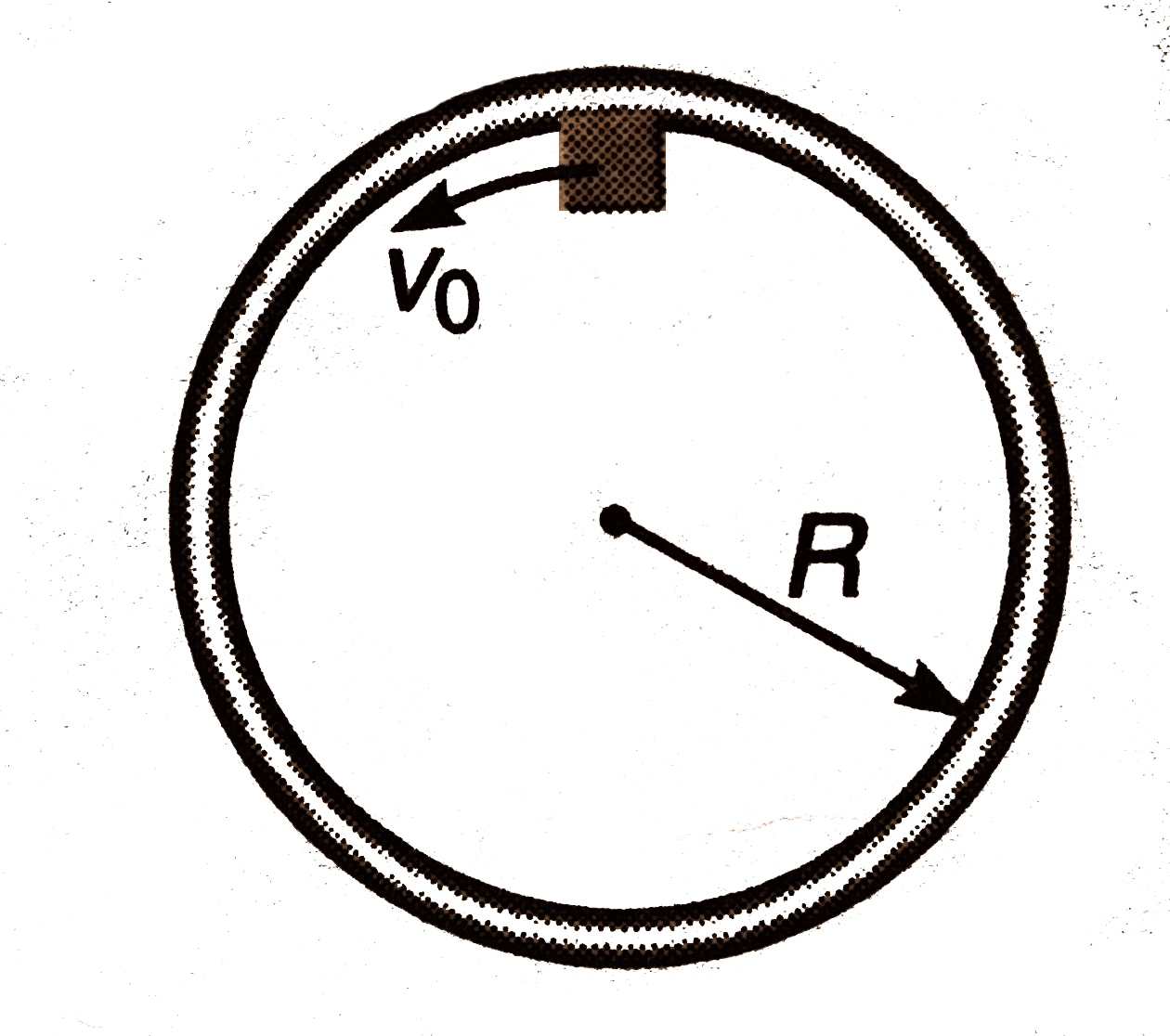
View SolutionA block of mass m slides 0n a frictionless table. It is constrained to move inside a ring of radius R . At time t=0 , block is moving along the inside of the ring (i.e. in the tangential direction) with velocity v0 . The coefficient of friction between the block and the ring is μ . Find the speed of the block at time t .
View SolutionThe smooth ring A can slide on a fixed horizontal rod as shown the pulley is fixed. If some instant velocity of ring is v, find the velocity of block at that instant.
View SolutionFour identical spheres having mass M and radius R are fixed tightly within a massless ring such that the centres of all spheres lie in the plane of ring. The ring is kept on a rough horizontal table as shown. The string is wrapped around the ring can roll without slipping. The other end of the string is passed over a massless frictionless pulley to a block of mass M. A force F is applied horizontally on the ring, at the same level as the centre, so that the system is in equilibrium. The moment of inertia of the combined ring system about the centre of ring will be
View SolutionA ring of mass M and radius R lies flat on a horizontal table. A light thread is wound around it and its free end is pulled with a constant velocity v . (a) Two small segment A and B (see fig.) in the ring are rough and have a coefficient of friction mu with the table. Rest of the ring is smooth. Find the speed with which the ring moves. (b) Find the speed of the ring if coefficient of friction is mu everywhere, for all points on the ring.
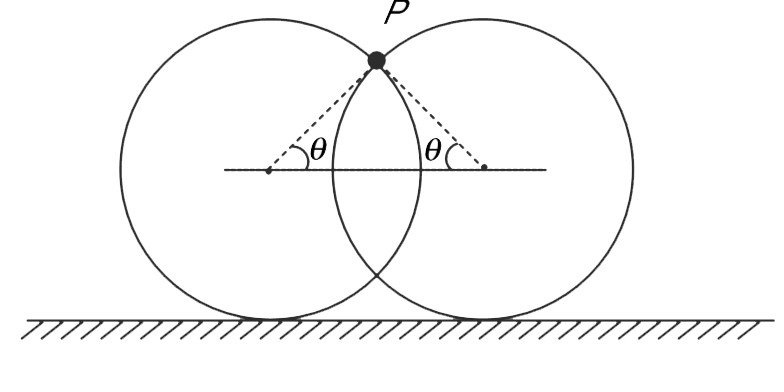
View SolutionTwo identical rings, each of mass M and radius R, are standing on a rough horizontal surface. The rings overlap such that the horizontal line passing through their centre makes an angle of θ=45∘ with the radius through their intersection point P. A small object of mass m is placed symmetrically on the rings at point P and released. Calculate the acceleration of the centre of the ring immediately after the release. There is no friction between the small object and the rings. The friction between the small object and the rings, and the friction between the rings and the ground is large enough to prevent slipping.
View SolutionA smooth ring of mass m can slide on a fixed horizontal rod. A string tied to the ring pases over a fixed pulley B and carries a block C of mass 2m as shown below. As the ring starts sliding
View SolutionThe figure shows mass m moves with velocity u. Find the velocity of ring at that moment. Ring is restricted to move on smooth rod.
View SolutionConsider two identical rings of mass M and radius R fixed on a horizontal axis in a gravity - free space. A particle of mass m is held at rest at the centre of 1^("st") ring and projected along the axis towards 2^("nd") ring. The minimum speed of projection so that the particle reaches the centre of 2^("nd") ring is
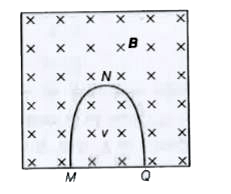
View Solutionएक अर्द्धवृत्ताकार वलय जिसकी त्रिज्या R है अपने तल के लम्बवत क्षैतिज चुम्बकीय क्षेत्र B में गिर रही है। MNQ स्थिति पर वलय की चाल है तथा वलय में विभवान्तर है
View Solutionω0কৌণিক বেগে ঘূর্ণায়মান m ভরের এবং r ব্যাসার্ধের একটি রিং অনুভূমিক অমৃসণ তলের ওপর রাখা হল । রিংটির কেন্দ্রের প্রাথমিক বেগ শূন্য । তলের ওপর রিংটির পিছলানো গতি বন্ধ হওয়ার মুহূর্তে রিংটির কেন্দ্রের বেগ কত হবে ?
View Solutionत्रिज्या R की एक पतली अर्द्धवृत्ताकार धात्विक वलय क्षैतिज चुम्बकीय प्रेरण B में इस प्रकार गिर रही है कि इसका तल ऊर्ध्वाधर रहता है। जब यह वलय MNQ की स्थिति में पहुँचती है तब इसका वेग । है। इस समय अर्द्धवृत्ताकार वलय में उत्पन्न विभवान्तर होगा

View SolutionA metal ring of mass m and radius R is placed on a smooth horizontal table and is set rotating about axis, such that ring moves with constant speed. The tension in the ring is mv2nπR Then the value of n is
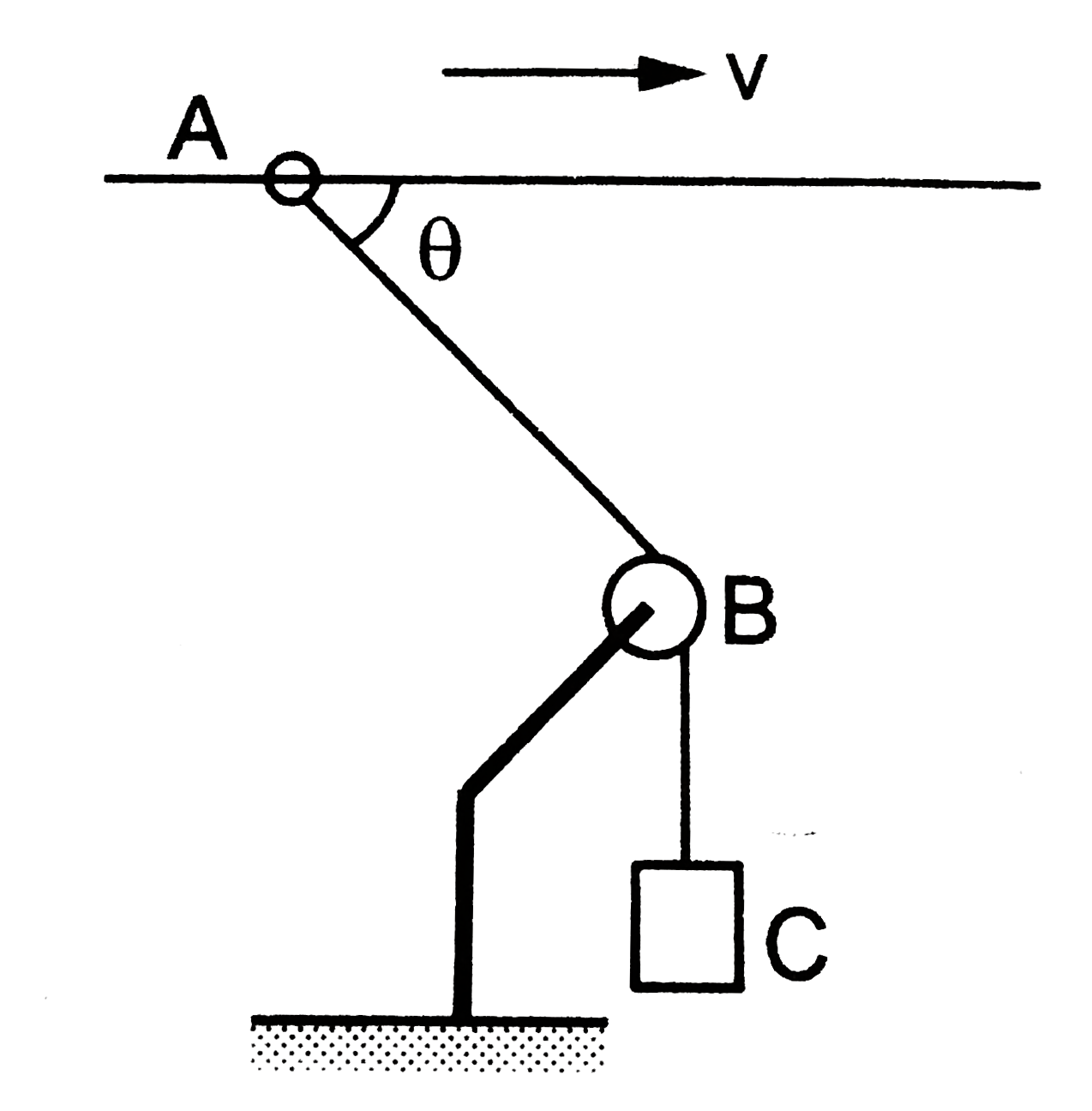
View SolutionA smooth ring A of mass m can slide on a fixed horizontal rod. A string tied to the ring passes over a fixed pulley B and carries a block C of mass M(=2m) as shown in figure. At an instant the string between the ring and the pulley makes an angle θ with the rod. a. Show that, if the ring slides with a speed v, the block descends with speed vcosθ, b. With what acceleration will the ring starts moving if the system is released from rest with θ=300
View Solution
