Two balls of equal mass have a head-on collision with speed 6m/s. If the coefficient of restitution is 13, find the speed of each ball after impact in m/s
The correct Answer is:2m/s
To solve the problem, we need to determine the speeds of two balls after a head-on collision, given that they have equal masses and a coefficient of restitution of 13. Let's break down the solution step by step.
1. Identify Initial Conditions:
- Let the mass of each ball be m.
- The initial speed of ball A (moving towards ball B) is u1=6m/s.
- The initial speed of ball B (moving towards ball A) is u2=−6m/s (negative because it's in the opposite direction).
2. Coefficient of Restitution:
- The coefficient of restitution e is given as 13.
- The formula for the coefficient of restitution is:
e=Relative speed after collisionRelative speed before collision
- This can be expressed as:
e=v2−v1u1−u2
where v1 and v2 are the final velocities of balls A and B, respectively.
3. Calculate Relative Speeds:
- The relative speed before the collision:
u1−u2=6−(−6)=6+6=12m/s
- Substitute this into the equation for e:
13=v2−v112
4. Express the Equation:
- Rearranging gives:
v2−v1=123=4(1)
5. Conservation of Momentum:
- Since the masses are equal, we can use the conservation of momentum:
mu1+mu2=mv1+mv2
- Simplifying (dividing by m):
6+(−6)=v1+v2
- This simplifies to:
0=v1+v2(2)
6. Solve the Equations:
- From equation (2), we can express v2 in terms of v1:
v2=−v1
- Substitute v2=−v1 into equation (1):
−v1−v1=4
−2v1=4⟹v1=−2m/s
- Now substitute back to find v2:
v2=−(−2)=2m/s
7. Final Speeds:
- The final speeds of the balls after the collision are:
- Ball A (v1): −2m/s (moving in the opposite direction)
- Ball B (v2): 2m/s
Conclusion:
The speed of ball A after impact is 2m/s in the opposite direction, and the speed of ball B after impact is 2m/s.
- Let the mass of each ball be m.
- The initial speed of ball A (moving towards ball B) is u1=6m/s.
- The initial speed of ball B (moving towards ball A) is u2=−6m/s (negative because it's in the opposite direction).
- The coefficient of restitution e is given as 13.
- The formula for the coefficient of restitution is:
e=Relative speed after collisionRelative speed before collision
- This can be expressed as:
e=v2−v1u1−u2
where v1 and v2 are the final velocities of balls A and B, respectively.
- The relative speed before the collision:
u1−u2=6−(−6)=6+6=12m/s
- Substitute this into the equation for e:
13=v2−v112
- Rearranging gives:
v2−v1=123=4(1)
- Since the masses are equal, we can use the conservation of momentum:
mu1+mu2=mv1+mv2
- Simplifying (dividing by m):
6+(−6)=v1+v2
- This simplifies to:
0=v1+v2(2)
- From equation (2), we can express v2 in terms of v1:
v2=−v1
- Substitute v2=−v1 into equation (1):
−v1−v1=4
−2v1=4⟹v1=−2m/s
- Now substitute back to find v2:
v2=−(−2)=2m/s
- The final speeds of the balls after the collision are:
- Ball A (v1): −2m/s (moving in the opposite direction)
- Ball B (v2): 2m/s
The speed of ball A after impact is 2m/s in the opposite direction, and the speed of ball B after impact is 2m/s.
Topper's Solved these Questions
- CENTRE OF MASSBOOK - ALLENCHAPTER - CENTRE OF MASSEXERCISE - EXERCISE-I
40 Videos
- CENTRE OF MASSBOOK - ALLENCHAPTER - CENTRE OF MASSEXERCISE - EXERCISE-II
43 Videos
- BASIC MATHEMATICS USED IN PHYSICS &VECTORS BOOK - ALLENCHAPTER - BASIC MATHEMATICS USED IN PHYSICS &VECTORS EXERCISE - EXERCISE-IV ASSERTION & REASON
11 Videos
- ELASTICITY, SURFACE TENSION AND FLUID MECHANICSBOOK - ALLENCHAPTER - ELASTICITY, SURFACE TENSION AND FLUID MECHANICSEXERCISE - Exercise 5 B (Integer Type Questions)
3 Videos
Similar Questions
A ball of mass 2kg moving with speed 5m/s collides directly with another ball of mass 3kg moving in the same direction with speed 4m/s. The coefficient os restitution is 2/3. Find the velocities of both ball after the collision.
View SolutionA ball of mass 2m moving due east with a speed of 8u collides directly with a ball B of mass m and the velocity of B after collision is 5 u due east. If the coefficient of restitution is 1/11, find the fraction of the total kinetic energy lost?
View Solution
Knowledge Check
Two balls of equal mass have a head on collisino with speed 4ms−1 each travelling in opposite direction. If the coefficient's of resolution is 12, the speed of each ball after impact will be
A1ms−1B2ms−1C3ms−1Ddata insufficient.Two balls of masses 2m and m are moving with speed 2v0 and v0 towards each other. If the coefficient of restitution e=1/3 , the speed of balls , if collision is head - on
Av03,4v03B2v03,5v03C2v03,4v03Dv03,2v03A 1 kg ball moving with a speed of 6 ms−1 collides head - on with a 0.5 kg ball moving in the opposite direction with a speed of 9 ms−1. If the coefficient of restitution is 13, the energy lost in the collision is
A303.4 JB66.7 JC33.3 JD67.8 J
A ball of a mass m hits the floor with as speed v making an angle of incidence θ with the normal. The coefficient of restitution is e. Find the speed of the reflected ball and the angle of reflection of the ball.
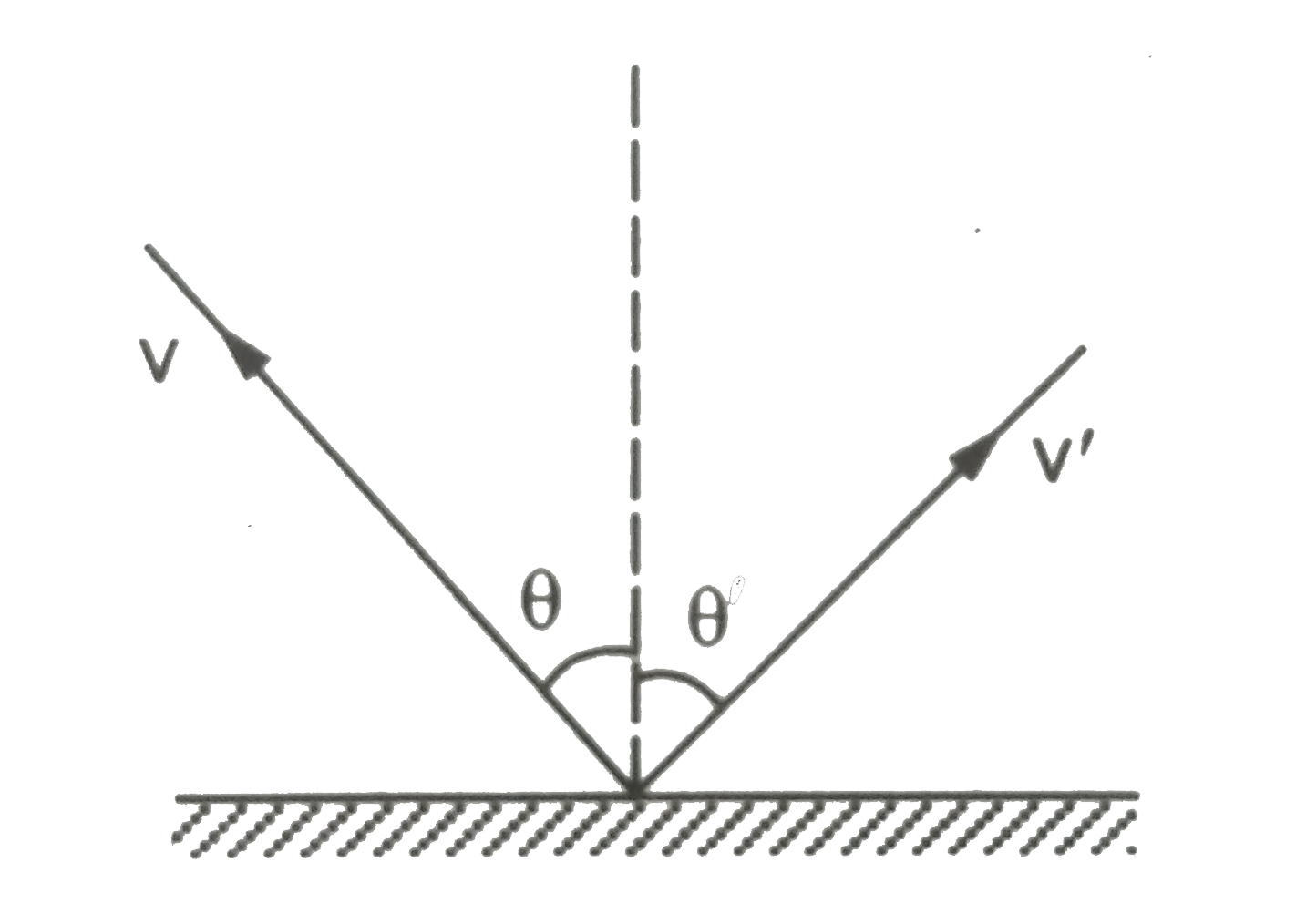
View SolutionA ball of mass m hits a floor with a speed v0 making an angle of incidence α with the normal. The coefficient of restitution is e. Find the speed of the reflected ball and the angle of reflection of the ball.
View SolutionA ball of mass m hits the floor with a speed v0 making an angle of incidence α with the normal. The coefficient of restitution is e. find the speed of the reflected ball and the angle of reflection of the ball.
View SolutionA ball of mass m moving with velocity v collides head-on which the second ball of mass m at rest. I the coefficient of restitution is e and velocity of first ball after collision is v1 and velocity of second ball after collision is v2 then
View SolutionA 2kg ball , moving at 10m/s , collides head - on with a 3kg ball moving in the opposite direction at 20m/s. If the coefficient of restitution is 1/3 , then the energy lost in the collision is
View Solution
