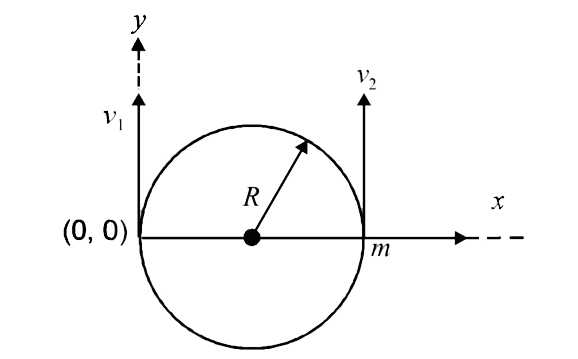
A particle of mass m, moving in a cicular path of radius R with a constant speed is located at point (2R,0) at time and a man starts moving with a velocity along the +ve y-axis from origin at time . Calculate the linear momentum of the particle w.r.t. the man as a function of time.
The correct Answer is:
and
where
Topper's Solved these Questions
- CENTRE OF MASSBook:ALLENChapter:CENTRE OF MASSExercise:EXERCISE-V A
Explore 20 Videos
- BASIC MATHEMATICS USED IN PHYSICS &VECTORS Book:ALLENChapter:BASIC MATHEMATICS USED IN PHYSICS &VECTORS Exercise:EXERCISE-IV ASSERTION & REASON
Explore 11 Videos
- ELASTICITY, SURFACE TENSION AND FLUID MECHANICSBook:ALLENChapter:ELASTICITY, SURFACE TENSION AND FLUID MECHANICSExercise:Exercise 5 B (Integer Type Questions)
Explore 3 Videos
Similar Questions
A particle of mass m is moving anticlockwise, in a circle of radius R in x−y plane with centre at (R,0) with a constant speed v2. If is located at point (2R,0) at time t=0. A man starts moving with a velocity v1 along the positive y-axis from origin at t=0. Calculate the linear momentum of the particle w.r.t. man as a function of time.
View SolutionA particle of mass 'm' is moving on a circular path of radius 'r' with uniform speed 'v'. Rate of change of linear momentum is
View Solution
Knowledge Check
A particle of mass m is moving on a circular path of radius r with uniform speed v , rate of change of linear momentum is
AZeroBIndependent of speedCProportional to radius rDProportional to v2A particle of mass m is moving along a circle of radius r with a time period T. Its angular momentum is
A2πmrTB4πmrTC2πmr2TD4πmr2TA particle moves along a semicircular path of radius R in time t with constant speed. For the particle calculate
Adistance travelledBdisplacementCaverage speedDaverage velocity
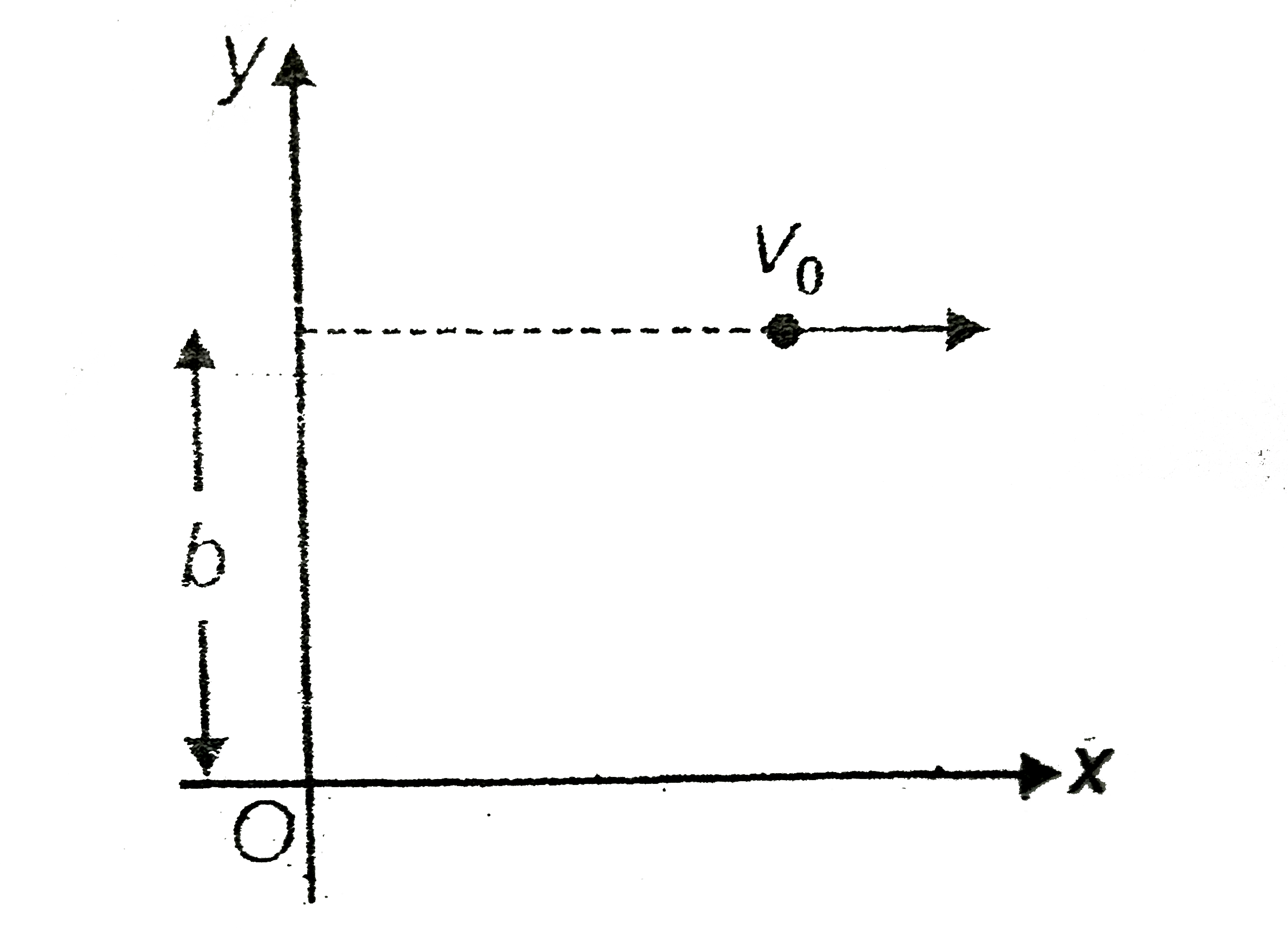
A particle of mass m is moving with constant velocity v0 along the line y=b. At time t=0 it was at the point (0,b). At time t=bv0, the
View SolutionA particle is moving in a circle of radius R with constant speed. The time period of the particle is T. In a time t=T6
Average speed of the particle is ……
View SolutionA particle moves along a circle of radius R with a constant angular speed ω. Its displacement (only magnitude) in time t will be
View SolutionA particle moves along a circle of radius R with a constant angular speed ω . Its displacement (only magnitude) in time t will be
View SolutionA particle is moving in a circle of radius R with constant speed. The time period of the particle is T. In a time t=T6
Average velocity of the particle is…..
View Solution