Question

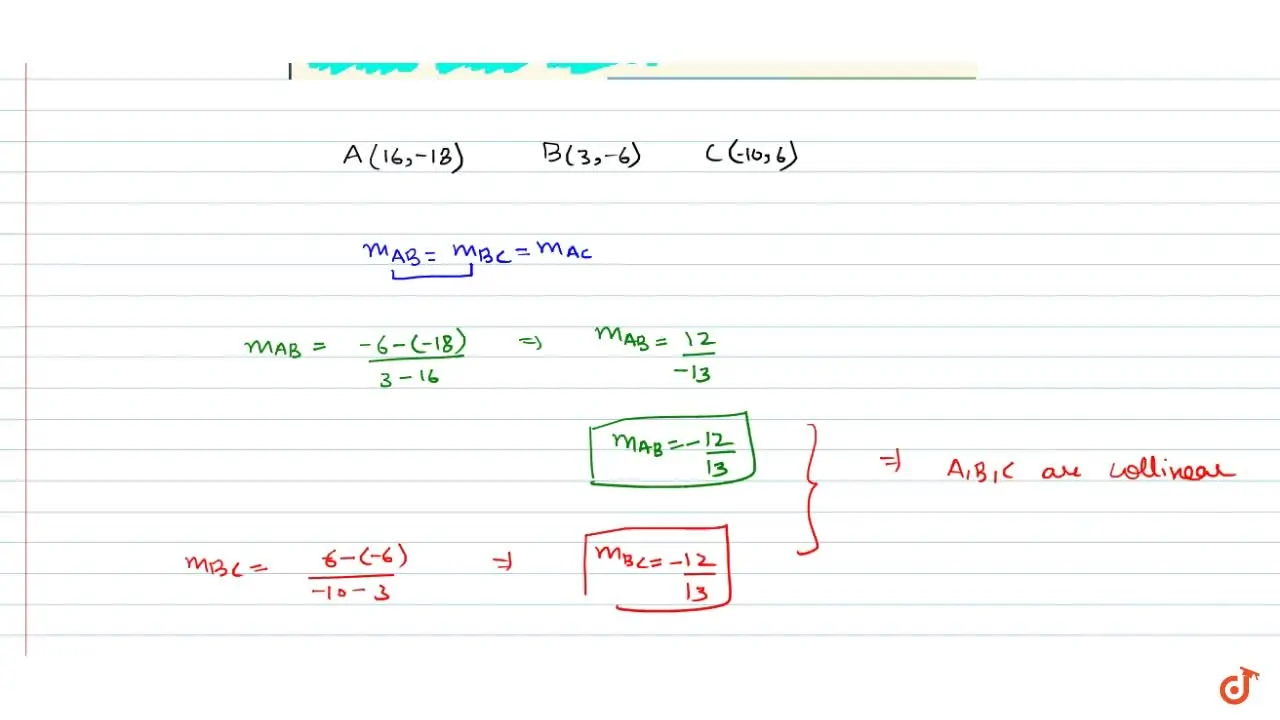
Using the method of slope, show that the following points are
collinear: $A(16,-18),B(3,-6),C(-10,6)$

Explanation

` A(16,-18), B(3,-6), C(-10,6)`
Slope of `A B=frac{y_{2}-y_{1}}{x_{2}-x_{1}}=frac{-6+18}{3-16}=-frac{12}{13}`
Slope of `B C=frac{y_{2}-y_{1}}{x_{2}-x_{1}}=frac{6+6}{-10-3}=-frac{12}{13}`

Since, Slope of `A B`= Slope of `B C=-frac{12}{13}`
Therefore,

The given points are collinear.