The slope of a line is double of the slope of another line. If tangent of the angle between them is 13, find the slopes of the lines.
Video Solution
Text Solution
Let m1 and m be the slopes of two given line such thatm1=2m.
We know that if theta is the angle between the lines l1 andl2 with slopes m1 and m2,
then tanθ=∣∣∣m2−m11+m1⋅m2∣∣∣
It is given that the tangent of the angle between the two lines is13
therefore 13=∣∣∣m−2⋅m1+(2⋅m)m∣∣∣
13=∣∣∣−m1+2⋅m2∣∣∣
13=−m1+2~m2 or 13=−{−m1+2~m2}=m1+2~m2
2m2+3m+1=0or 2m2−3m+1=0
m=−1,−12text or m=1,12
Correspondingm1=−2,−1,2,1
|
Topper's Solved these Questions
Similar Questions
Knowledge Check
The slope of a line is 3 times the slope of the other line and the tangent of the angle between them is 4/13, sum of their slopes is equal to
A2B4C6D8The slope of the given line is ,
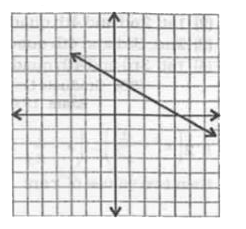 APositiveBNegativeCUndefinedDZero
APositiveBNegativeCUndefinedDZeroSlope of a budget line is
AIncreaseing throghoutBDecreasing through outCConstant throughoutDFluctuating through out