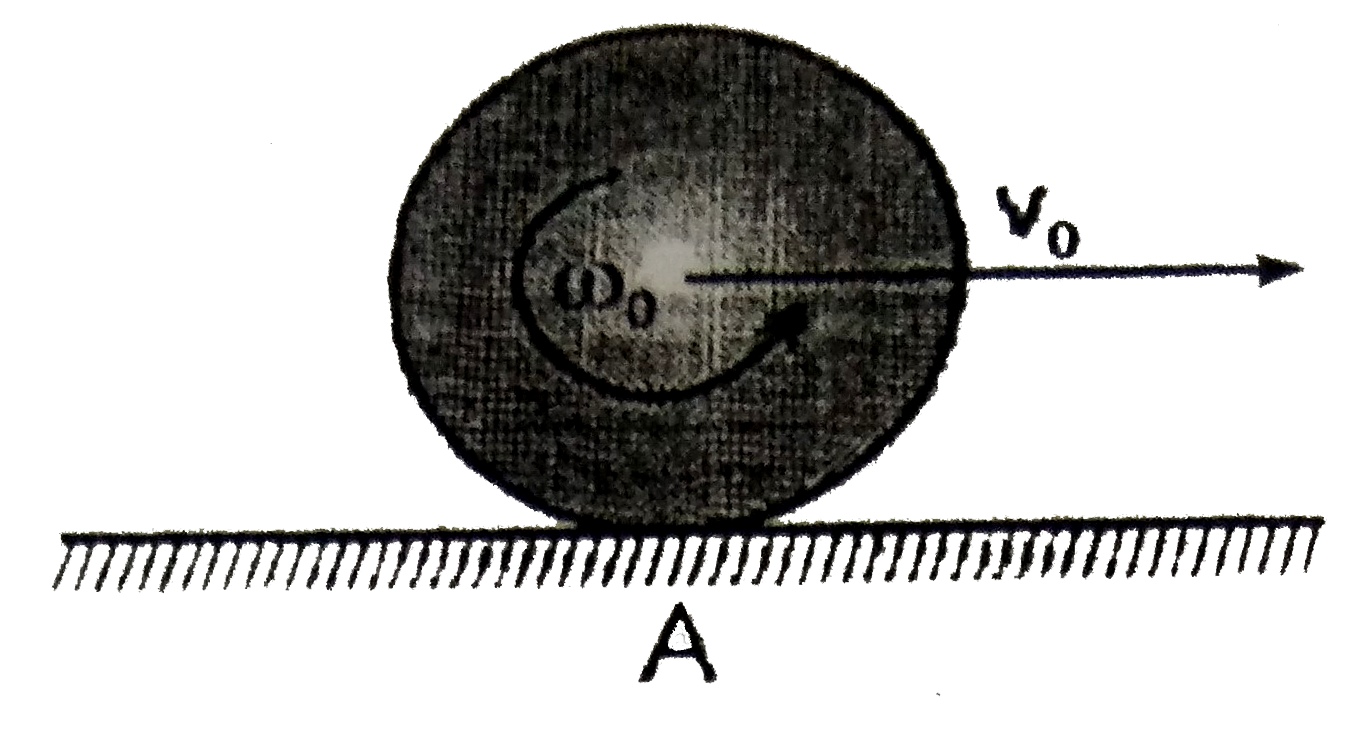
A solid sphere of mass $m$ and radius $r$ is given an initial angular velocity $ω_{0}$ and a linear velocity $v_{0} = λ r ω_{0}$ from a point $A$ on a rough horizontal surface .It is observed that the ball turns back and returens to the point $A$ after some time if $λ$ is less than a certain maximum value $λ_{0}$ . Find $λ_{0}$ .

Video Solution

Text Solution

Verified by Experts

The correct Answer is: $λ_{0} = 2 / 5$

Ball will comes back to the initial position if its angular velocity is greater than zero is the same direction (in which it was released) at the moment its's linear velocity becomes zero. In his condition ball would returen back.

$0 = v_{0} + (μ g) t$
$t = \frac{v_{0}}{μ g}$ (time when ball stop)
For rotation motion

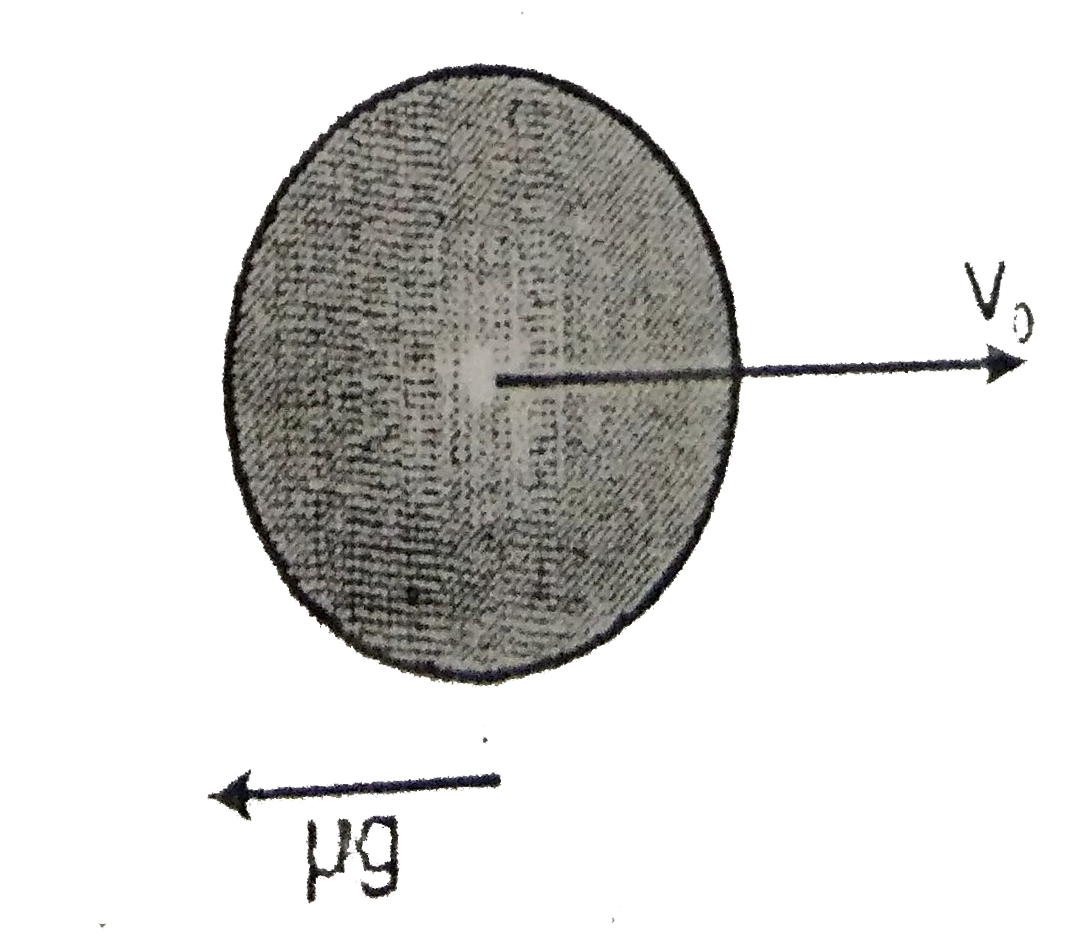
$τ = I α$
$\Rightarrow α = \frac{τ}{I} = μ g m \times R ¯ ¯¯¯¯¯¯¯¯¯¯ ¯ \frac{2}{5} M R^{2} = \frac{5 μ g}{2 R^{2}}$
using $ω_{f} = ω_{0} - α t$
$ω_{f} > 0$
$\Rightarrow \frac{v_{0}}{α R} > \frac{5 μ g}{2 R} . \frac{v_{0}}{μ g}$ for limiting condition
$\Rightarrow λ_{0} = \frac{2}{5}$

|

Updated on:21/07/2023

Class 11 PHYSICS QUESTION BANK

Topper's Solved these Questions

Similar Questions

Knowledge Check

Question 1 - Select One
A disc is given an angular velocity $ω_{0}$ and a linear velocity $v_{0}$ as shown in the figure. It is released on a rough horizontal surface of friction coefficient $μ .$ Mark the correct statement $(ω_{0} = 3 v_{0} / R)$
AThe frictional force will be $μ$ mg during the entire motion.
BAfter some time the disc will start rolling without sliding along positive x-axis.
CAfter some time the disc will start rolling without sliding along negative x-axis.
DThe mechnical energy of the disc will remain conserved.
Question 2 - Select One or More
A disc is given an initial angular velocity $ω_{0}$ and placed on a rough horizontal surface as shown Fig. The quantities which will not depend on the coefficient of friction is/are

Athe time until rolling begins
Bthe displacement of the disc until rolling begins
Cthe velocity when rolling begins
Dthe work done by the force of friction
Question 3 - Select One
A solid sphere of mass $m$ and radius $R$ rolls without slipping on a horizontal surface such that $v_{c . m .} = v_{0}$ .
AThe kinetic energy of rotation is $\frac{1}{5} m v_{0}^{2}$ .
BThe total kinetic energy is $\frac{7}{10} m v_{0}^{2}$ .
CThe mechanical energy (assume the ground as reference) is $m g R + \frac{7}{10} m v_{0}^{2}$ .
DAll options are correct.

RESONANCE-DAILY PRACTICE PROBLEMS-dpp 66

Home
Ask Doubt
Profile