There are four arrangements of a solid cylinder and a plank as shown in the figures. Some surfaces are smooth and some are rough as indicated . There is no slipping at each rough surface. The plank and / or centre of cylinder are given a horizontal constant velocity as shown in each of the situations. Usinn this information fill in the blanks.
![There are four arrangements of a solid cylinder and a plank as shown in the figures. Some surfaces are smooth and some are rough as indicated . There is no slipping at each rough surface. The plank and // or centre of cylinder are given a horizontal constant velocity as shown in each of the situations. Usinn this information fill in the blanks. The speed of center of mass of the cylinder is .......................... (ii) , The angular velocity of the cylinder is ........................ (iii),(RESPHYDPP04XIE01537Q03.png]() The speed of center of mass of the cylindr is ............... . (iv), The angular velocity of the cylinder is ............ . (a) V (b) V//R (c) 2V//R (d) 4V//R (e) cannot be determined from the given information (f) Zero." loading="eager" src="https://d10lpgp6xz60nq.cloudfront.net/physics_images/RES_PHY_DPP_67_XI_E01_537_Q01.png" width="80%" class="mx-auto" style="background:white;"> The speed of center of mass of the cylinder is ..........................
The speed of center of mass of the cylindr is ............... . (iv), The angular velocity of the cylinder is ............ . (a) V (b) V//R (c) 2V//R (d) 4V//R (e) cannot be determined from the given information (f) Zero." loading="eager" src="https://d10lpgp6xz60nq.cloudfront.net/physics_images/RES_PHY_DPP_67_XI_E01_537_Q01.png" width="80%" class="mx-auto" style="background:white;"> The speed of center of mass of the cylinder is ..........................
(ii) , The angular velocity of the cylinder is ........................
The angular velocity of the cylinder is ........................
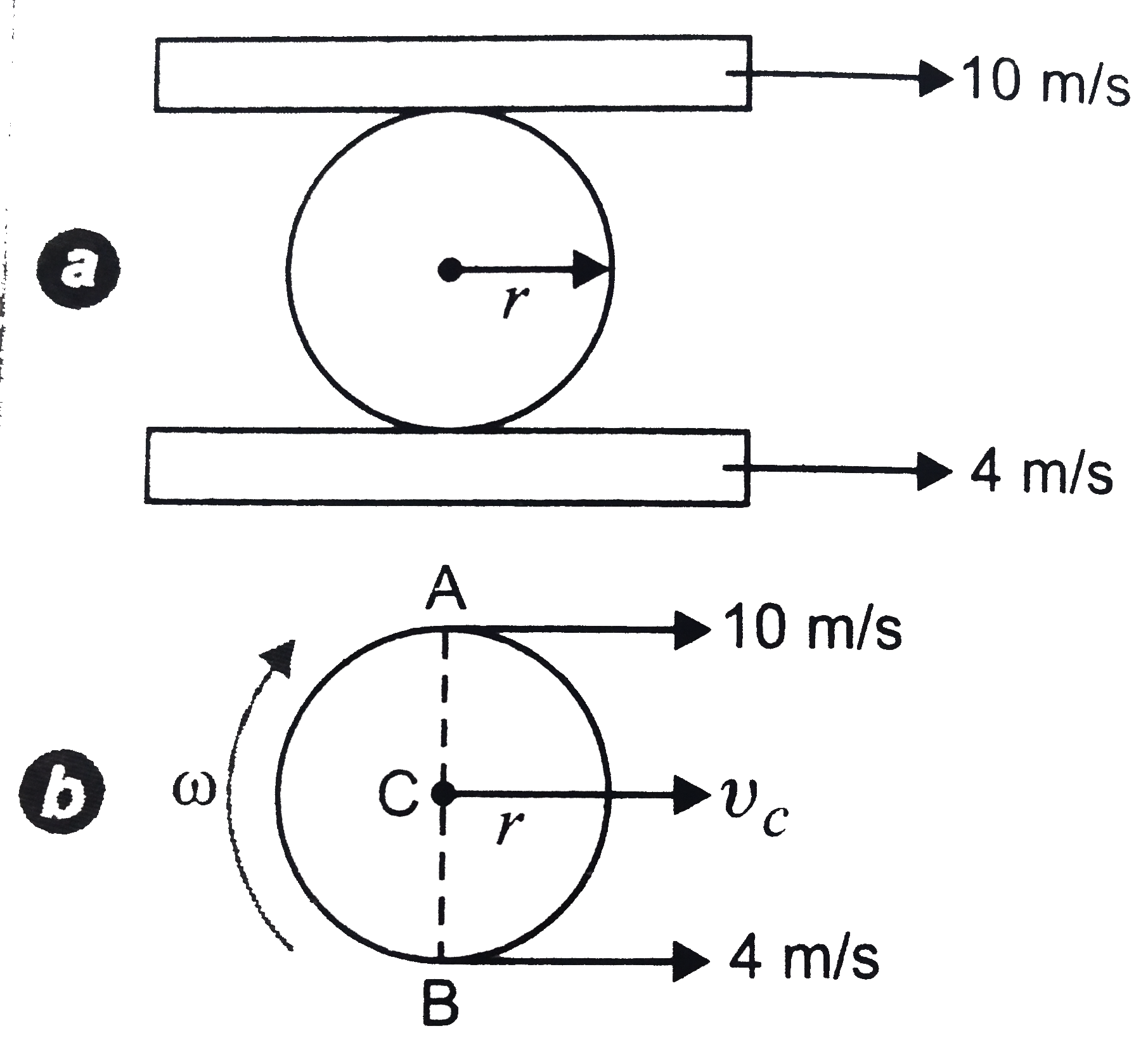
(iii),(RESPHYDPP04_XIE01537_Q03.png width=80%>Thespeedofcenterofmassofthecyl∈dris................<br>(iv),<imga≤ocr_imageload∈g=lazysrc=https://d10lpgp6xz60nq.cloudfront.net/physics_images/RES_PHY_DPP_04_XI_E01_537_Q04.pngwh=80%mx-autosty≤=background:white;>Theanga––rvelocityofthecyl∈deris.............<br>(a) V<br>(b) V//R<br>(c) 2V//R<br>(d) 4V//R<br>(e)ca∩otbedeterminedomthegiven∈formation<br>(f) Zero.
(i)d(ii)b(ii)f(iv)c
(i)e(ii)v(iii)f(iv)b
(i)e(ii)d(iii)f(iv)c
(i)e(ii)v(iii)f(iv)a
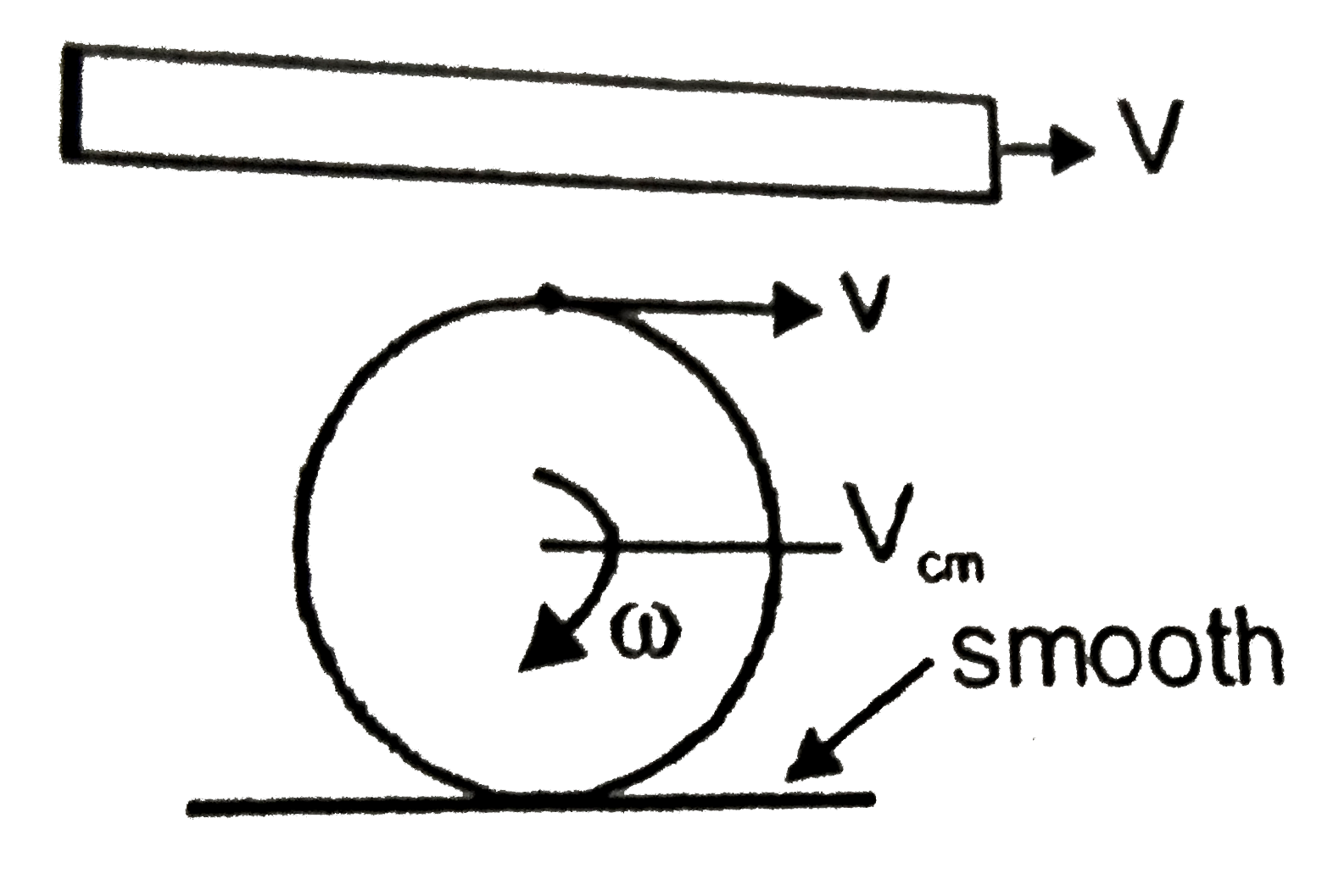
Vcm=ωR=V,Vcm=V−ωR
ω depends on value of friction between plank & cylinder, hence Vcm is undetermined.
(ii)ω=2v2R=VR
(ii)ω=2V2R=VR, hence Vcm=0
(iv)ωA/C=3V+VR=4VR⇒ω=4VR
Topper's Solved these Questions
- DAILY PRACTICE PROBLEMSBOOK - RESONANCECHAPTER - DAILY PRACTICE PROBLEMSEXERCISE - dpp 68
7 Videos
- DAILY PRACTICE PROBLEMSBOOK - RESONANCECHAPTER - DAILY PRACTICE PROBLEMSEXERCISE - dpp 69
5 Videos
- DAILY PRACTICE PROBLEMSBOOK - RESONANCECHAPTER - DAILY PRACTICE PROBLEMSEXERCISE - dpp 66
4 Videos
- CURRENT ELECTRICITYBOOK - RESONANCECHAPTER - CURRENT ELECTRICITYEXERCISE - Exercise
54 Videos
- ELASTICITY AND VISCOCITYBOOK - RESONANCECHAPTER - ELASTICITY AND VISCOCITYEXERCISE - Advanced Level Problems
9 Videos
Similar Questions
A cylinder of mass 2kg and radius 10cm is held between two planks as shown in Fig. Calculate KE of the cylinder when there is no slipping at any point.
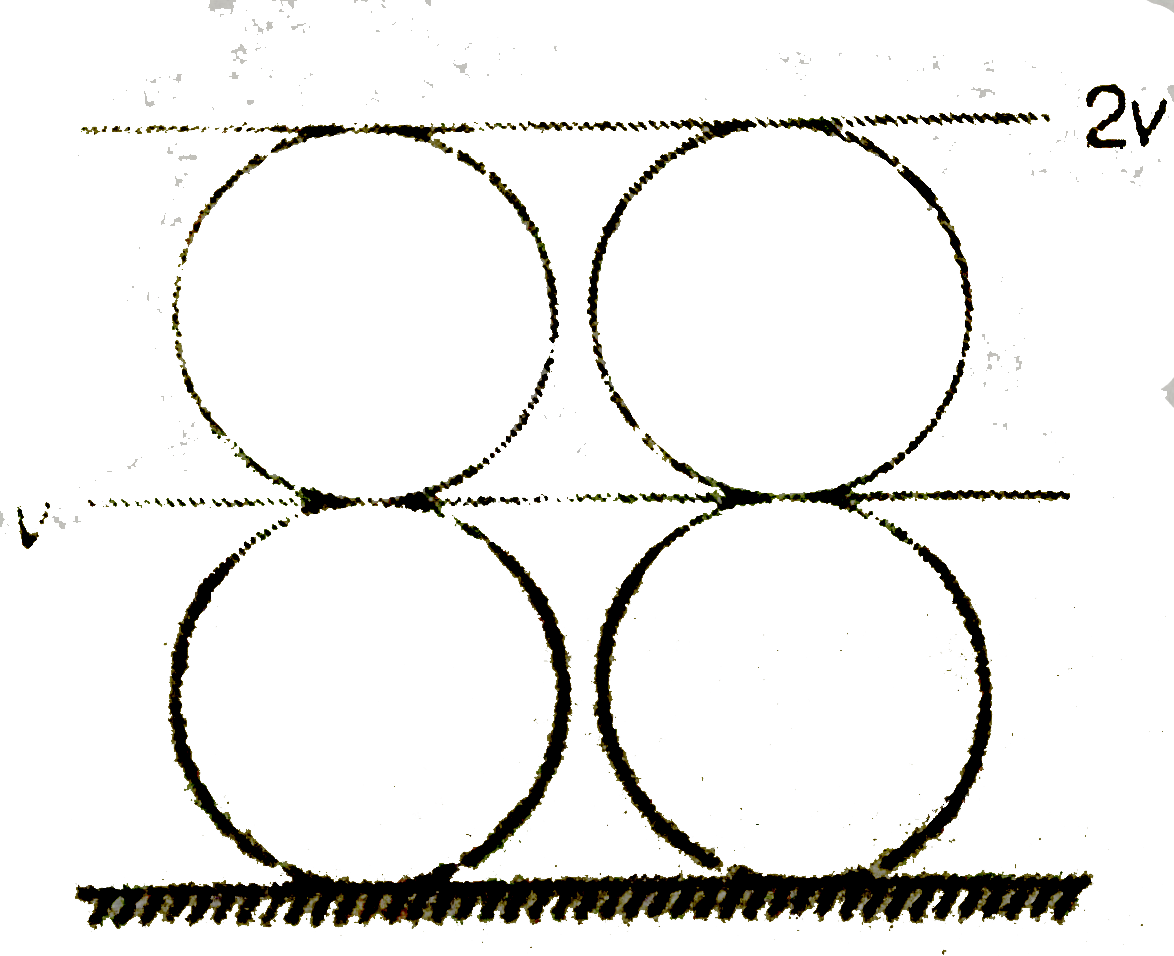
View SolutionTwo thin planks are moving on a four identical cylinders as shown. There is no slipping at any contact points. Calculate the ratio of angular speed of upper cylinder to lower cylinder
View Solution
Knowledge Check
Consider the situation shown in the figure. The wall is smooth but the surfaces of A and B in contact are rough. Then,
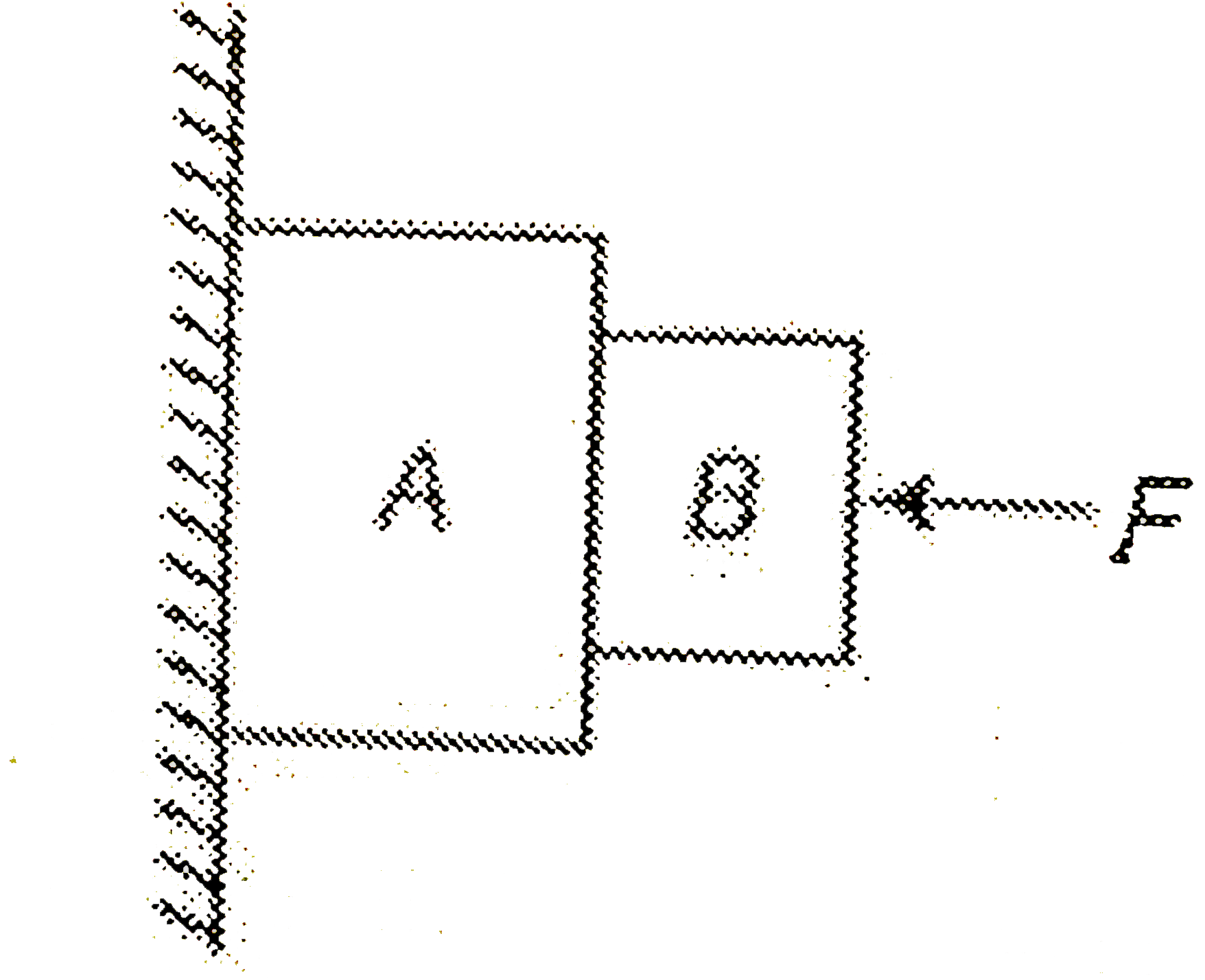 Asystem may remain in equilibriumBboth bodies must move togetherCthe system cannot remain in equilibriumDNone of the above
Asystem may remain in equilibriumBboth bodies must move togetherCthe system cannot remain in equilibriumDNone of the aboveA plank P is placed on a solid cylinder S, which rolls on a horizontal surface. The two are of equal mass. There is no slipping at any of the surfaces in contact. The ratio of kinetic energy of P to the kinetic energy of S is:
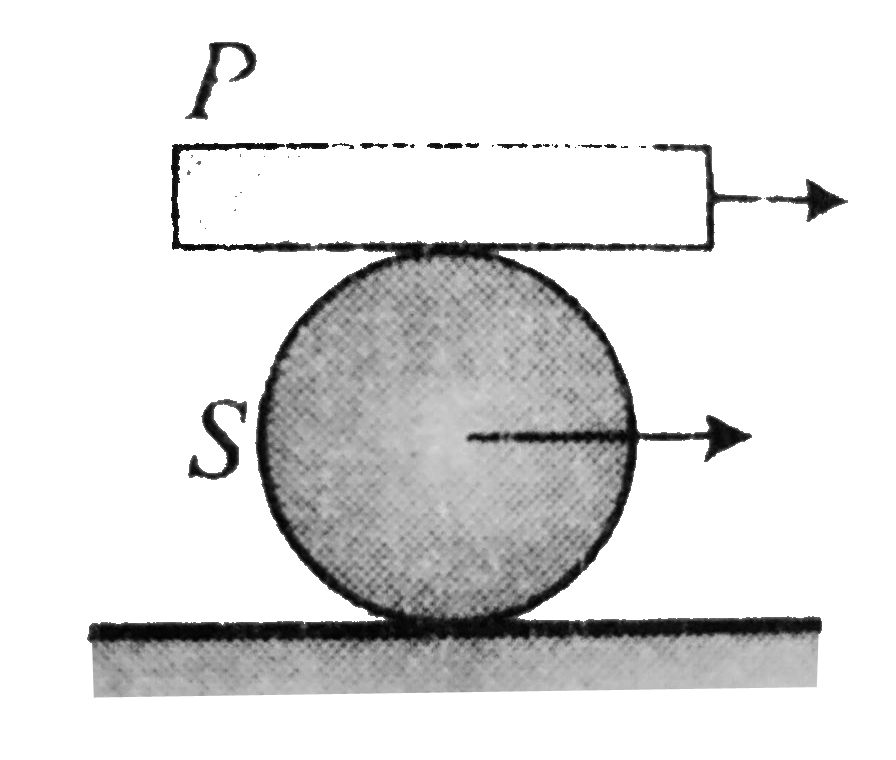 A1:1B2:1C8:3D11:8
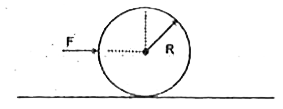
A1:1B2:1C8:3D11:8A solid cylinder of mass M and radius R pure rolls on a rough surface as shown in the figure. Choose the correct alternative (s).
 AThe acceleration of the centre of mass is FMBThe acceleration of the centre of mass is 23FMCThe friction force on the cylinder acts backwardDThe magnitude of the friction force is F3
AThe acceleration of the centre of mass is FMBThe acceleration of the centre of mass is 23FMCThe friction force on the cylinder acts backwardDThe magnitude of the friction force is F3
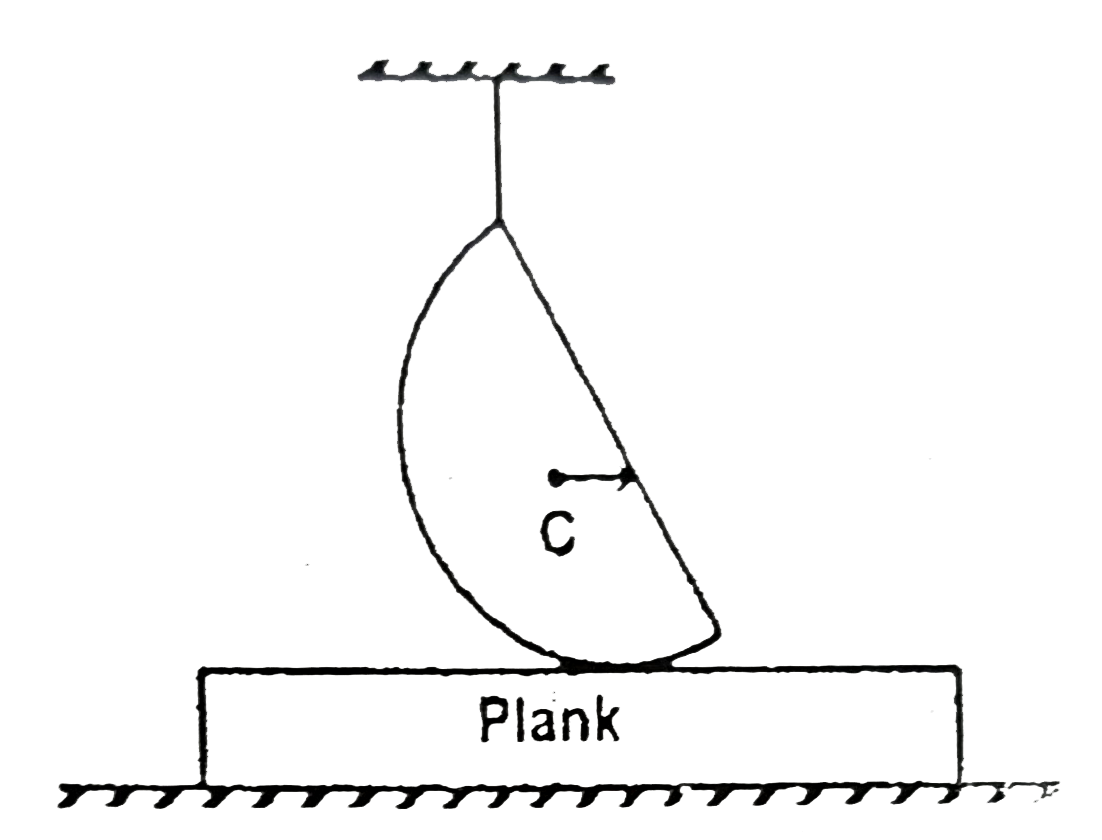
Lower surface of a plank is rough and lying at rest on a rough horizontal surface. Upper surface of the plank is smooth and has a smooth hemisphere placed over it through a light string as shown in the figure. After the string is burnt, trajectory of C.M, of the sphere is
View SolutionA cylinder of mass m and radius R is kept on a rough surface after giving its centre a horizontal speed v0. Find the speed of the centre of the cylinder when it stops slipping.
View SolutionA force F is applied horizontally on a cylinder in the line of centre as shown in the figure. The cylinder is on a rough surface of coefficient of fricition μ. The direction of the friction force acting on the cylinder will be
View SolutionA sphere of radius 1 m is kept on a plank kept on horizontal surface. Accelerations of plank and centre of sphere are indicated in the figure.

If sphere is not slipping on the plank then its angular acceleration is
View SolutionA cylinder is kept at rest on a sufficiently rough horizontal surface. A horizontal constant force is applied at the centre of mass of cylinder.
View Solution
