The drwing shows a bicycle wheel of radius r, resting against a small step whose height is h=r/5. A clockwise torque increases, there comes a time when the wheel just begins to rise up and loses contact with the ground. Let this torque be T. What is the magniitude of the horizontal component of the acceleration of the centre of the wheel when a torque of 2T is applied? Assume that the wheel doesn't slip at the edge of the step when this torque is applied (ignore the mass of the spokes).
The drwing shows a bicycle wheel of radius r, resting against a small step whose height is h = r//5. A clockwise torque increases, there comes a time when the wheel just begins to rise up and loses contact with the ground. Let this torque be T. What is the magniitude of the horizontal component of the acceleration of the centre of the wheel when a torque of 2T is applied? Assume that the wheel doesn't slip at the edge of the step when this torque is applied (ignore the mass of the spokes).

A

4.8m/s2

B

3m/s2

C

2.4m/s2

D

1.5m/s2

Video Solution
Doubtnut Promotions Banner
Text Solution
Verified by Experts

The correct Answer is:C

When the wheel gets just lifted T=mg(3r/5). But when a torque of 2T is applied, we can write:
mg(3r/5)=lα,
where l=2mr2
The horizontal acceleration is rα(4/5).

Updated on:21/07/2023
|
Class 12PHYSICSQUESTION BANK

Topper's Solved these Questions

Similar Questions

Knowledge Check

  • A wheel of radius r and mass m stands in front of a step of height h. The least horizontal force which should be applied to the axle of the wheel to allow it to raise onto the step is
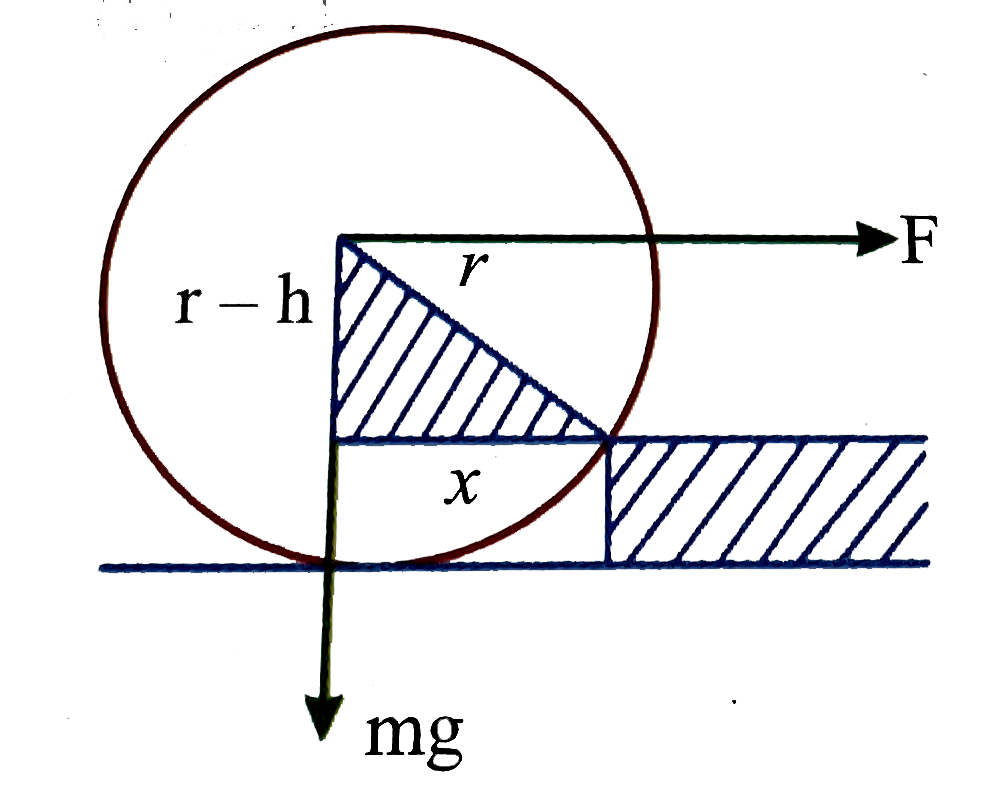    ocr_image

    Amgh(2r−h)r−h
    Bmgh(r−h)
    Cmg(√h(2r−h))r−h
    Dmghr

  • The angular momentum of a wheel changes from 3 L to 7 L in 4 sec. What is the magnitude of torque acting on it ?

    AL
    B2 L
    C3 L
    D4 L

  • A flywheel of moment of inertia I is rotating with uniform angular speed ω. If a torque τ retards the wheel, then the time in which the wheel comes to rest is

    AIωτ
    B2Iωτ
    CIω2τ
    DIω4τ