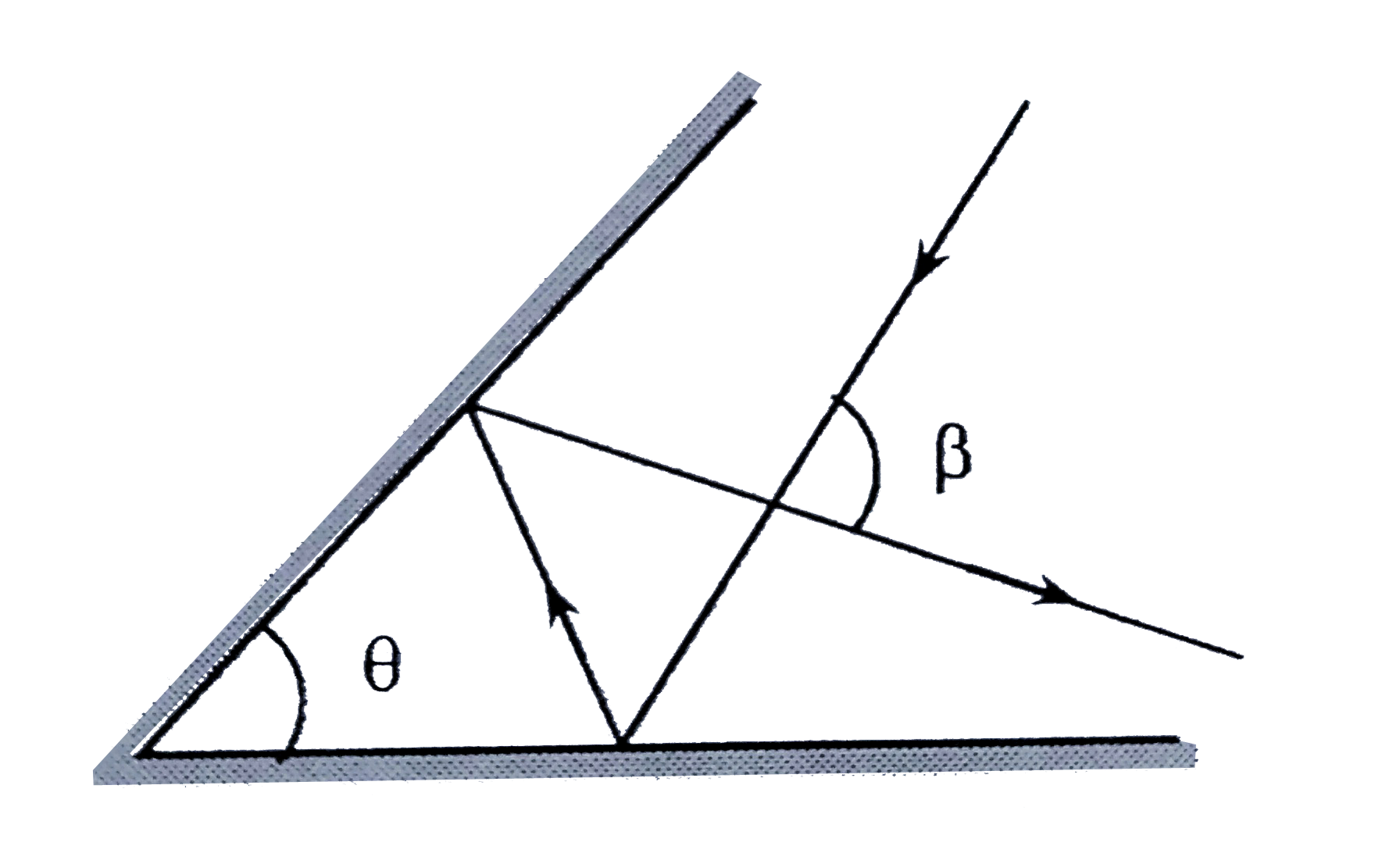
The reflecting surface of two intersecting flat mirrors are at an angle θ(0∘<θ<90∘). As shown in Figure. For a light ray that strikes the horizontal mirror, the emerging ray intersects the incident ray at an angle β . If one of the mirrors is rotated at dθdt=1∘/s, then the angle β changes aat the rate of
1∘/s
2∘/s
−1∘/s
−2∘/s
The correct Answer is:D
From geometry β=(180∘−2θ)
So, dβdt=−2dθdt
Topper's Solved these Questions
- TEST PAPERSBOOK - FIITJEECHAPTER - TEST PAPERSEXERCISE - PHYSICS
747 Videos
- SIMPLE HARMONIC MOTIONBOOK - FIITJEECHAPTER - SIMPLE HARMONIC MOTIONEXERCISE - Assignment Problems (Objective) (Level-II)
20 Videos
- WORK, ENERGY AND POWER BOOK - FIITJEECHAPTER - WORK, ENERGY AND POWER EXERCISE - COMPREHENSIONS ( Comprehension-II)
5 Videos
Similar Questions
Knowledge Check
A ray of light is incident at an angle of 35∘ on a plane mirror. What is angle θ ?
 A35∘B45∘C55∘Dnone of these
A35∘B45∘C55∘Dnone of theseWhen a mirror is rotated by a angle θ , the reflected ray will rotated by
A0∘Bθ/2CθD2θTwo plane mirrors are inclined at angle θ as shown in figure. If a ray parallel to OB strikes the other mirror at P and finally emerges parallel to OA after two reflections then θ is equal to
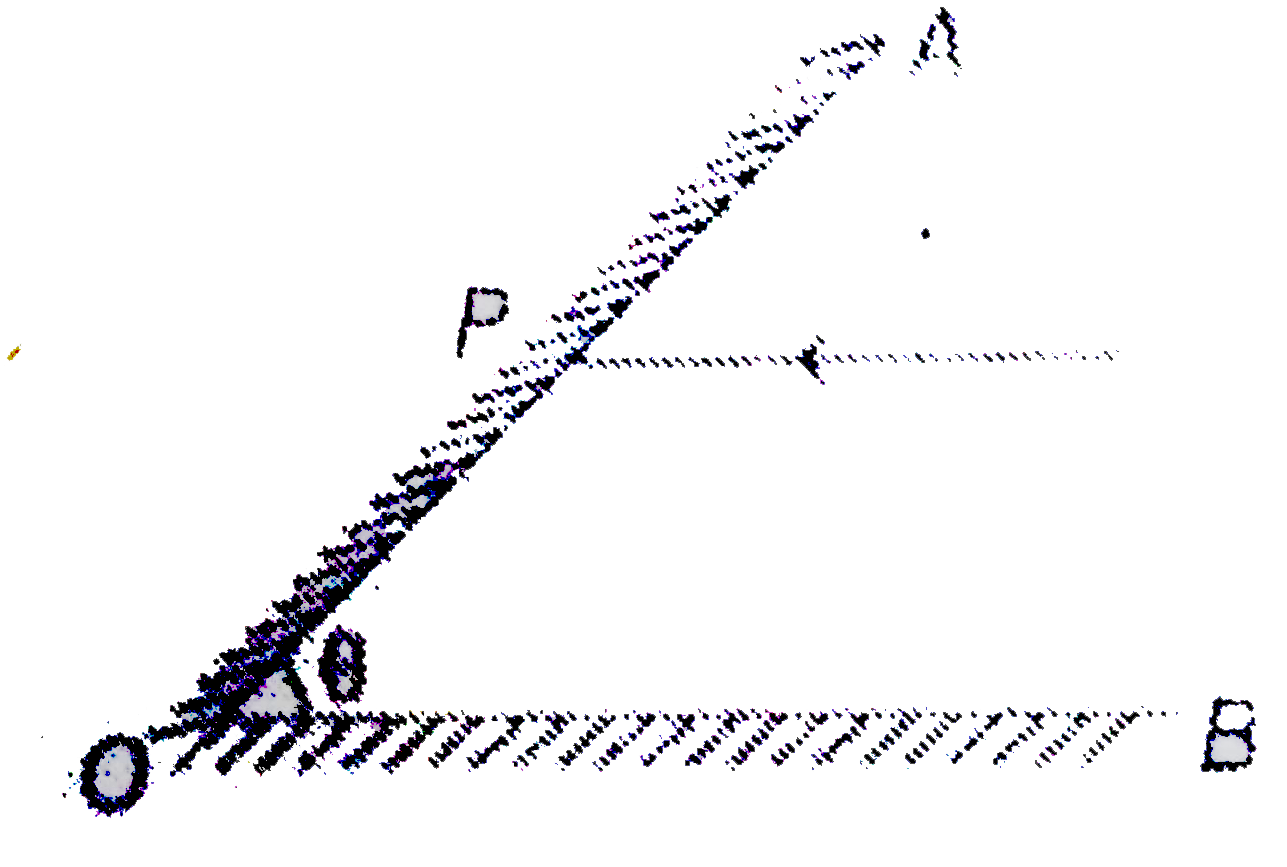 A90∘B60∘C45∘D30∘
A90∘B60∘C45∘D30∘
plane mirror makes an angle of 30∘ with horizontal. If a vertical ray strikes the mirror, find the angle between mirror and reflected ray
View SolutionA ray of light is directed towards a corner reflector as shown. The incident ray makes an angle of 22∘ with one of the mirrors. At what angle θ does the ray emerge?

View SolutionTwo plane mirrors are arranged at right angles to each other as shown in figure.A ray of light is incident on the horizontal mirror at an angle θ. For what value of θthe ray emerges parallel to the incoming ray after reflection from the vertical mirror ?
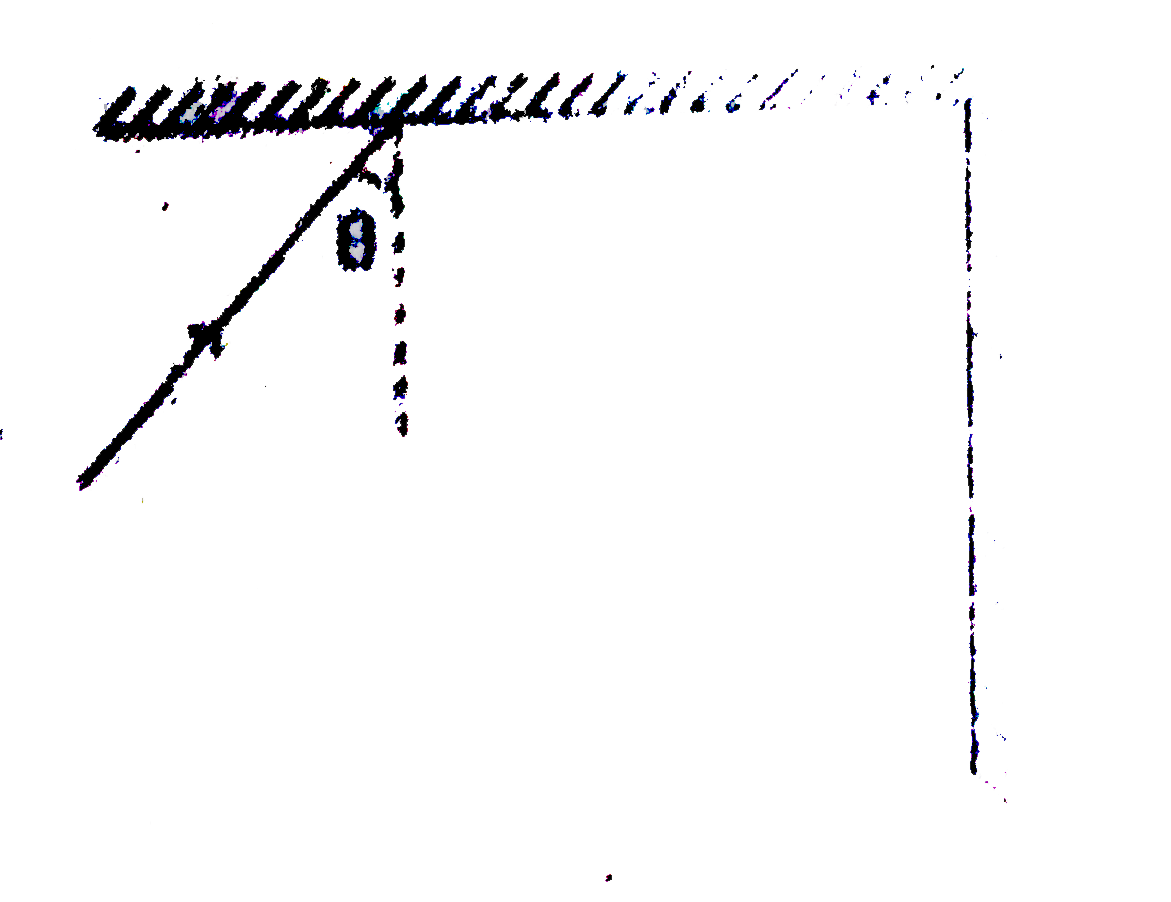
View SolutionA ray of light is reflected by two perpendicular plane mirrors as shown. The angle θ is equal to:

View SolutionPlane mirrors A and B are kept at angle θ with respect to each other . Light falls on a is reflected then falls on B and is reflected. The emergent ray is opposite to the incident direction . The angle θ is equal to
View Solution

