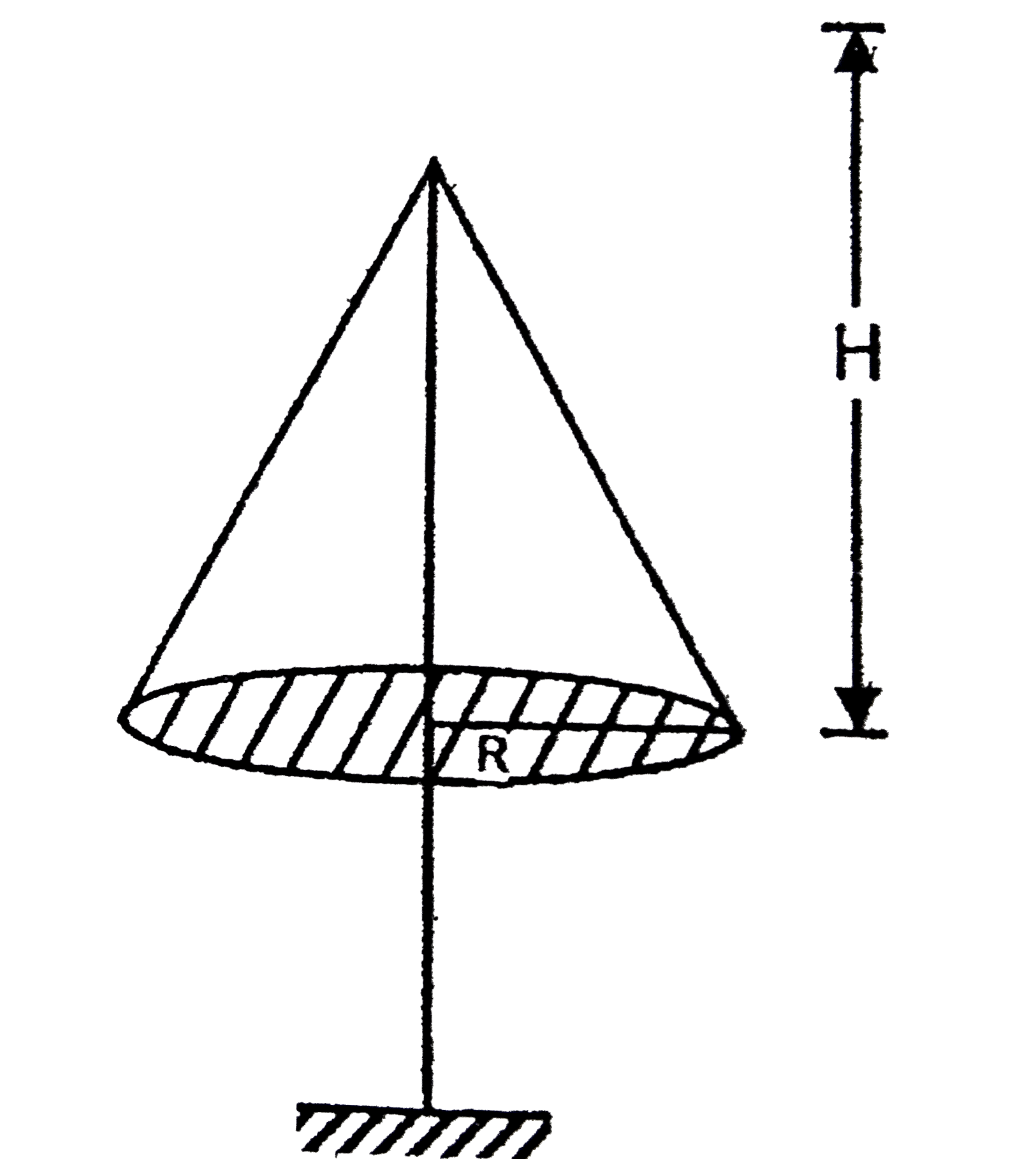
A solid wooden cone has been supported by a string inside water as shown in the figure. The radius of the circular base of the cone is R and the volume of the cone is V. In equilibrium the base of the cone is at a depth H below the water surface. Density of wood is d(<ρ, density of water) Then tension in the string is
Vg(ρ−d)
Vg(d+ρ)
2Vg(ρ−d)
None of these
The correct Answer is:A
(a) Buoyancy force is FB=vρg
Weight of the cone W=vdg
∴ Tension T=W−Fa=vg(d−ρ)
(b) FB−vρg
Buoyancy is resultant of vertically upward force (F1) applied by water perssure on the base of the cone
and the vertically downward force (F2) applied by water on the slant surface. [The horizontal component of this force same up to zero due to symmetry

∴F1−F2=FB
πR2.[ρ0+ρgH]−F2=vρg
∴F2=πR2[ρ0+ρgH]−vρg
Similar Questions
A solid wooden cone has been supported by a string inside water as shown in the figure. The radius of the circular base of the cone is R and the volume of the cone is v. In equilibrium the base of the cone is at a depth H below the water surface. Density of wood is d(<ρ, density of water).
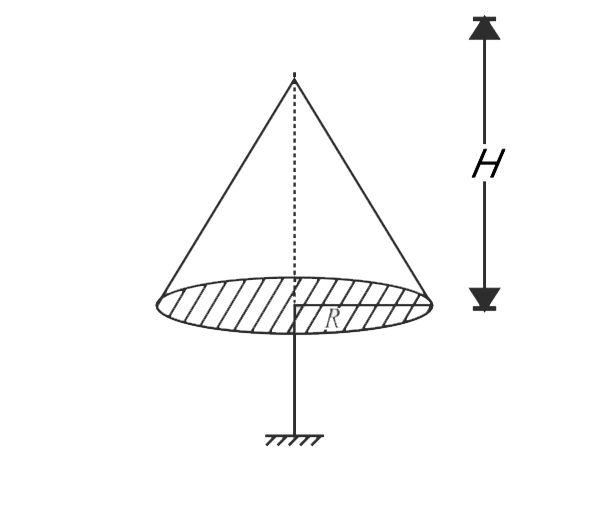
(a) Find tension in the string.
(b) Find the force applied by the water on the slant surface of the coone. take atmospheric pressure to be p0.
View SolutionThe radius of base of a cone is r and height is h. Find its volume.
View Solution
Knowledge Check
The radius of the base of a solid right circular cone is 8 cm and its height is 15 cm. The total surface area of the cone is:
A128 πB200πC136πD120πThe curved surface area of a right circular cone is 156π and the radius of its base is 12 cm. What is the volume of the cone, in cm3 ?
A192πB210πC240πD180πA sphere of radius r has the same volume as that of a cone with a circular base of radius r. Find the height of the cone.
A2rBr3C4rD(23)r
If a sphere of radius 2r has the same volume as that of a cone with circular base of radius r, then find the height of the cone.
View SolutionA solid sphere of radius r is melted and cast into the shape of a solid cone of height r , the radius of the base of the cone is 2r (b) 3r (c) r (d) 4r
View SolutionShow that the right circular cone of least curved surface and given volume has an altitude equal to √2time the radius of the base.
View SolutionA toy is in the form of a hemisphere surmounted by a right circular cone of the same base radius as that of the hemisphere. If the radius of the base of the cone is 21cm and its volume is 2/3 of the volume of the hemisphere, calculate the height of the cone and the surface area of the toy. (Useπ=227).
View SolutionThe volume of a right circular cone is 1232 cm^3. If the radius of its base is 14 cm, find its curved surface.
View Solution
