In ABC, ∠A=1000and AB=AC. Find ∠B and ∠C
To solve the problem, we need to find angles B and C in triangle ABC, where angle A is given as 100 degrees and sides AB and AC are equal.
1. Identify Given Information:
- Angle A = 100 degrees
- AB = AC (This means triangle ABC is isosceles with AB and AC as the equal sides)
2. Use the Property of Isosceles Triangles:
- In an isosceles triangle, the angles opposite the equal sides are equal. Therefore, angle B = angle C.
3. Apply the Triangle Sum Property:
- The sum of the angles in any triangle is 180 degrees. Thus, we can write the equation:
Angle A+Angle B+Angle C=180∘
- Substituting the known values:
100∘+Angle B+Angle C=180∘
4. Substitute Angle B for Angle C:
- Since angle B = angle C, we can replace angle C in the equation:
100∘+Angle B+Angle B=180∘
- This simplifies to:
100∘+2⋅Angle B=180∘
5. Solve for Angle B:
- Rearranging the equation gives:
2⋅Angle B=180∘−100∘
- Simplifying further:
2⋅Angle B=80∘
- Dividing both sides by 2:
Angle B=40∘
6. Find Angle C:
- Since angle B = angle C, we have:
Angle C=40∘
Final Answers:
- Angle B = 40 degrees
- Angle C = 40 degrees
- Angle A = 100 degrees
- AB = AC (This means triangle ABC is isosceles with AB and AC as the equal sides)
- In an isosceles triangle, the angles opposite the equal sides are equal. Therefore, angle B = angle C.
- The sum of the angles in any triangle is 180 degrees. Thus, we can write the equation:
Angle A+Angle B+Angle C=180∘
- Substituting the known values:
100∘+Angle B+Angle C=180∘
- Since angle B = angle C, we can replace angle C in the equation:
100∘+Angle B+Angle B=180∘
- This simplifies to:
100∘+2⋅Angle B=180∘
- Rearranging the equation gives:
2⋅Angle B=180∘−100∘
- Simplifying further:
2⋅Angle B=80∘
- Dividing both sides by 2:
Angle B=40∘
- Since angle B = angle C, we have:
Angle C=40∘
- Angle B = 40 degrees
- Angle C = 40 degrees
Topper's Solved these Questions
Similar Questions
ABCD is a cyclic quadrilateral in which: BCAD, ∠ADC=1100 and ∠BAC=500. Find ∠DAC ∠DBC=800 and ∠BAC=400. Find ∠BCD ∠BCD=1000and ∠ABD=700 find ∠ADB
View SolutionIn a parallelogram ABCD, the diagonals bisect each other at O. If ∠ABC=300, ∠BDC=100and ∠CAB=700, Find: ∠DAB, ∠ADC, ∠BCD, ∠AOD, ∠DOC, ∠BOC, ∠AOB, ∠ACD, ∠CAB, ∠ADB
Given that
∠ABC=302,∠ABC=∠ADC=300
[We know that measure of opposite angles are equal in a parallelogram]
∠BDC=100
∠CAB=700
∠BDA=∠ADB=∠ADC–∠BDC
302–102=202
∠DAB=1800–300=1500
∠ADB=∠DBC=200 (alternate angles)
∠BCD=∠DAB=1500
[we know, opposite angles are equal in a parallelogram]
∠DBA=∠BDC=100
[we know, Alternate interior angles are equal]
InΔABC
∠CAB+∠ABC+∠BCA=1800
[since, sum of all angles of a triangle is 180^0]
700+300+∠BCA=1800
∠BCA=1800–1000=800
∠DAB=∠DAC+∠CAB=700+800=1500
∠BCD=1500 (opposite angle of the parallelogram)
∠DCA=∠CAB=700
In ΔDOC∠BDC+∠ACD+∠DOC=1800
[since, sum of all angles of a triangle is 180^0]
100+700+∠DOC=1800
∠DOC=1800−800
∠ACB=800
View SolutionIn ABC, if ∠A=1000, AD bisects ∠A and ∠AD ⊥BC . Then, ∠B= 500 (b) 900 (c) 400 (d) 1000
View SolutionIn square ABCD, P and Q are mid-point of AB and CD respectively. If AB=8cm and PQ and BD intersect at O, then find area of OPB
View SolutionIn Fig.81, side BC of ABC has been produced to D and CEBA. If ∠ABC=650, ∠BAC=550, find ∠ACE, ∠ECD and ∠ACD.
View SolutionIn Fig. 71, if ABCD and CDEF, find ∠ACE
View SolutionIn a ABC, If LandM are points on ABandAC respectively such that LMparallelBC
Prove that: ar(LBC)=ar(MBC)
View SolutionIn a ABC , If LandM are points on ABandAC respectively such that LMparallel BC
Prove that: ar(LOB)=ar(MOC)
View SolutionIn a ABC, If LandM are points on ABandAC respectively such that LMparallelBC
Prove that: ar(LCM)=ar(LBM)
View SolutionIn Figure, ABCD is a parallelogram in which ∠DAO=400, ∠BAO=350 and ∠COD=650. Find: ∠ABO (ii) ∠ODC (iii) ∠ACB (iv) ∠CBD
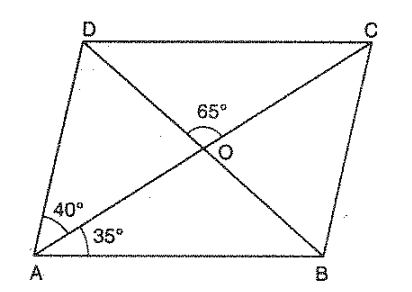
View Solution
