A particle is projected with an initial velocity of 200m/s in a direction making an angle of 30∘ with the vertical. The horizontal distance covered by the particle in 3s is
300m
150m
175m
125m
The correct Answer is:A
To solve the problem of finding the horizontal distance covered by a particle projected with an initial velocity of 200 m/s at an angle of 30 degrees with the vertical, we can follow these steps:
Step 1: Understand the components of the initial velocity
The initial velocity v0=200m/s is given at an angle of 30∘ with the vertical. To find the horizontal and vertical components of the velocity, we can use trigonometric functions.
- The angle with the horizontal will be 90∘−30∘=60∘.
Step 2: Calculate the horizontal component of the velocity
The horizontal component vx can be calculated using:
vx=v0⋅cos(60∘)
Substituting the values:
vx=200⋅cos(60∘)=200⋅12=100m/s
Step 3: Use the horizontal component to find the distance
The horizontal distance x covered in time t can be calculated using the formula:
x=vx⋅t
Given t=3s:
x=100⋅3=300m
Conclusion
The horizontal distance covered by the particle in 3 seconds is 300meters.
---
The initial velocity v0=200m/s is given at an angle of 30∘ with the vertical. To find the horizontal and vertical components of the velocity, we can use trigonometric functions.
The horizontal component vx can be calculated using:
vx=v0⋅cos(60∘)
Substituting the values:
vx=200⋅cos(60∘)=200⋅12=100m/s
The horizontal distance x covered in time t can be calculated using the formula:
x=vx⋅t
Given t=3s:
x=100⋅3=300m
The horizontal distance covered by the particle in 3 seconds is 300meters.
Topper's Solved these Questions
- MOTION IN A PLANEBook:NARAYNAChapter:MOTION IN A PLANEExercise:Level-II(C.W)
Explore 45 Videos
- MOTION IN A PLANEBook:NARAYNAChapter:MOTION IN A PLANEExercise:Level-III(C.W)
Explore 48 Videos
- MOTION IN A PLANEBook:NARAYNAChapter:MOTION IN A PLANEExercise:C.U.Q
Explore 36 Videos
- MECHANICAL PROPERTIES OF SOLIDSBook:NARAYNAChapter:MECHANICAL PROPERTIES OF SOLIDSExercise:LEVEL-II (H.W)
Explore 24 Videos
- MOTION IN A STRAIGHT LINEBook:NARAYNAChapter:MOTION IN A STRAIGHT LINEExercise:Level 2 H.W
Explore 29 Videos
Similar Questions
A particle Is projected at point 'A' with initial velocity 5m/s at an angle θ=37∘ with the vertical y axis. A strong horizontal wind gives the particle a constant horizontal acceleration 6m/s2 in the x direction. If the particle strikes the ground at a point directly under its released position, determine the height 'h' of point A. The downward acceleration is taken as the constant
g=10m/s2(take sin37∘=35,cos37∘=45)
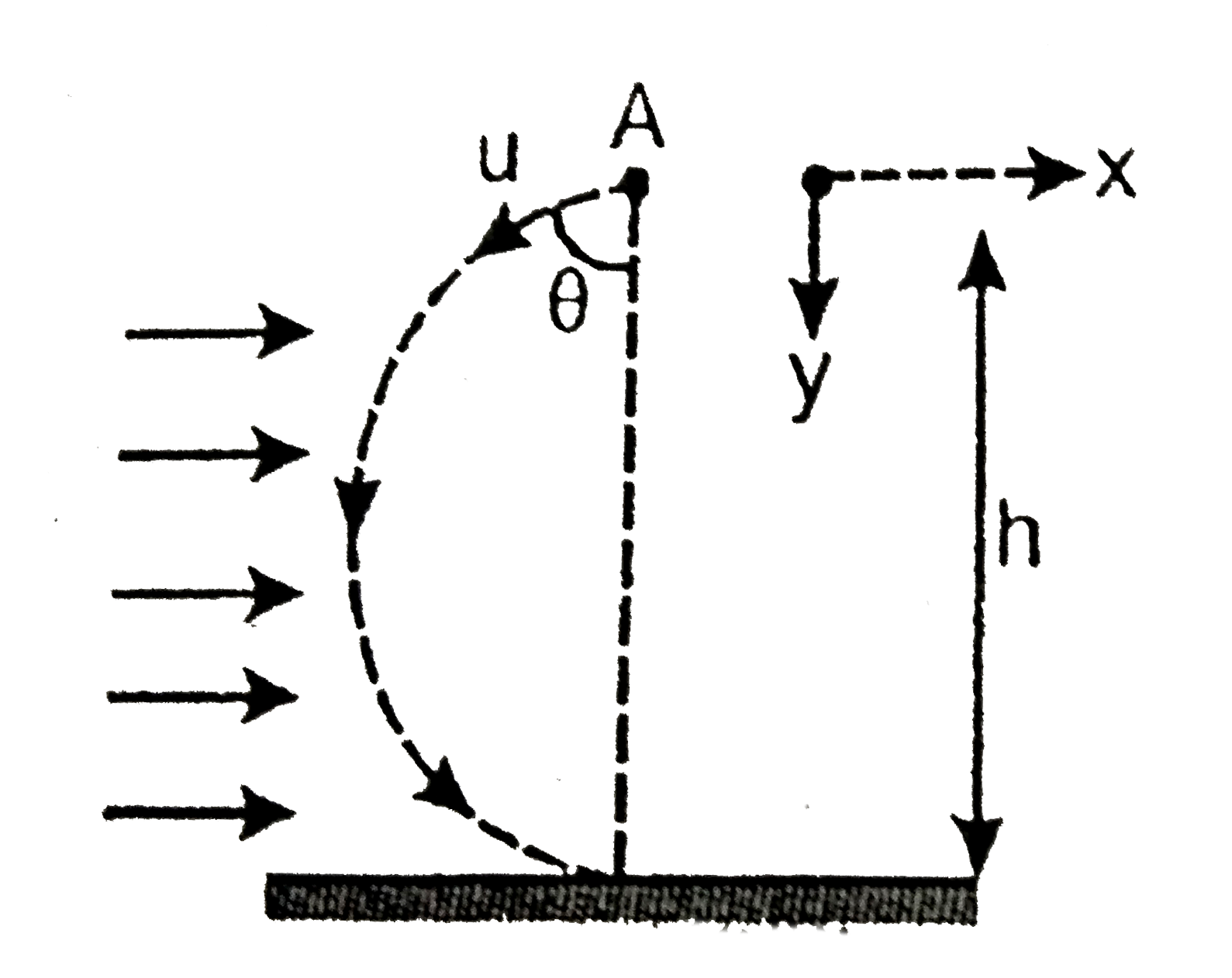
View SolutionIf a particle is projected with a velocity 49m/sec. making an angle 60∘ with the horizontal, its time of flight is given by
View Solution
Knowledge Check
A particle A is projected from the ground with an initial velocity of 10m/s at an angle of 60∘ with horizontal. From what height should an another particle B be projected horizontally with velocity 5m/s so that both the particles collide in ground at point C if both are projected simultaneously g=10m/s2.
 A10mB15mC20mD30m
A10mB15mC20mD30mA particle is projected from the ground with an initial velocity of 20m/s at an angle of 30∘ with horizontal. The magnitude of change in velocity in a time interval from t=0 to t=0.5s is (g=10m/s2)
A5m/sB2.5m/sC2m/sD4m/sA particle is projected upwards with a velocity of 110m/sec at an angle of 60∘ with the vertical. Find the time when the particle will move perpendicular to its initial direction, taking g =10m/sec2
A10 secondsB22 secondsC5 secomdsD10√3 seconds
A particle is projected upwards with a velocity of 100m/sec at an angle of 60∘ with the vertical. Find the time when the particle will move perpendicular to its initial direction, taking g=10m/sec2.
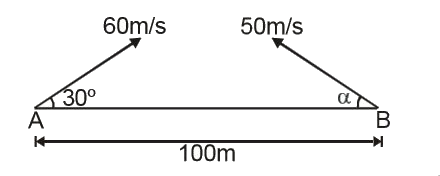
View SolutionA particle A is projected with an initial velocity of 60 m/s. at an angle 30∘ to the horizontal. At the same time a second particle B is projected in opposite direction with initial speed of 50 m/s from a point at a distance of 100 m from A. If the particles collide in air, find (a) the angle of projection ∝ of particle B,
View SolutionA particle A is projected with an initial velocity of 60m/s at an angle 30∘ to the horizontal. At the same time a second particle B is projected in opposite direction with initial speed of 50m/s from a point at a distance of 100 m from A. If the particles collide in air, find (a)the angle of projection α of particle B, (b) time when the collision takes place and (c) the distance of P from A, where collision occurs. (g=10m/s2)
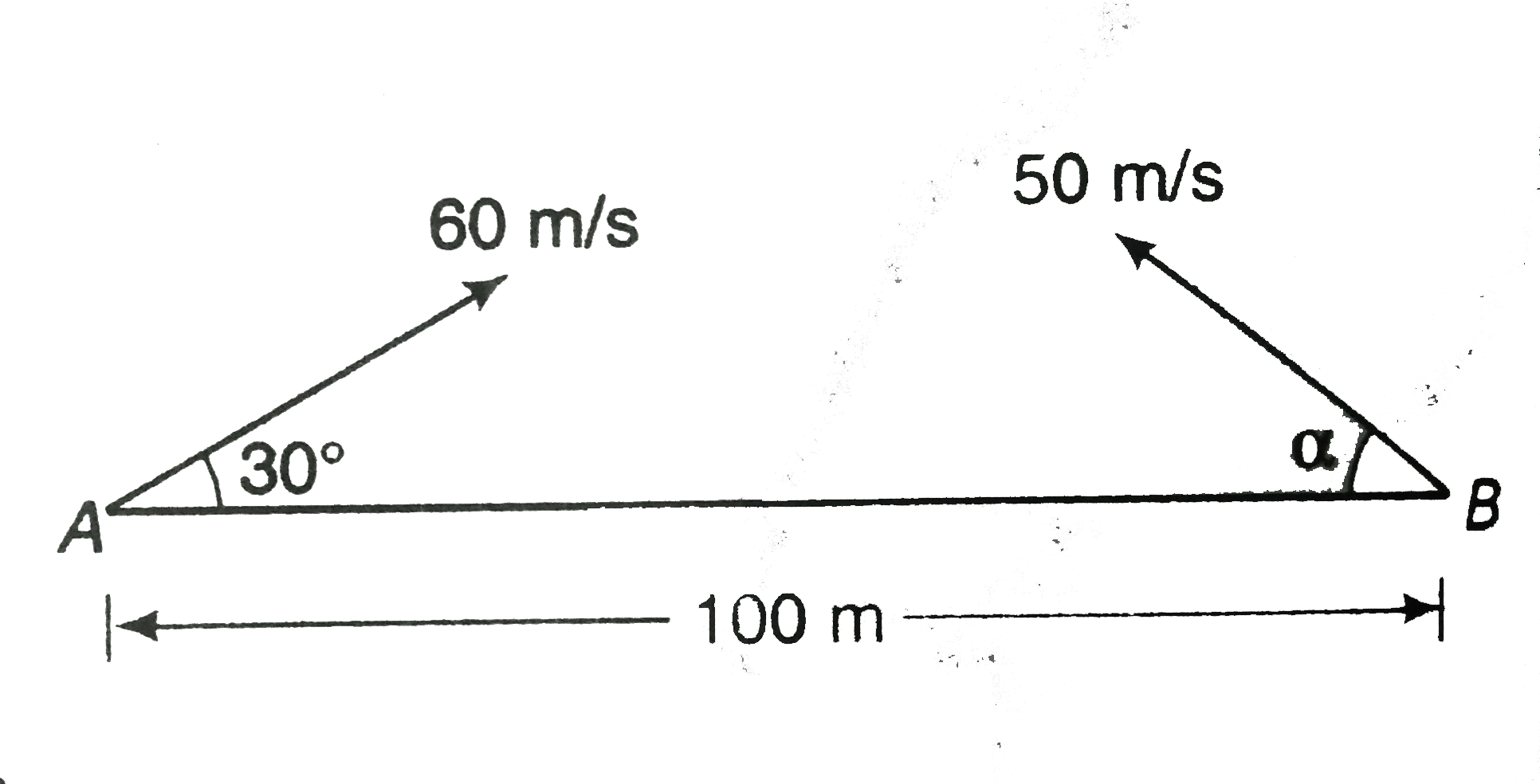
View SolutionIf a particle is projected with a velocity 49m/s making an angle 60∘ with the horizontal, its time of flight is
View SolutionA particle A is projected with an initial velocity of 60m/s at angle 30∘ to the horizontal . At the same time a second particle B is projected in opposite directions with initial speed of 50m/s from a point at a distance of 100m from A.
if the particle collides in air,

Answer the following questions:
Time of collisions will be:
View Solution
