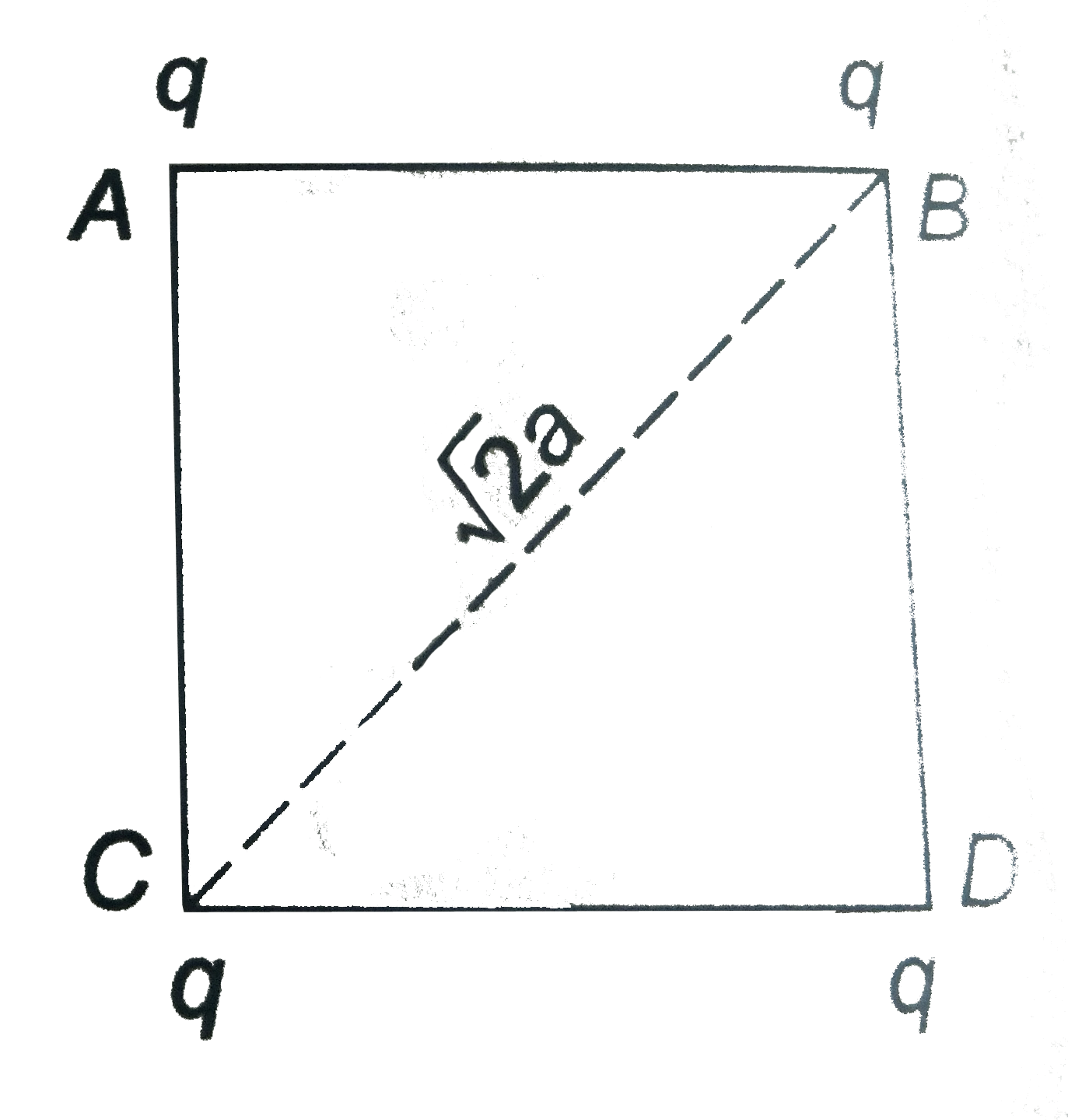
Equal charges q are placed at the four corners A,B,C,Dof a square of length a. The magnitude of the force on the charge at B will be
3q24πε0a2
4q24πε0a2
(1+2√22)q24πε0a2
(√2+1√2)q24πε0a2
The correct Answer is:3
Force on qatB:
Due to q→A:F1=14π∈0q2a2 , along AB
Due to qatD:F2=14π∈0q2a2 , along DB
Due to qatC:F3=14π∈0q2(√2a)2,CB
The resultant of F1 and F2,F'=√2.14π∈0q2a2 along CB
Resultant force on qatB
F0=F'+F3=14π∈0q2q2[√2+12]
=(1+2√22)q24π∈0a2
Topper's Solved these Questions
Similar Questions
Four identical point charges q are placed at four corners of a square of side a. Find the potential energy of the charge system.
 .
.
View SolutionConsider four equal charges (q, each) placed on the corners of a square with side a. Determine the magnitude and direction of the resultant force on the charge on lower right corner.
View Solution
Knowledge Check
Equal charges q are placed at the three corners B, C, D of a square ABCD of side a. The potential at A is
A14πε0qaB3q4πε0Cq4πε0a[2+1√2]Dq4πε0a[1+√2]Equal charges q are placed at the three corners B, C, D of a square ABCD of side 'a'. The ratio of potential at A to centre O is
A2√2+13√2B2+√26C2√2+16D2√2+13q,2q, 3q and 4q charges are placed at the four corners A,B,C and D of a square. The field at the centre P of the square has the direction parallel to
 AABBCBCACDBD
AABBCBCACDBD
Four identical charges q are placed at the corners of a square of side a. Find the potential energy of one of the charges due to the remaining charges.
 .
.
View SolutionFour equal charges Q are placed at the four corners of a square of each side is 'a'. Work done in removing a charge −Q from its centre to infinity is
View SolutionEqual charges q are placed at the vertices A and B of an equilatral triangle ABC of side a. The magnitude of electric field at the point C is
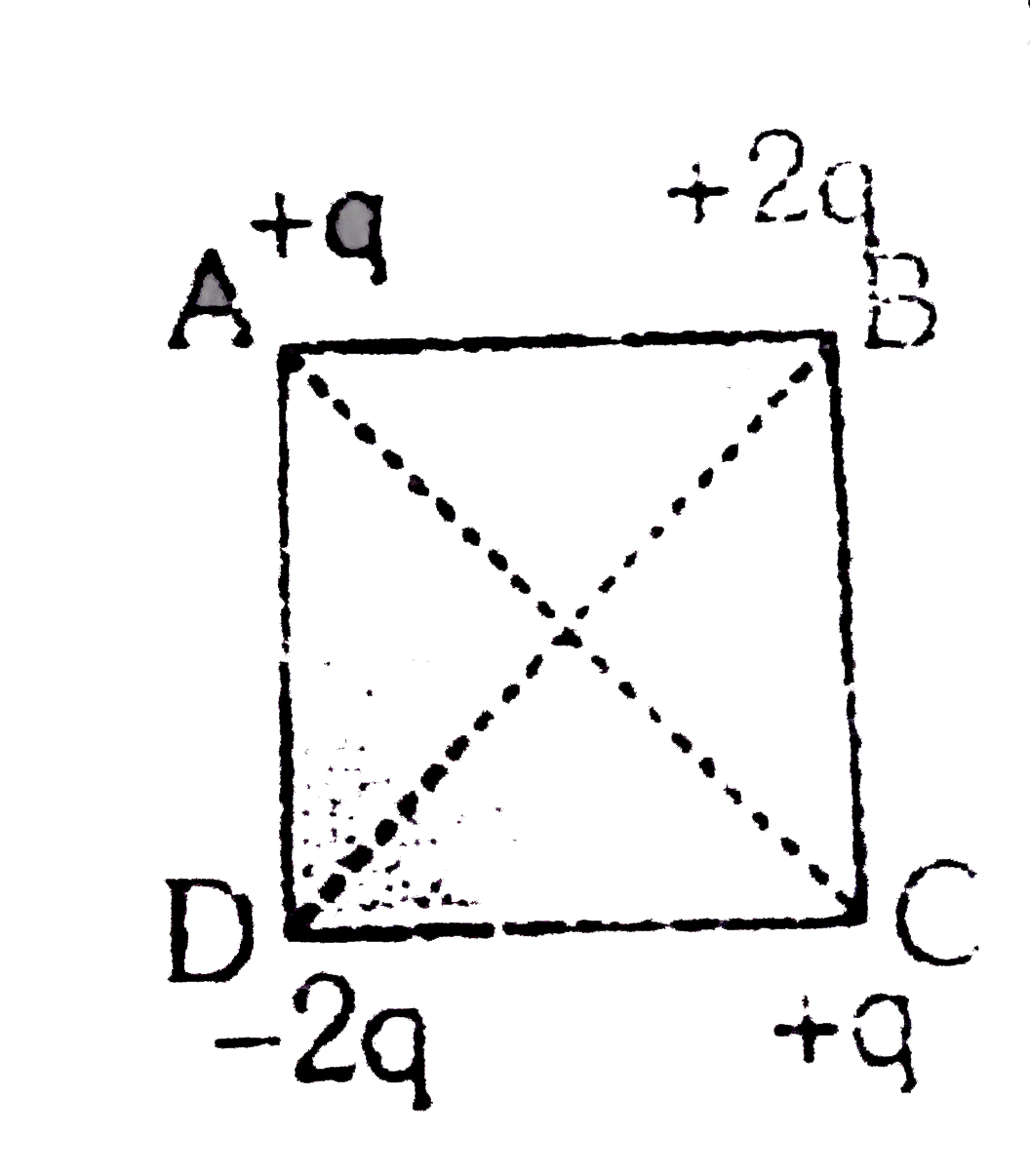
View SolutionFour charges are arranged at the corners of a square ABCD as shown in the figure. The force on the charge kept at the centre O will be:
View SolutionThree point charges each of value +q are placed on three vertices of a square of side a metre. What is the magnitude of the force on a point charge of value −q coulomb placed at the centre of the square?
View Solution
