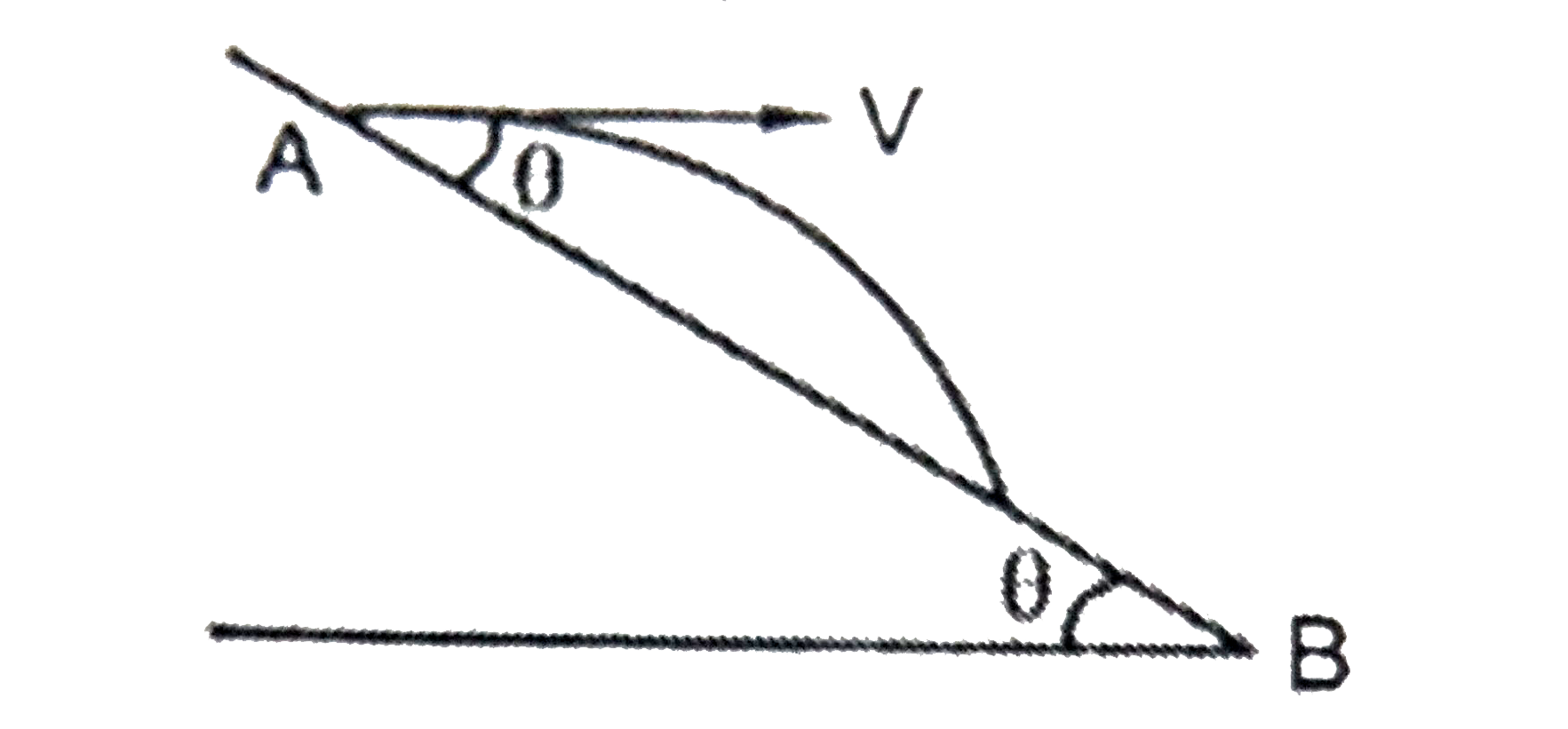
A particle is to be projected horizontally with velocity $v$ from a point $P$ , which is $60 m$ above the foot of a plane inclined at angle $45^{\circ}$ with horizontal as shown in figure. The particle hits the plane perpendicularly at $A$ . After rebound from inlined plane it again hits at $B$ . If coefficient of restitution between particles and plane is $\frac{1}{\sqrt{2}}$ then,

A

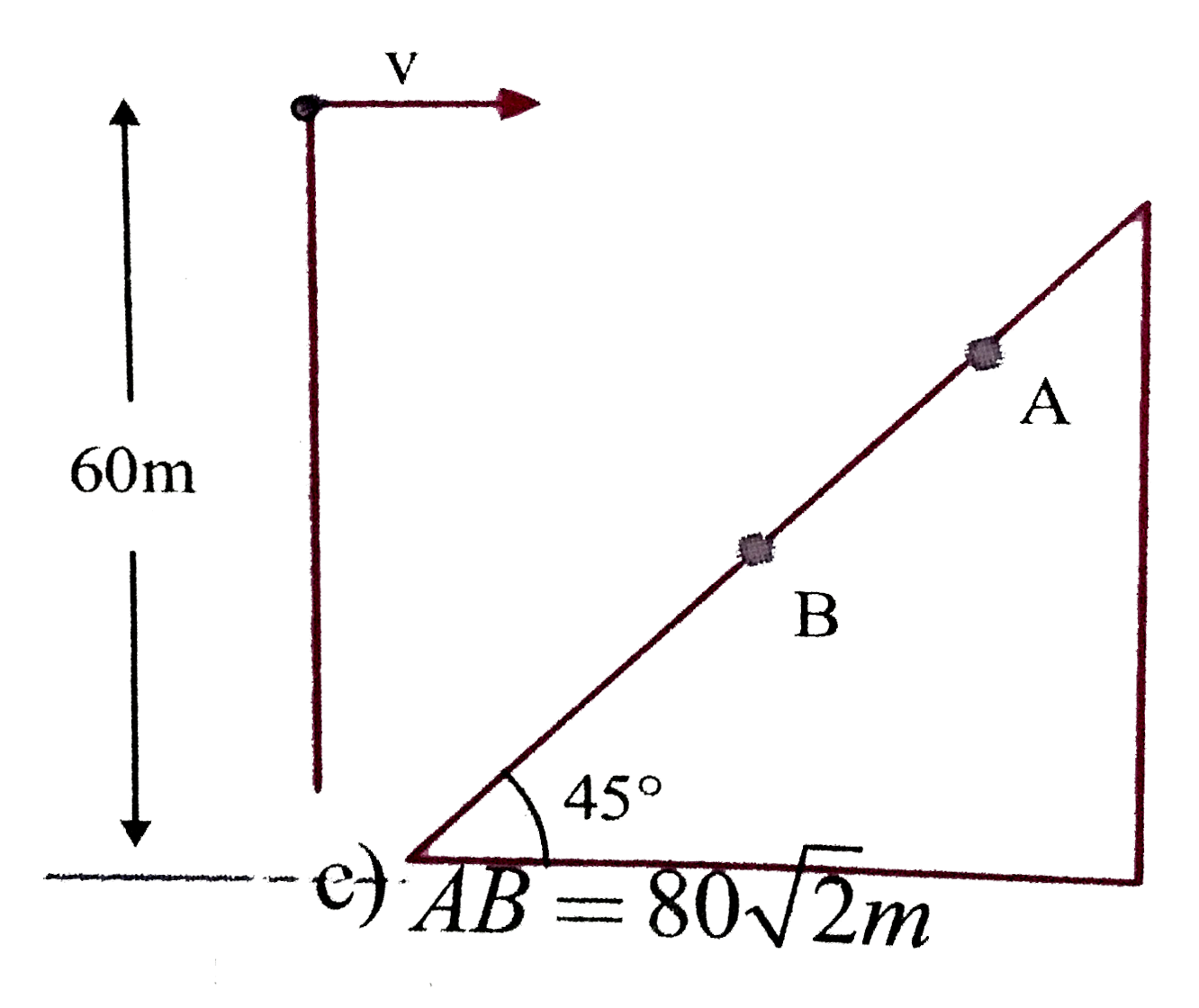
$v = 20 m / s$

B

$v = 10 m / s$

C

$A B = 80 \sqrt{2}$ m

D

$A B = 80 m$

Video Solution

Text Solution

Verified by Experts

The correct Answer is:A, C

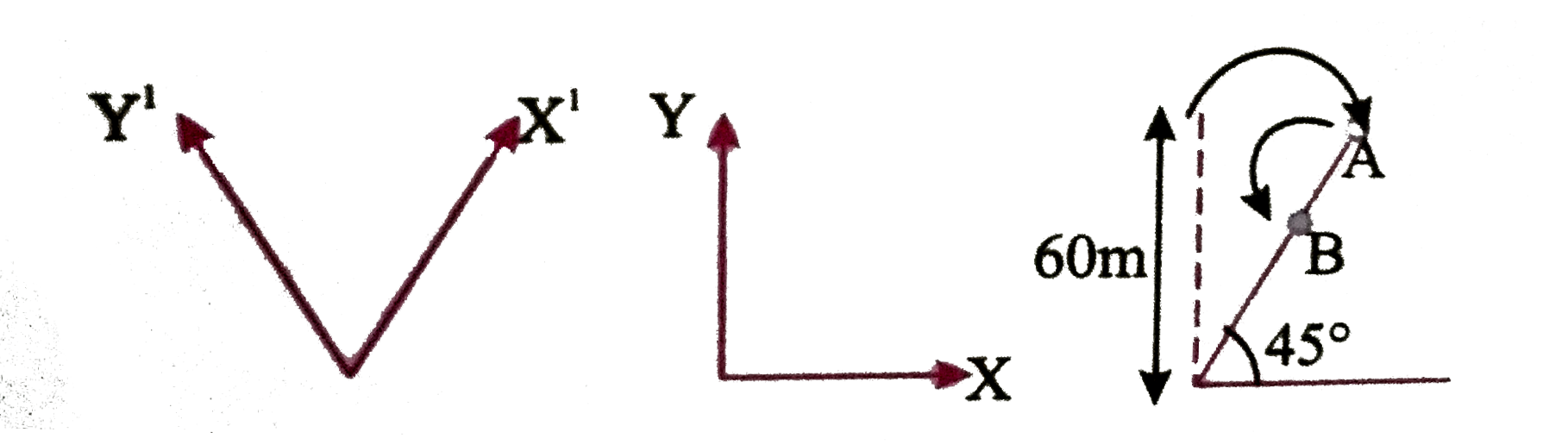
$\begin{matrix} u_{x}^{1} = v {cos 45}^{0}, a_{x}^{1} = - g {sin 45}^{0} u_{y}^{1} = - v {sin 45}^{0}, a_{y}^{1} = - g {cos 45}^{0} \end{matrix}$
At point
$v_{x}^{1} = 0 \Rightarrow 0 = v {cos 45}^{0} - g {sin 45}^{0} t, t = v / g$
${Tan 45}^{0} = \frac{h - \frac{1}{2} g t^{2}}{v t}$ , $h - \frac{1}{2} g t^{2} = v t$
$h = \frac{1}{2} g [\frac{v^{2}}{g^{2}}] + \frac{v^{2}}{g}$
$h = \frac{v^{2}}{g} + \frac{v^{2}}{2 g} = \frac{3 v^{2}}{2 g}$ , $v = \sqrt{\frac{2 g h}{3}} = 20 m / s$
$v' =$ velocity just before collision $= \sqrt{2}$
$v'' =$ velocity after collision $= v$
$t_{A B} = \frac{2 v}{g {cos 45}^{0}} = 2 \sqrt{2} \frac{v}{g} = 4 \sqrt{2} sec$
$A B = \frac{1}{2} \times 10 \times \frac{1}{\sqrt{2}} \times 16 \times 2 = 80 {\sqrt{2}}_{m}$

|

Updated on:21/07/2023

Class 11 PHYSICS CENTRE OF MASS

Topper's Solved these Questions

Similar Questions

Knowledge Check

Question 1 - Select One
A particle is projected horizontally with a speed V=5m/s from the top of a plane inclined at an angle $θ = 37^{\circ}$ to the horizontal $(g = 10 m / s^{2})$

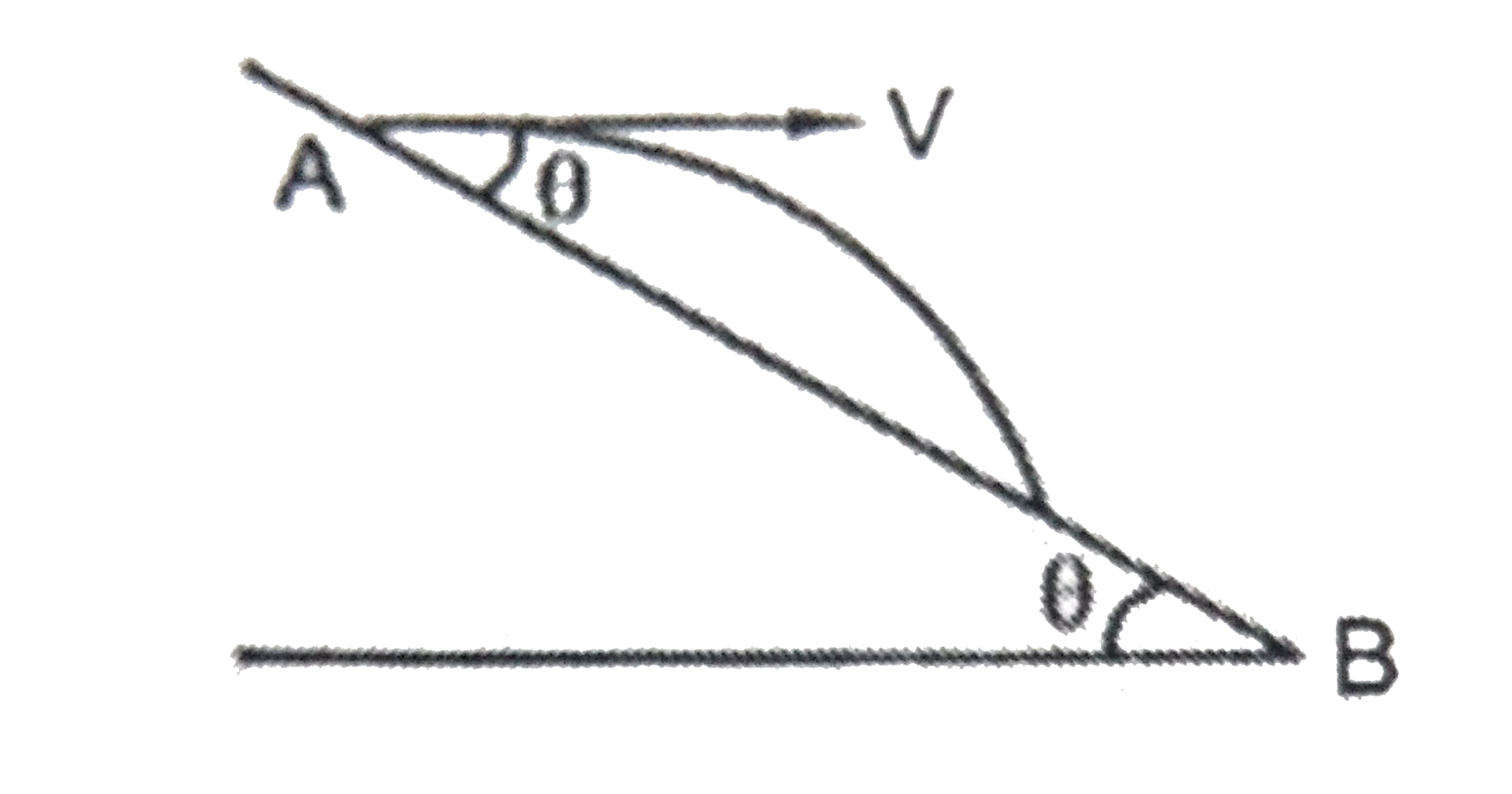
What is the velocity of the particle just before it hits the plane?
A $5 \sqrt{3} m / s$
B $\frac{5}{2} \sqrt{13} m / s$
C $10 \sqrt{13} m / s$
D $5 \sqrt{26} m / s$
Question 2 - Select One
A ball is projected horizontally with a speed v from the top of the plane inclined at an angle $45^{\circ}$ with the horizontal. How far from the point of projection will the ball strikes the plane?
A $\frac{2 v^{2}}{g}$
B $\sqrt{2} [\frac{2 v^{2}}{g}]$
C $\frac{v^{2}}{g}$
D $\sqrt{2} \frac{v^{2}}{g}$
Question 3 - Select One
A particle is projected horizontally with a speed V=5m/s from the top of a plane inclined at an angle $θ = 37^{\circ}$ to the horizontal $(g = 10 m / s^{2})$

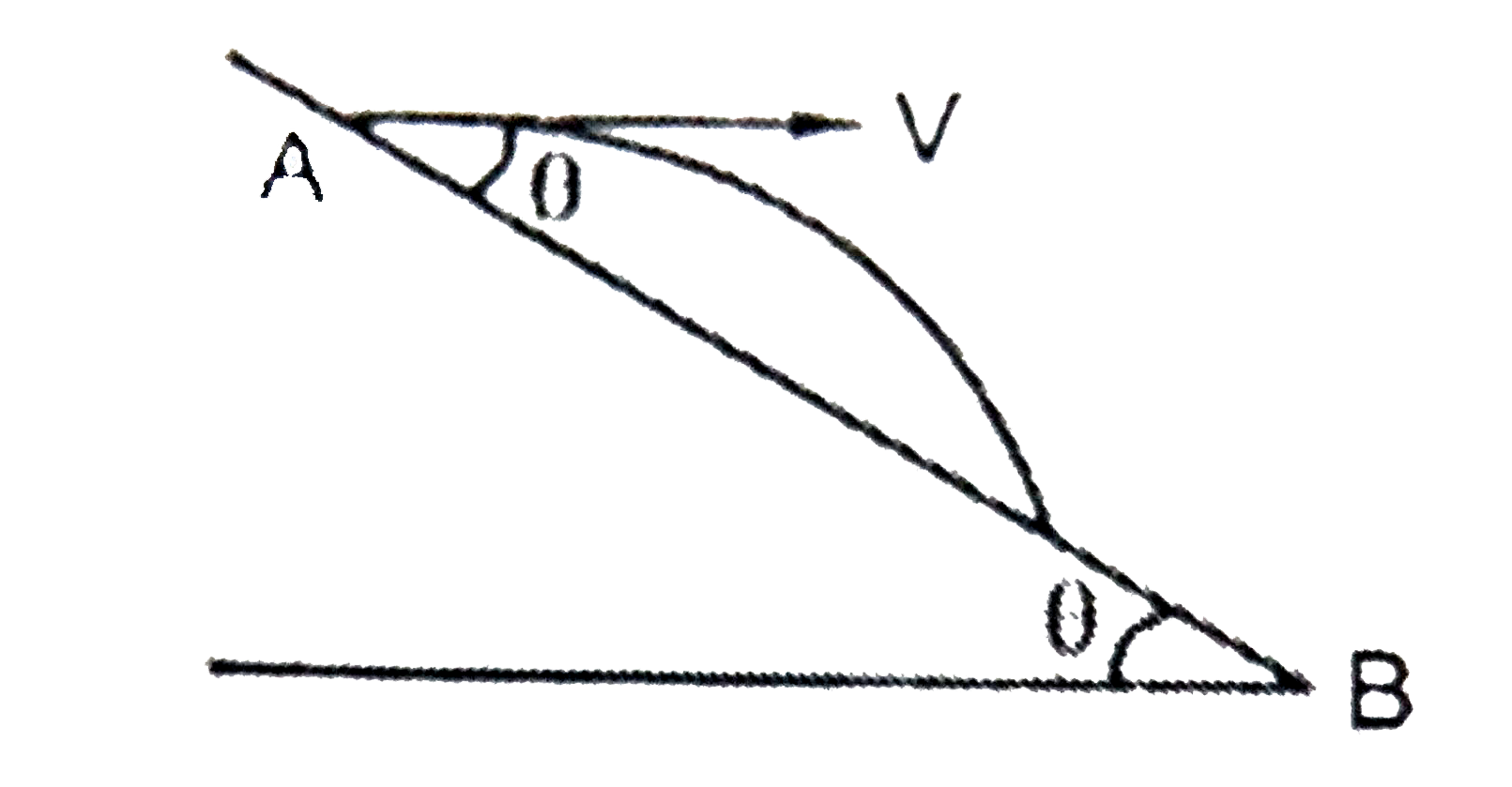
Find the time taken by the particle to hit the plane.
A $\frac{3}{4} s$
B $3 s$
C $4 s$
D $\frac{4}{3} s$

NARAYNA-COLLISION-Multiple Answer

Home
Ask Doubt
Profile