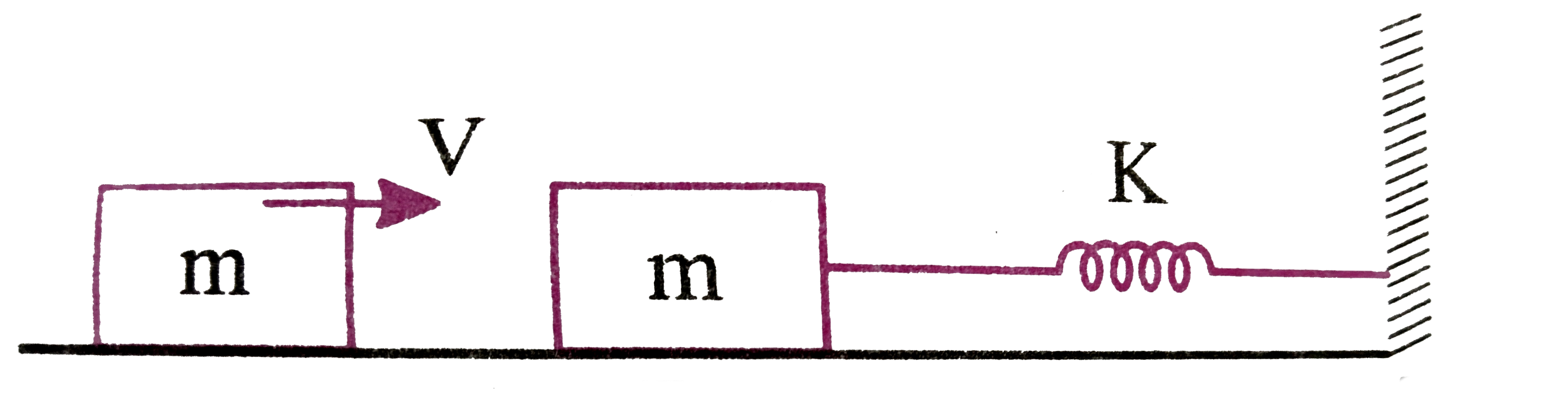
A body A of mass moving with velocity v while passing through its mean position collides in perfect inelastically with a body B of same mass which is connected to a vertical wall through a spring whose spring constant is k. After collision it sticks to B and executes S.H.M. Find the amplitude of reulting motion:
√mvv
√m2kv
mk√v
m2k√v
The correct Answer is:B
Assuming the collision lests for a small interval. We can apply conservation of momentum and get the common velocity = v2K.E.=12(2m)(v2)2=14mv2
This is also the total energy of system as the spring is unstretched at this moment. If the amplitude is A, total energy =12kA2∴12kA2=14mv2
∴A=√m2k.v
Topper's Solved these Questions
- OSCILLATIONSBOOK - NARAYNACHAPTER - OSCILLATIONSEXERCISE - Passage-I
2 Videos
- OSCILLATIONSBOOK - NARAYNACHAPTER - OSCILLATIONSEXERCISE - Passage
33 Videos
- OSCILLATIONSBOOK - NARAYNACHAPTER - OSCILLATIONSEXERCISE - LEVEL-V
1 Videos
- NEWTONS LAWS OF MOTIONBOOK - NARAYNACHAPTER - NEWTONS LAWS OF MOTIONEXERCISE - PASSAGE TYPE QUESTION
6 Videos
- PHYSICAL WORLDBOOK - NARAYNACHAPTER - PHYSICAL WORLDEXERCISE - C.U.Q
10 Videos
Similar Questions
A body of mass m moving with velocity v collides head-on elastically with another body of mass 2m which is initially at rest. The ratio of K. E. of mass m before and after the collision will be
View Solution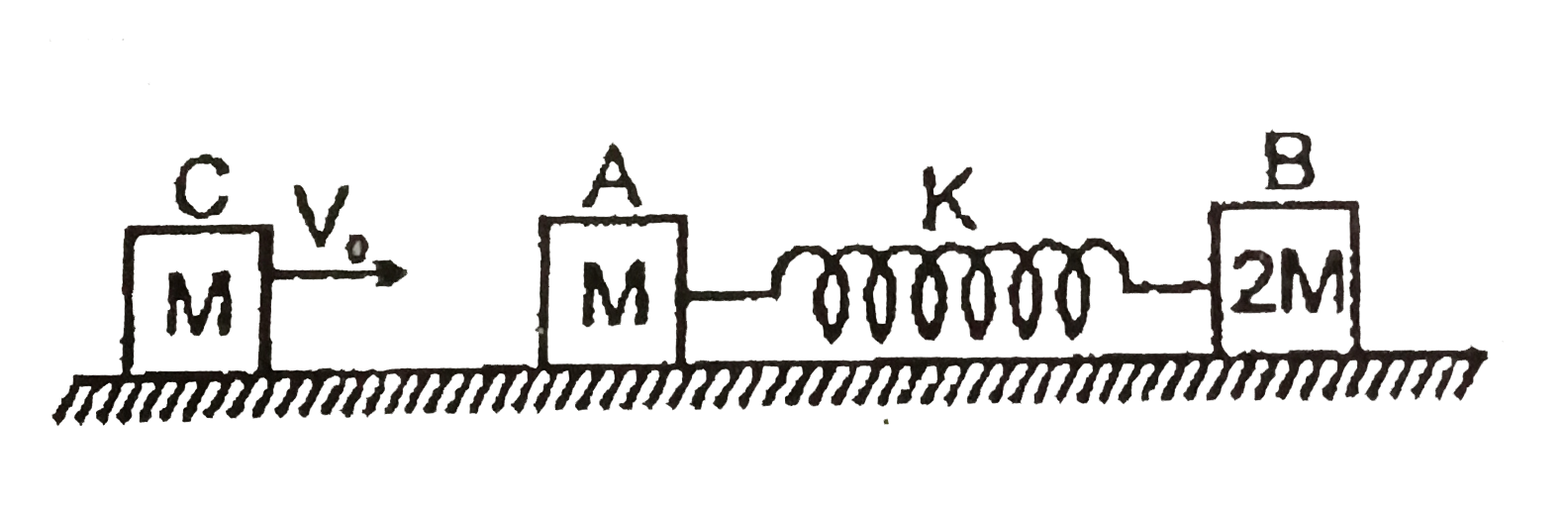 Block C of mass M is moving with velocity V0 and collides elastically with block A of mass M and connected to another block B of mass 2M through a spring of spring constant K. What is K if X0 is the compression of spring when velocity of A and B is same
Block C of mass M is moving with velocity V0 and collides elastically with block A of mass M and connected to another block B of mass 2M through a spring of spring constant K. What is K if X0 is the compression of spring when velocity of A and B is same
View Solution
Knowledge Check
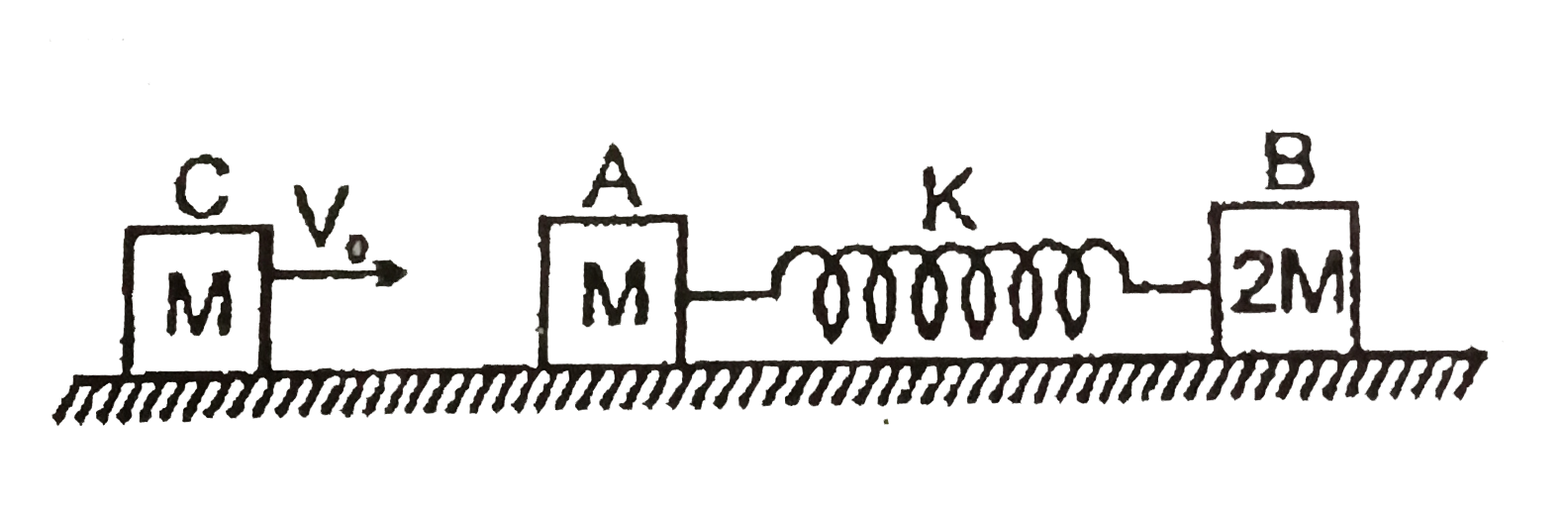 Block C of mass M is moving with velocity V0 and collides elastically with block A of mass M and connected to another block B of mass 2M through a spring of spring constant K. What is K if X0 is the compression of spring when velocity of A and B is same A(MV20X20)B(MV202X20)C(3MV202X20)D(2MV203X20)
Block C of mass M is moving with velocity V0 and collides elastically with block A of mass M and connected to another block B of mass 2M through a spring of spring constant K. What is K if X0 is the compression of spring when velocity of A and B is same A(MV20X20)B(MV202X20)C(3MV202X20)D(2MV203X20)A block C of mass m is moving with velocity v0 and collides elastically with block A of mass m and connect to another block B of mass 2m through spring constant k. What is k if x0 is compression of spring when velocity of A and B is same ?
 Amv20x20Bmv202x20C32mv20x20D23mv20x20
Amv20x20Bmv202x20C32mv20x20D23mv20x20A block C of mass m is moving with velocity v0 and collides elastically with block A of mass m and connect to another block B of mass 2m through spring constant k. What is k if x0 is compression of spring when velocity of A and B is same ?
 Amv20x20Bmv202x20C32mv20x20D23mv20x20
Amv20x20Bmv202x20C32mv20x20D23mv20x20
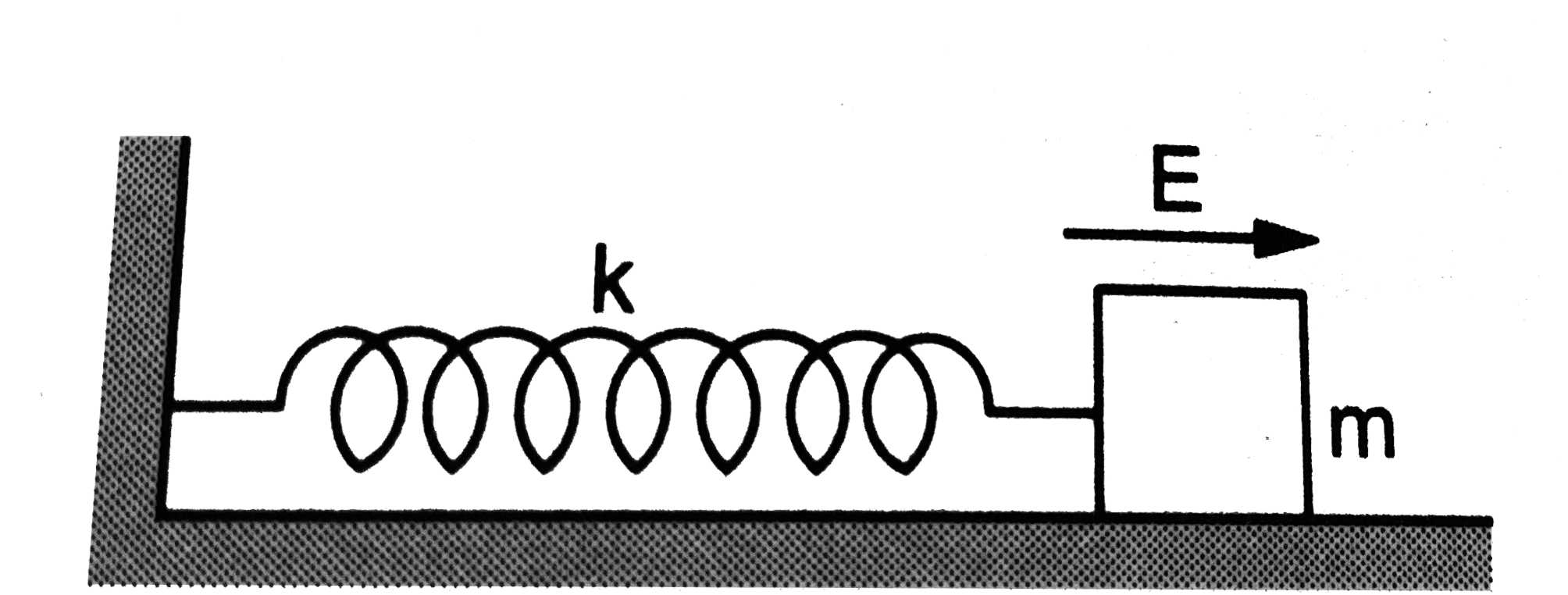
A block of mass m having a charge q is placed on a smooth horizontal table and is connected to a wall through an unstressed spring of spring constant k as shown in figure(29.E1) . A horizontal electric field E parallel to the spring is switched on. Find the amplitude of the resulting SHM of the block.
View SolutionA body is executing SHM with an amplitude of 0.1 m. Its velocity while passing through the mean position is 3ms−1. Its frequency in Hz is
View SolutionA 0.20kg object mass attached to a spring whose spring constant is 500N/m executes simple harmonic motion. If its maximum speed is 5.0m/s, the amplitude of its oscillation is
View SolutionA block of mass 'm' collides perfectly inelastically with another identical block connected to a spring of of force constant 'K'. The amplitude of resulting SHM will be
View SolutionA body of mass m moving with velocity v makes a head-on collision with another body of mass 2 m which is initially at rest. The loss of kinetic energy of the colliding body (mass m ) is
View Solution
