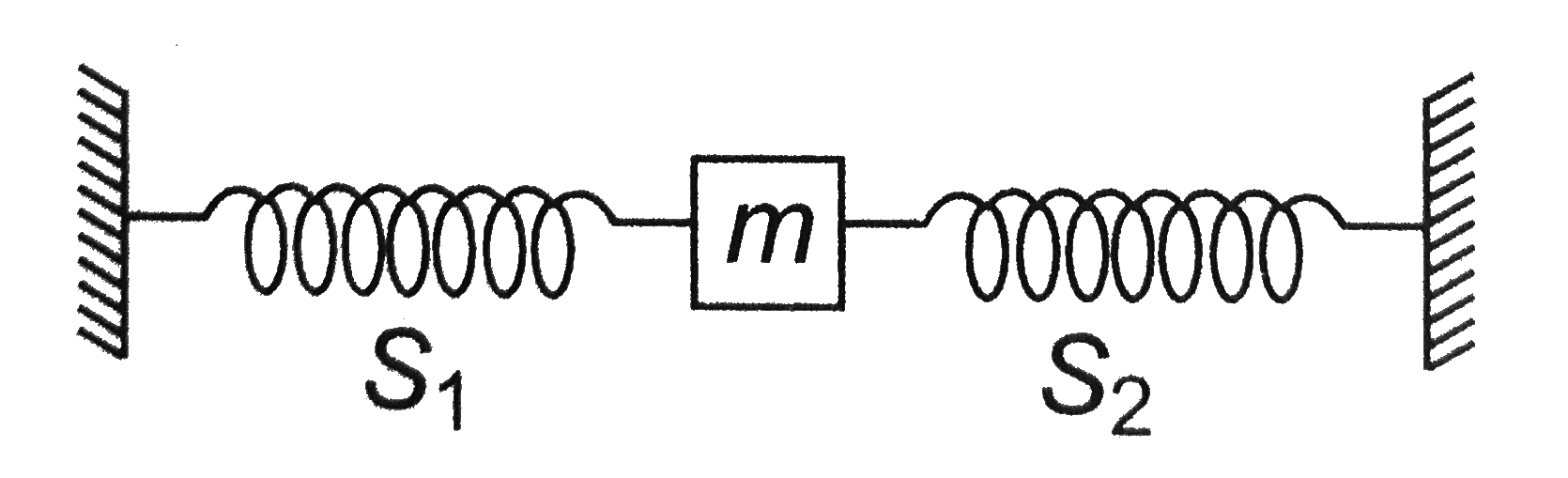
When a mass m is connected individually to two spring S1 and S2 , the oscillation frequencies are v1andv2. If the same mass is attached to the two springs as shwon in figure., the oscillation frequecy would be
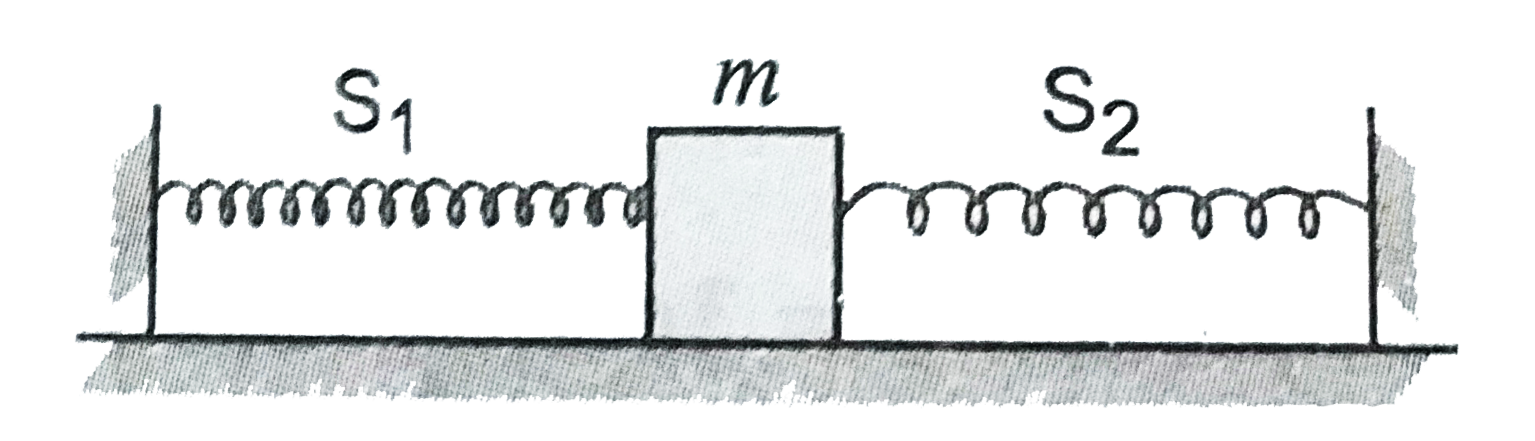
v1+v2
√v21+v22
(1v1+1v2)−1
√v21−v22
The correct Answer is:B
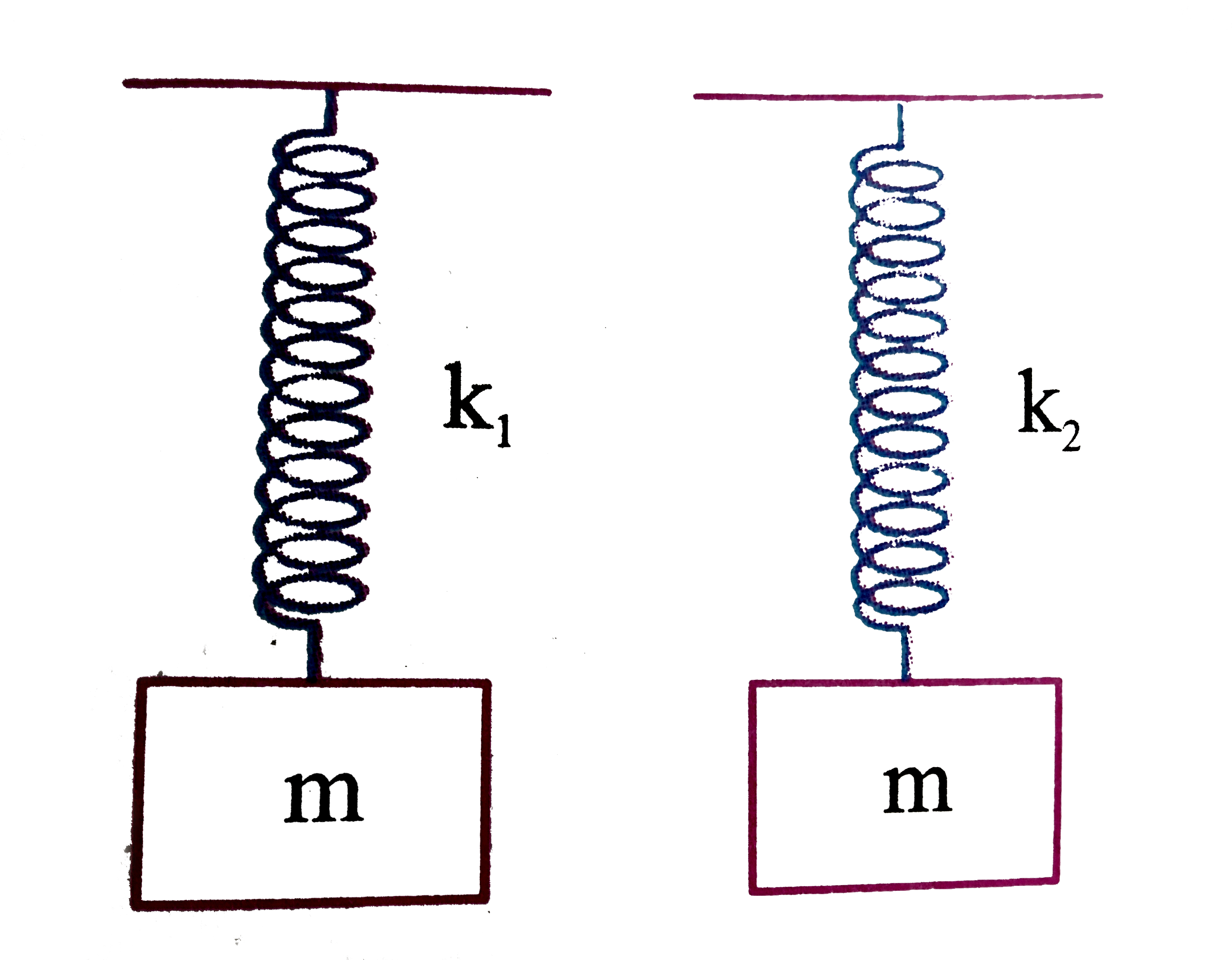
When the mass is connected to the two springs individually,
v1=12π√k1m….(i)
v2=12π√K2m….(ii)
Now the block is connected with two springs considered as parallel.
Here equivalent spring constant,
Keq=k1+k2
Time period of oscillation of the spring block-system is
T=2π√mkeq=2π√mk1+k2
Hence frequency,
v=1T=12π×√k1+k2m
v=12π[k1m+k2m]1/2
From eq. (i) k1m=4π2v21 and from.
(ii), k2m=4πv22
⇒v=12π[4π2v211+4π2v221]1/2
=2π2π[v21+v22]1/2
⇒v=√v21+v22
Topper's Solved these Questions
- OSCILLATIONSBOOK - NARAYNACHAPTER - OSCILLATIONSEXERCISE - Passage-I
2 Videos
- OSCILLATIONSBOOK - NARAYNACHAPTER - OSCILLATIONSEXERCISE - Passage
33 Videos
- OSCILLATIONSBOOK - NARAYNACHAPTER - OSCILLATIONSEXERCISE - LEVEL-V
1 Video
- NEWTONS LAWS OF MOTIONBOOK - NARAYNACHAPTER - NEWTONS LAWS OF MOTIONEXERCISE - PASSAGE TYPE QUESTION
6 Videos
- PHYSICAL WORLDBOOK - NARAYNACHAPTER - PHYSICAL WORLDEXERCISE - C.U.Q
10 Videos
Similar Questions
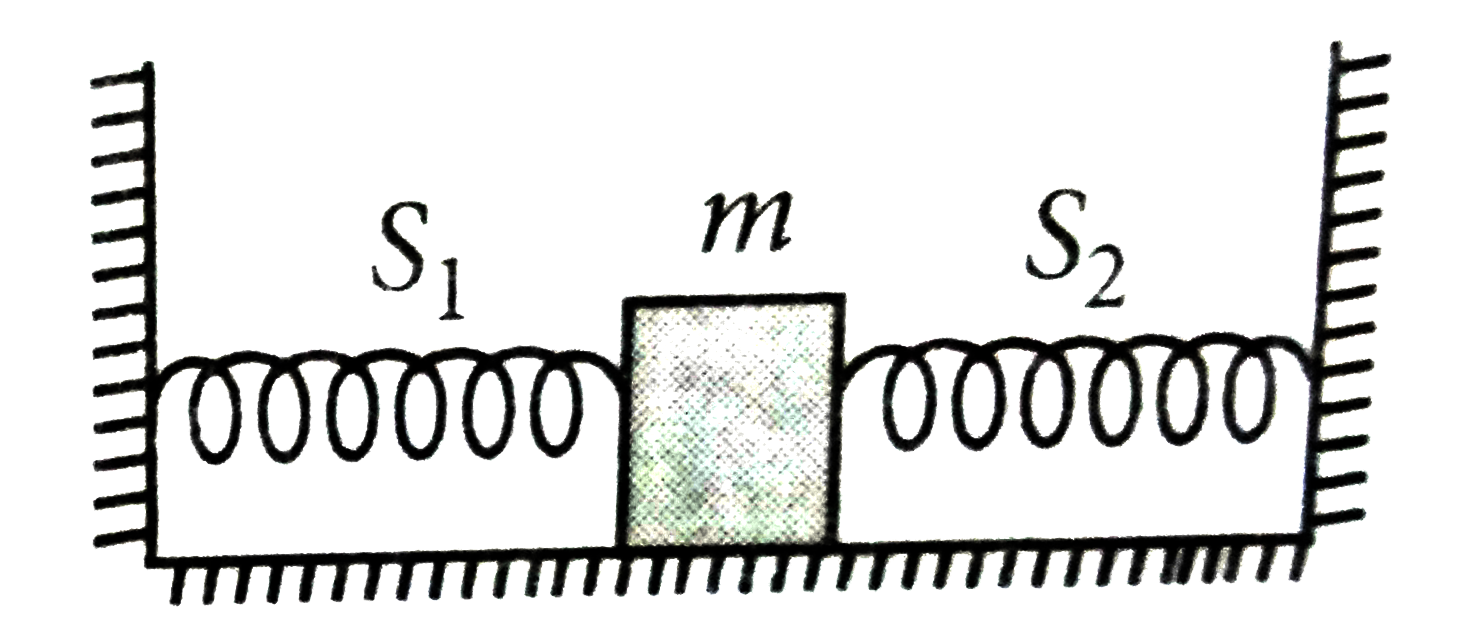
When a mass m is connected individually to two springs S1andS2, the oscillation frequencies are υ1andυ2. If the same mass is attached to the two springs as shown in figure, the oscillation frequency would be
View SolutionA mass is suspended separately by two springs of spring constants k1 and k2 in successive order. The time periods of oscillations in the two cases are T1 and T2 respectively. If the same mass be suspended by connecting the two springs in parallel, (as shown in figure) then the time period of oscillations is T. The correct relations is

View Solution
Knowledge Check
When a mass m is connected individually to two springs S1 and S2, the oscillation frequencies are v1 and v2. If the same mass is attached to the two springs as shown in figure, the oscillation frequency would be
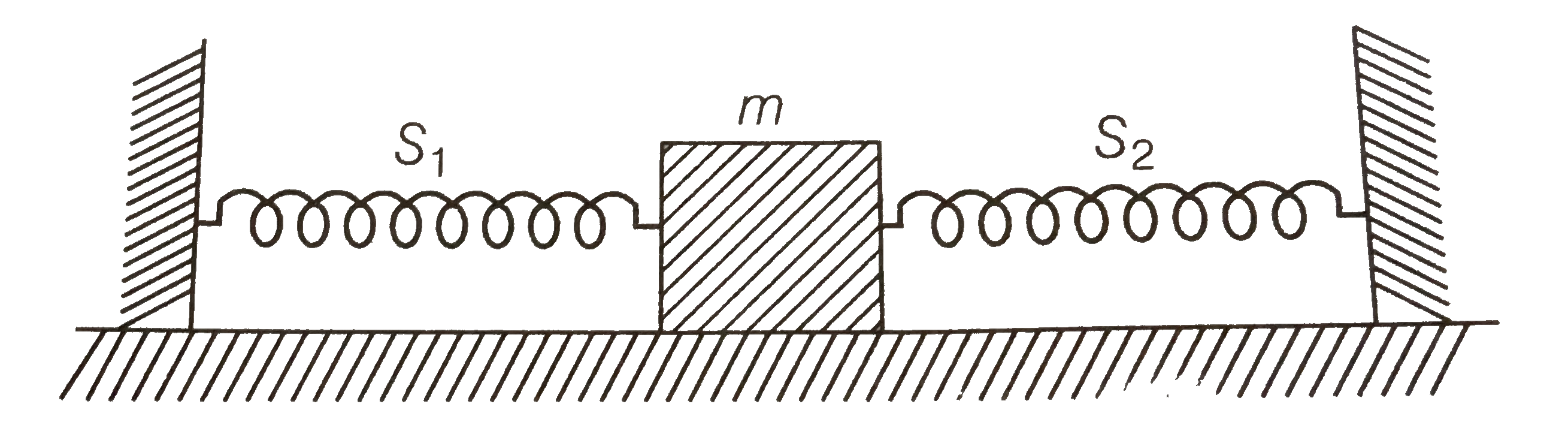 Av1+v2B√v21+v22C(1v1+1v2)−1D√v21−v22
Av1+v2B√v21+v22C(1v1+1v2)−1D√v21−v22Two masses m1 and m2 are attached to a spring balance S as shown in figure. m1>m2 then the reading of spring balance will be .
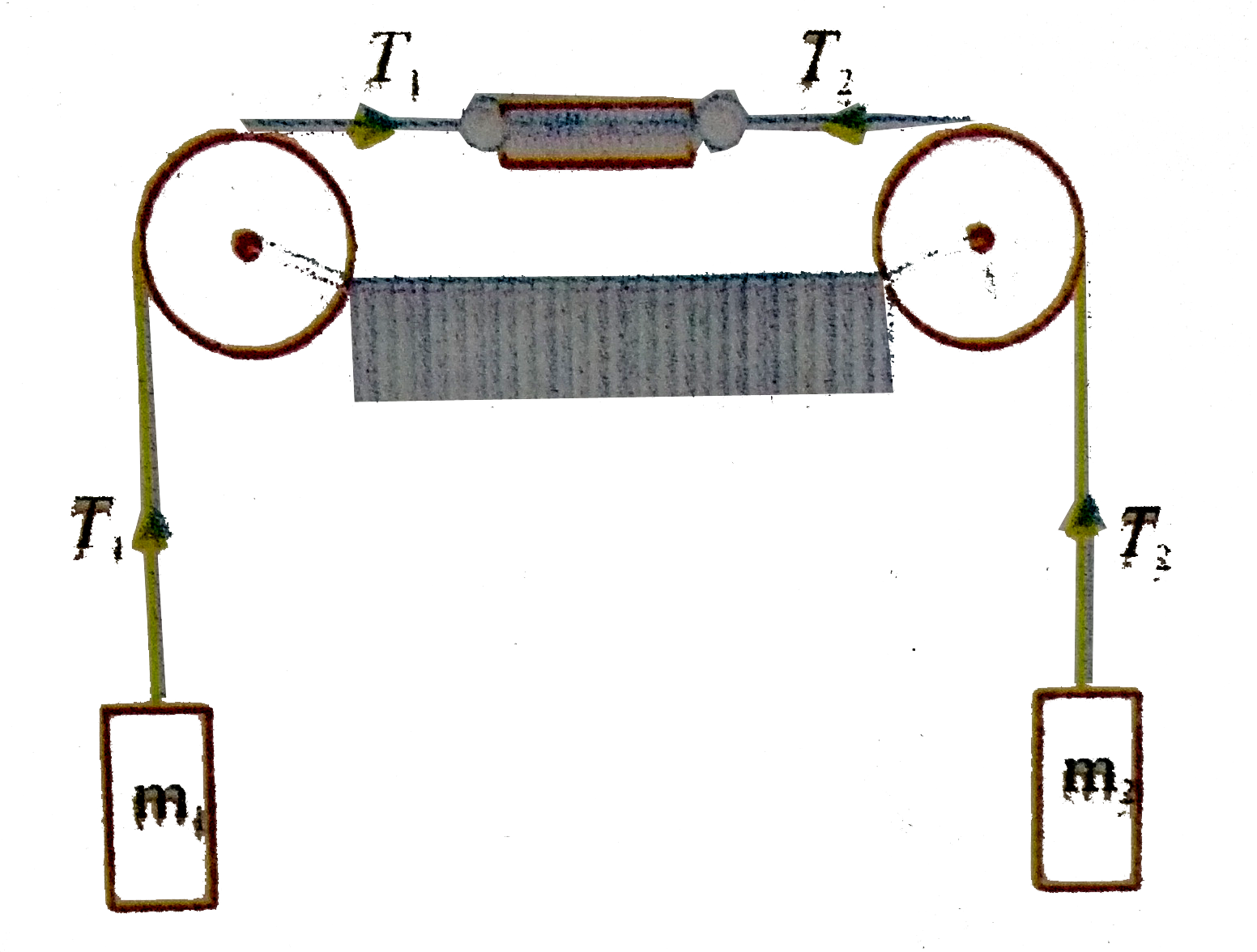 .A(m1−m2)B(m1+m2)C2m1m2m1+m2Dm1m2m1+m2
.A(m1−m2)B(m1+m2)C2m1m2m1+m2Dm1m2m1+m2Two masses m1 and m2 are attached to a spring balance S as shown in Figure. If m1>m2 then the reading of spring balance will be
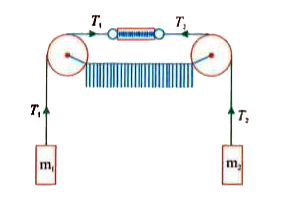 A(m1−m2)B(m1+m2)C2m1m2m1+m2Dm1m2m1+m2
A(m1−m2)B(m1+m2)C2m1m2m1+m2Dm1m2m1+m2
S1andS2 are two identical springs. The oscillation frequency is f. If one springs is removed, frequency will be

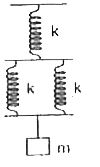
View SolutionA particle of mass m is attached to three identical springs of spring constant k as shwon in figure. The time period of vertical oscillation of the particle is

View SolutionIn the figure S1 and S2 are identical springs. The oscillation frequency of the mass m is f. If one spring is removed, the frequency will become

View SolutionIn figure S1 andS1 are identical springs. The oscillation frequency of the mass m is f. if one spring is removed, the frequency will become
View SolutionCalculate the period of oscillations of block of mass m attached with a set of springs as shown
View Solution
