A sphere of solid material of relative density 9 has a concentric spherical cavity and sinks in water. If te radius of the sphere be R. Then the radius of the cavity (r) will be related to R as
To solve the problem, we need to relate the radius of the cavity (r) to the radius of the sphere (R) given that the sphere sinks in water and has a relative density of 9. Here are the steps to derive the relationship:
Step 1: Understand the Concept of Relative Density
Relative density (RD) is defined as the ratio of the density of a substance to the density of water. For this problem, the relative density of the sphere is given as 9. This means:
Relative Density=Density of Sphere (d)Density of Water(ρw)=9
Thus, we can express the density of the sphere as:
d=9⋅ρw
Step 2: Write the Weight of the Sphere
The weight (W) of the sphere can be expressed as:
W=Volume of Sphere×Density of Sphere×g
The volume of the sphere (with radius R) is:
V=43πR3
So, the weight of the sphere becomes:
W=43πR3⋅d⋅g=43πR3⋅(9ρw)⋅g
Thus,
W=12πR3ρwg
Step 3: Write the Buoyant Force
The buoyant force (B) acting on the sphere when it is submerged in water is given by Archimedes' principle:
B=Volume of Water Displaced×Density of Water×g
The volume of water displaced is equal to the volume of the sphere minus the volume of the cavity:
B=(43πR3−43πr3)⋅ρw⋅g
This simplifies to:
B=43π(R3−r3)ρwg
Step 4: Set Up the Equation for Sinking
Since the sphere sinks, the weight of the sphere equals the buoyant force:
W=B
Substituting the expressions we derived:
12πR3ρwg=43π(R3−r3)ρwg
Step 5: Cancel Common Terms
We can cancel π, ρw, and g from both sides:
12R3=43(R3−r3)
Step 6: Solve for r
Multiply both sides by 3 to eliminate the fraction:
36R3=4(R3−r3)
Expanding the right side:
36R3=4R3−4r3
Rearranging gives:
36R3−4R3=−4r3
32R3=−4r3
Dividing both sides by -4:
−8R3=r3
Thus, we have:
r3=89R3
Step 7: Take the Cube Root
Taking the cube root of both sides gives:
r=(89)1/3R
Final Relation
Thus, we can express the relationship between the radius of the cavity (r) and the radius of the sphere (R) as:
r=23R
Relative density (RD) is defined as the ratio of the density of a substance to the density of water. For this problem, the relative density of the sphere is given as 9. This means:
Relative Density=Density of Sphere (d)Density of Water(ρw)=9
Thus, we can express the density of the sphere as:
d=9⋅ρw
The weight (W) of the sphere can be expressed as:
W=Volume of Sphere×Density of Sphere×g
The volume of the sphere (with radius R) is:
V=43πR3
So, the weight of the sphere becomes:
W=43πR3⋅d⋅g=43πR3⋅(9ρw)⋅g
Thus,
W=12πR3ρwg
The buoyant force (B) acting on the sphere when it is submerged in water is given by Archimedes' principle:
B=Volume of Water Displaced×Density of Water×g
The volume of water displaced is equal to the volume of the sphere minus the volume of the cavity:
B=(43πR3−43πr3)⋅ρw⋅g
This simplifies to:
B=43π(R3−r3)ρwg
Since the sphere sinks, the weight of the sphere equals the buoyant force:
W=B
Substituting the expressions we derived:
12πR3ρwg=43π(R3−r3)ρwg
We can cancel π, ρw, and g from both sides:
12R3=43(R3−r3)
Multiply both sides by 3 to eliminate the fraction:
36R3=4(R3−r3)
Expanding the right side:
36R3=4R3−4r3
Rearranging gives:
36R3−4R3=−4r3
32R3=−4r3
Dividing both sides by -4:
−8R3=r3
Thus, we have:
r3=89R3
Taking the cube root of both sides gives:
r=(89)1/3R
Thus, we can express the relationship between the radius of the cavity (r) and the radius of the sphere (R) as:
r=23R
Topper's Solved these Questions
- MECHANICAL PROPERTIES OF FLUIDSBOOK - NARAYNACHAPTER - MECHANICAL PROPERTIES OF FLUIDSEXERCISE - Illustration
62 Videos
- MECHANICAL PROPERTIES OF FLUIDSBOOK - NARAYNACHAPTER - MECHANICAL PROPERTIES OF FLUIDSEXERCISE - EVALUATE YOURSELF - 1
2 Videos
- MECHANICAL PROPERTIES OF FLUIDSBOOK - NARAYNACHAPTER - MECHANICAL PROPERTIES OF FLUIDSEXERCISE - Level 1 (H.W)
55 Videos
- MATHEMATICAL REVIEW & PHYSICAL WORLDBOOK - NARAYNACHAPTER - MATHEMATICAL REVIEW & PHYSICAL WORLDEXERCISE - C.U.Q
13 Videos
- MECHANICAL PROPERTIES OF SOLIDSBOOK - NARAYNACHAPTER - MECHANICAL PROPERTIES OF SOLIDSEXERCISE - LEVEL-II (H.W)
24 Videos
Similar Questions
A sphere of material of relative density 8 has a concentric spherical cavity and just sinks in water.If the radius of the sphere is 2cm then the volume of the cavity is
View SolutionA sphere of radius R has a uniform volume density ρ. A spherical cavity of radius b, whose center lies at →a, is removed from the sphere.
i. Find the electric field at any point inside the spherical cavity.
ii. Find the electric field outside the cavity
(a) at points inside the large sphere but outside the cavity and
(b) at points outside the large sphere.

View Solution
Knowledge Check
A sphere of solid material of specific gravity 8 has a concentric spherical cavity and just sinks in water. The ratio of radius of cavity to that of outer radius of the sphere must be
A71/32B51/32C91/32D31/32A sphere of radius R and made of material of relative density σ has a concentric cavit of radius r. It just floats when placed in a tank full of water. The value of the ratio R/r will be
A(σσ−1)1/3B(σ−1σ)1/3C(σ+1σ)1/3D(σ−1σ+1)1/3A hemi-spherical cavity is created in solid sphere (of radius 2R) as shown in the figure. Then
 Axcm=−R/15Bycm=−R40Czcm=zeroDNone of these
Axcm=−R/15Bycm=−R40Czcm=zeroDNone of these
A fixed sphere of radius R and uniform density ρ has a spherical cavity of radius R/2 such that the surface of the cavity passes through the centre of the sphere. A particle of mass m is located at the centre (A) of the velocity. Calculated
(a) The gravitational field at A.
(b) The velocity with which the particle strikes the centre O of the sphere. (Neglect earth's gravity)
View SolutionCharge Q is distributed on two concentric spheres of radius r and R resp. if charge density of both spheres is same then electric potential at centre will be
View SolutionA glass sphere of radius 2R and refractive index n has a spherical cavity of radius R, concentric with it.

Q. When viewer is ono left side of the hollow sphere, what will be the shift in position of the object?
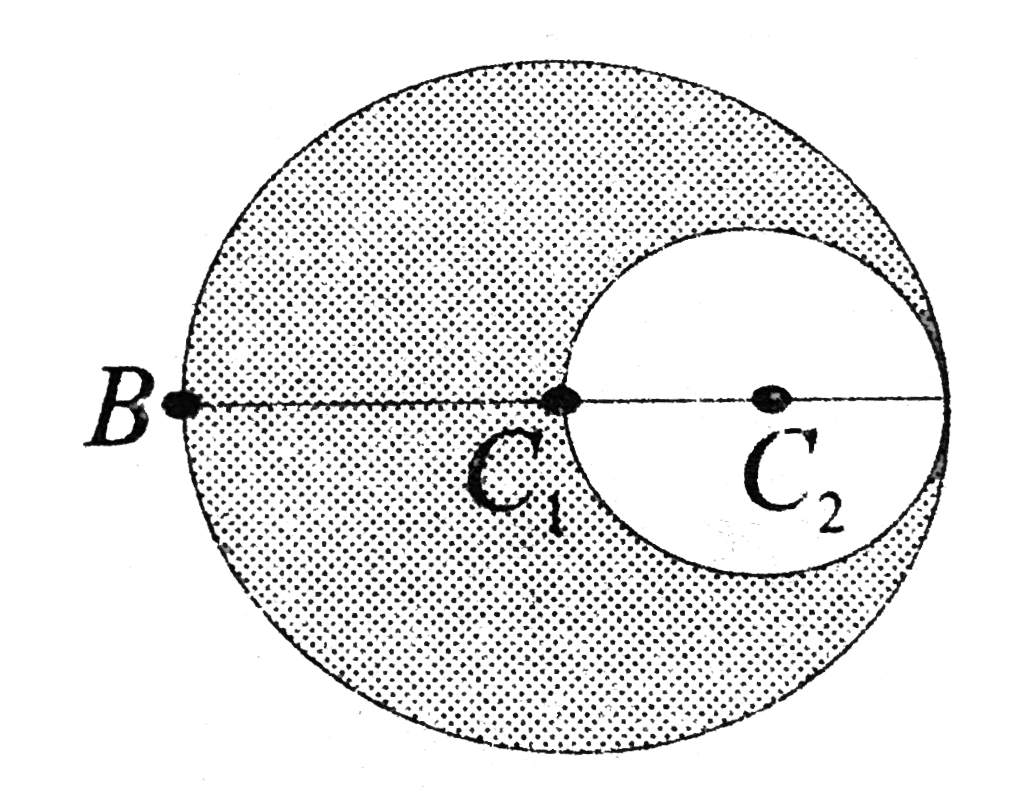
View SolutionA positively charge sphere of radius r0 carries a volume charge density ρ. A spherical cavity of radius r0/2 is then scooped out and left empty. C1 is the center of the sphere and C2 that of the cavity. What is the direction and magnitude of the electric field at point B?
View SolutionA solid metallic sphere has a charge +3Q. Concentric with this sphere is a conducting spherical shell having charge −Q. The radius of the sphere is a and that of the spherical shell is b(b>a) What is the electric field at a distance R(a<R<b) from the centre
View Solution
