Maximum excess pressure inside a thin-walled steel tube of radius r and thickness △r(<<r), so that the tube would not rupture would be (breaking stress of steel is σmax
σmax×rΔr
σmax×Δrr
σmax
σmax×2Δrr
The correct Answer is:B
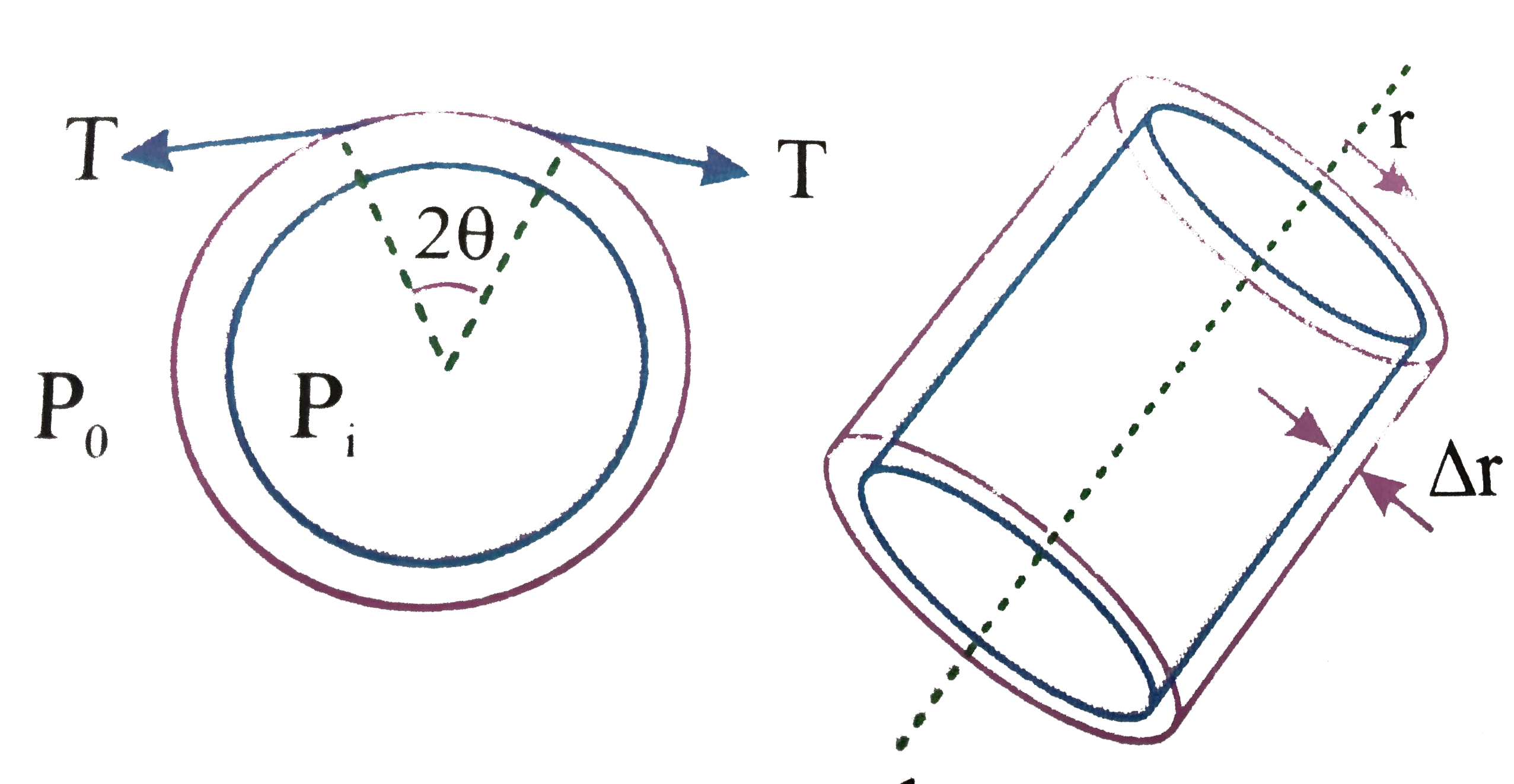
Consider a small linerar element fo the tube, which subtends an angle 2θ at centre as shwon in figure.
2Tsinθ=ΔP×A where ΔP=P1−P0 and A is the area of element.
As θ is very small, sinθ≈θ
So, 2T×θ=ΔP×l×(2rθ)
⇒ΔP=Tlr
σ (Stress develop in tube) =TΔr×l where Δr× is CSA
σ=ΔP×lrΔr×L=ΔP×rΔrL
For no rupturing, σ=σmaximum
SO ΔP×rΔr≤σmaximum
ΔP∣ (maximum value)(=σmax imum×Δrr)
Topper's Solved these Questions
- MECHANICAL PROPERTIES OF SOLIDSBOOK - NARAYNACHAPTER - MECHANICAL PROPERTIES OF SOLIDSEXERCISE - Comprehension-1:
2 Videos
- MECHANICAL PROPERTIES OF SOLIDSBOOK - NARAYNACHAPTER - MECHANICAL PROPERTIES OF SOLIDSEXERCISE - Comprehension-2:
2 Videos
- MECHANICAL PROPERTIES OF SOLIDSBOOK - NARAYNACHAPTER - MECHANICAL PROPERTIES OF SOLIDSEXERCISE - COMPREHENSION TYPE
11 Videos
- MECHANICAL PROPERTIES OF FLUIDSBOOK - NARAYNACHAPTER - MECHANICAL PROPERTIES OF FLUIDSEXERCISE - EXERCISE - III
30 Videos
- MOTION IN A PLANEBOOK - NARAYNACHAPTER - MOTION IN A PLANEEXERCISE - Level-II(H.W)
31 Videos
Similar Questions
The excess pressure inside a thin spherical bubble, of radius R and surface tension T, is △p. What is the work by this outward pressure - developed force during an infinitesimal increase dR in the redius ?
View SolutionA capcitor is made of two circular paltes of radius R each, separated by a distance d<<R. The capacitor is connected to a constant voltage. A thin conducting disc of radius r<<R and thickness t<<r is placed at a center of the bottom plate. Find the minimum voltage required to lift the disc if the mass of the disc si m.
View Solution
Knowledge Check
Maximum excess pressure inside a thin-walled steel tube of radius r and thickness △r(<<r), so that the tube would not rupture would be (breaking stress of steel is σmax
Aσmax×r△rBσmax×△rrCσmaxDσmax×△2rrMaximum pressure difference between inner and outer surface of a thin walled circular tube of radius r and thickness Δr(Δr<<r) so that tube won't break is (Breaking stress of the material is σ0)
Aσ0rΔrBΔrσ0rC2σ0rΔDσ0Excess pressure inside a soap bubble of radius r and surface tension T is
A2TrB4TrCT2rDT4r
The excess pressure inside a soap bubble of radius R is (S is the surface tension)
View SolutionThe excess pressure inside a soap bubble P and the radius R of the bubble are related as
View SolutionThe maximum surface charge density of a uniformly charged spherical radius R and wall thickness 't', so that it will not burst apart, is
View SolutionThe excess pressure inside the drop of a liquid of surface tension T and radius of drom r is
View SolutionCurrent flows through a straight, thin-walled tube of radius r. The magnetic field at a distance x from the axis of the tube has magnitude B.
View Solution
