A truncated cone of solid rubber of a mass M is placed verticle. If its linear dimensions are given and Y = Young's modulus of the cone, find the deformation of the cone.
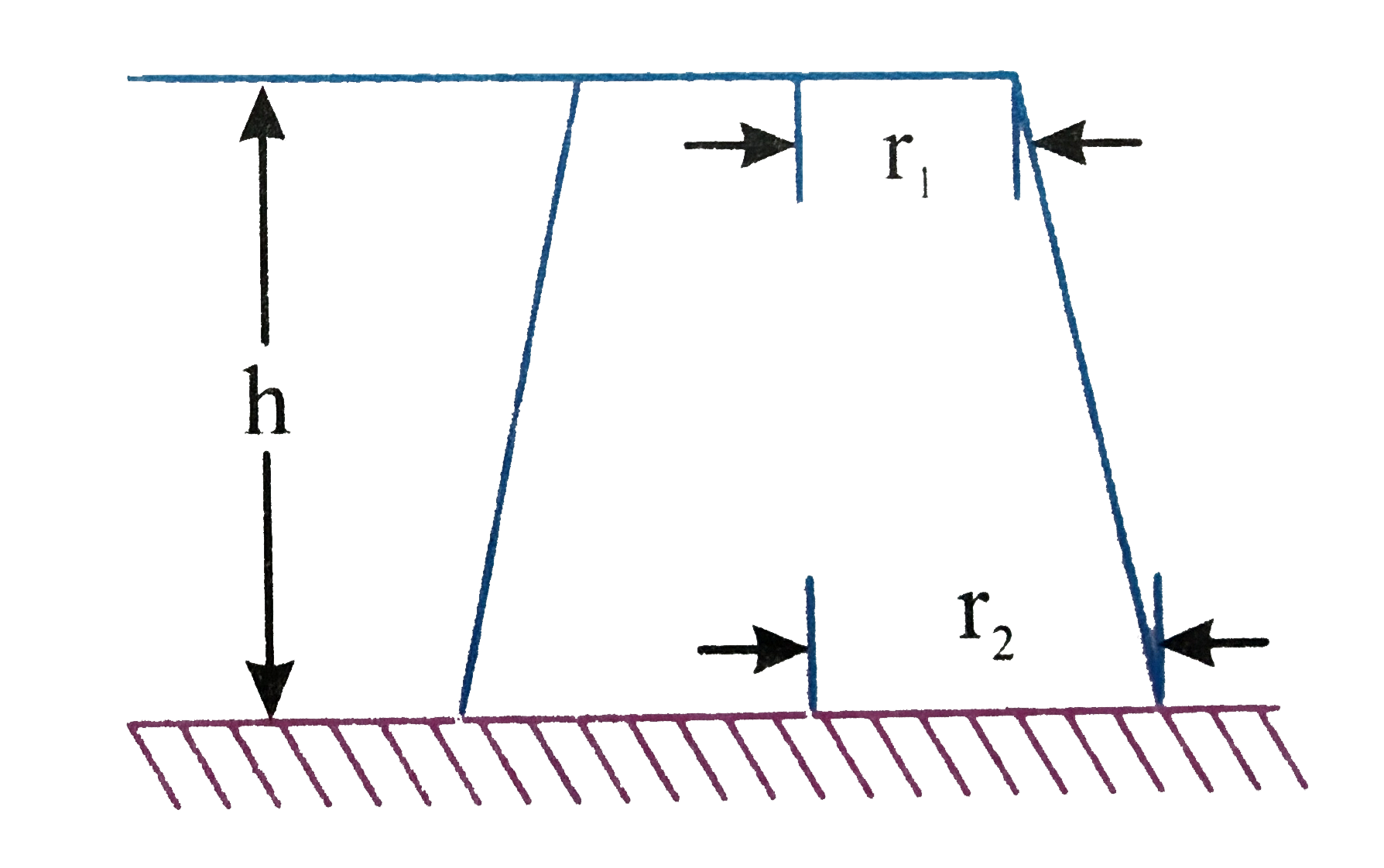
Δl=FH2πr1r2Y
Δl=FH6πr1r2Y
Δl=FH3πr1r2Y
Δl=FHπr1r2Y
The correct Answer is:D
Consider a small elememt at a distance x form the top.
let by the elongation of this elemement
dy=FdxYA,A=πr2
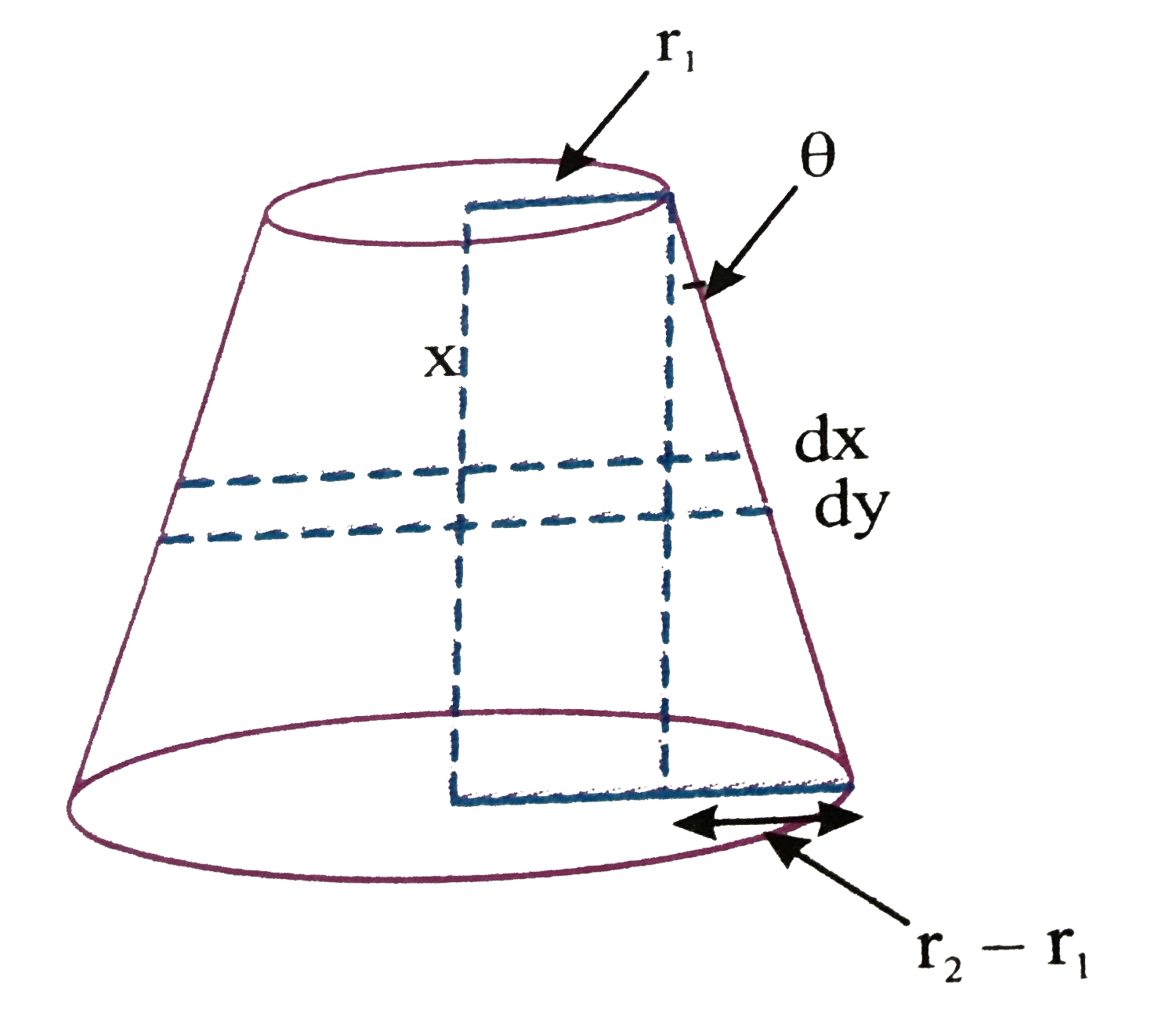
dx = length of that element
F=mg which is constatn
r=a+xtanθ
dy=FYπ×∫H0dx(a+xtanθ)2,tanθ=b−aH
where a=r1,b=r
∴Dl=FHπr1(r1+Htanθ)Y,∴Δl=FHπr1r2Y
Topper's Solved these Questions
- MECHANICAL PROPERTIES OF SOLIDSBOOK - NARAYNACHAPTER - MECHANICAL PROPERTIES OF SOLIDSEXERCISE - Comprehension-1:
2 Videos
- MECHANICAL PROPERTIES OF SOLIDSBOOK - NARAYNACHAPTER - MECHANICAL PROPERTIES OF SOLIDSEXERCISE - Comprehension-2:
2 Videos
- MECHANICAL PROPERTIES OF SOLIDSBOOK - NARAYNACHAPTER - MECHANICAL PROPERTIES OF SOLIDSEXERCISE - COMPREHENSION TYPE
11 Videos
- MECHANICAL PROPERTIES OF FLUIDSBOOK - NARAYNACHAPTER - MECHANICAL PROPERTIES OF FLUIDSEXERCISE - EXERCISE - III
30 Videos
- MOTION IN A PLANEBOOK - NARAYNACHAPTER - MOTION IN A PLANEEXERCISE - Level-II(H.W)
31 Videos
Similar Questions
Knowledge Check
A uniform solid right circular cone of base radius R is joined to a uniform solid hemisphere of radius R and of the same density, as shown. The centre of mass of the composite solid lies at the centre of base of the cone. The height of the cone is
 A1.5RB√3RC3RD2√3R
A1.5RB√3RC3RD2√3RA solid right circular cone is cut into two parts at the middle of its height by a plane parallel to its base . The ratio of the volume of the smaller cone to the whole cone is
A1:2B1:4C1:6D1:8
Solid cylinder of brass 8m high and 4m diameter is melted and recast into a cone of diameter 3m. Find the height of the cone.
View SolutionFind the centre of mass of a uniform solid cone.
View SolutionThe radius and height of a cone are in the ratio 3:4. If its volume is 301.44cm3 Find the radius of the cone.
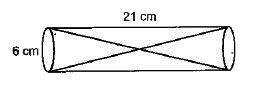
View SolutionTwo solid cones A and B are placed in a cylindrical tube as shown in the given figure. The ratio of their capacities are 2 : 1. Find the heights and capacities of the cones. Also, find the volume of the remaining portion of the cylinder.
View SolutionIf the are of the base of a right circular cone is 3850cm2 and its height is 84 cm, find the slant height of the cone.
View SolutionTwo solid cones A and B are placed in a cylindrical tube as shown in the figure The ratio of their capacities is 2:1 Find the heights and capacities of cones Also find the volume of the remaining portion of the cylinder. 21 cm
View Solution
