A pendulum bob of mass m hangs form a massless elastic string. The potential energy (elastic + gravitational) of the system (bob + string + earth) measured relative to the position of the bob corresponding to the normal length of the string is: (where x = static deformation (elongation) of the string.)
mgx
−12mgx
2mgx
−mgx
The correct Answer is:B
Potential energy of bob w.r.t given refference =−mgx
elastic potential energy of spring =12kx2
=12mgx×x2=12mgx
Hence total potential energy of the system
=−mgx+12mgx=−12mgx.
Topper's Solved these Questions
- MECHANICAL PROPERTIES OF SOLIDSBook:NARAYNAChapter:MECHANICAL PROPERTIES OF SOLIDSExercise:MULTIPLE ANSWER TYPE
Explore 10 Videos
- MECHANICAL PROPERTIES OF SOLIDSBook:NARAYNAChapter:MECHANICAL PROPERTIES OF SOLIDSExercise:COMPREHENSION TYPE
Explore 11 Videos
- MECHANICAL PROPERTIES OF SOLIDSBook:NARAYNAChapter:MECHANICAL PROPERTIES OF SOLIDSExercise:NCERT BASED QUESTIONS
Explore 21 Videos
- MECHANICAL PROPERTIES OF FLUIDSBook:NARAYNAChapter:MECHANICAL PROPERTIES OF FLUIDSExercise:EXERCISE - III
Explore 30 Videos
- MOTION IN A PLANEBook:NARAYNAChapter:MOTION IN A PLANEExercise:Level-II(H.W)
Explore 31 Videos
Similar Questions
The string of a pendulum is horizontal. The mass of the bob is m. Now the string is released. The tension in the string in the lowest position is -
View SolutionA pendulum bob of mass m and length L is released from angle θ with the vertical. Find
(a) the speed of the bob at the bottom of the swing and
(b) tension in the string at that time
View Solution
Knowledge Check
If the string of a pendulum were cut when the bob is at its central position, the bob would fall to the earth due to the absence of the
Aforce of buoyancyBforce of deformationCforce exerted by the string in the downward directionDforce exerted by the string in the upward directionA pendulum bob of mass m charge q is at rest with its string making an angle θ with the vertical in a uniform horizontal electric field E. The tension in the string in
AmgsinθBmgCqEsinθDqEcosθA pendulum bob is hanging from the roof of an elevator with the help of a light string. When the elevator moves up with uniform acceleration 'a' the tension in the string is T1.When the elevator moves down with the same acceleration, the tension in the string is T1 .If the elevator were stationary, the tension in the string would be
AT1+T22B√T1+T2CT1T2T1+T2D2T1T3T1+T2
A bob of mass m hangs from the ceiling of a smooth trolley car which is moving with a constant acceleration a. If young's modulus, radius and length of the string are Y,r and I, respectively, find the (a) stress in the string and (b) extension of the string when it makes a constant angle relative to vertical.
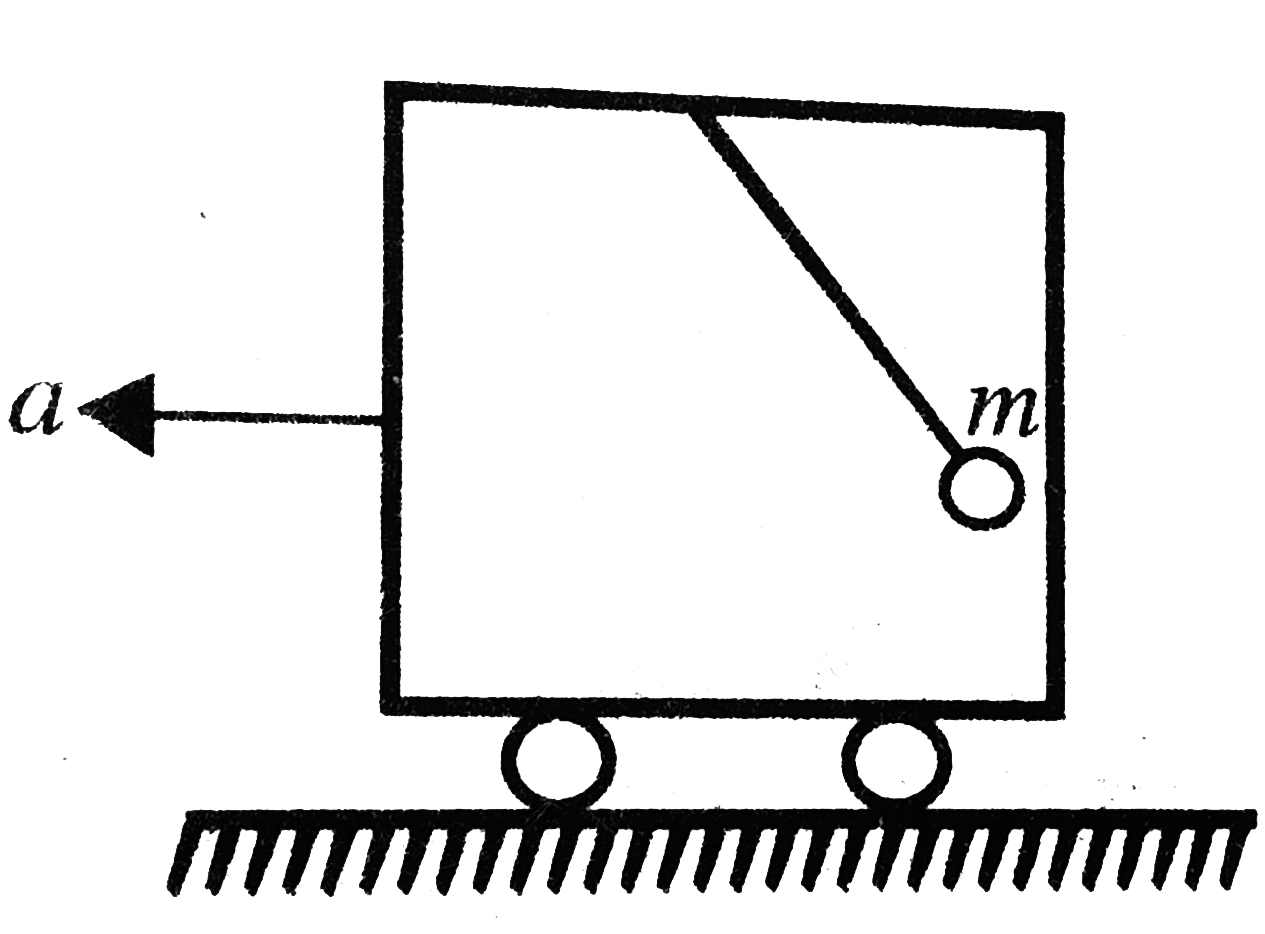
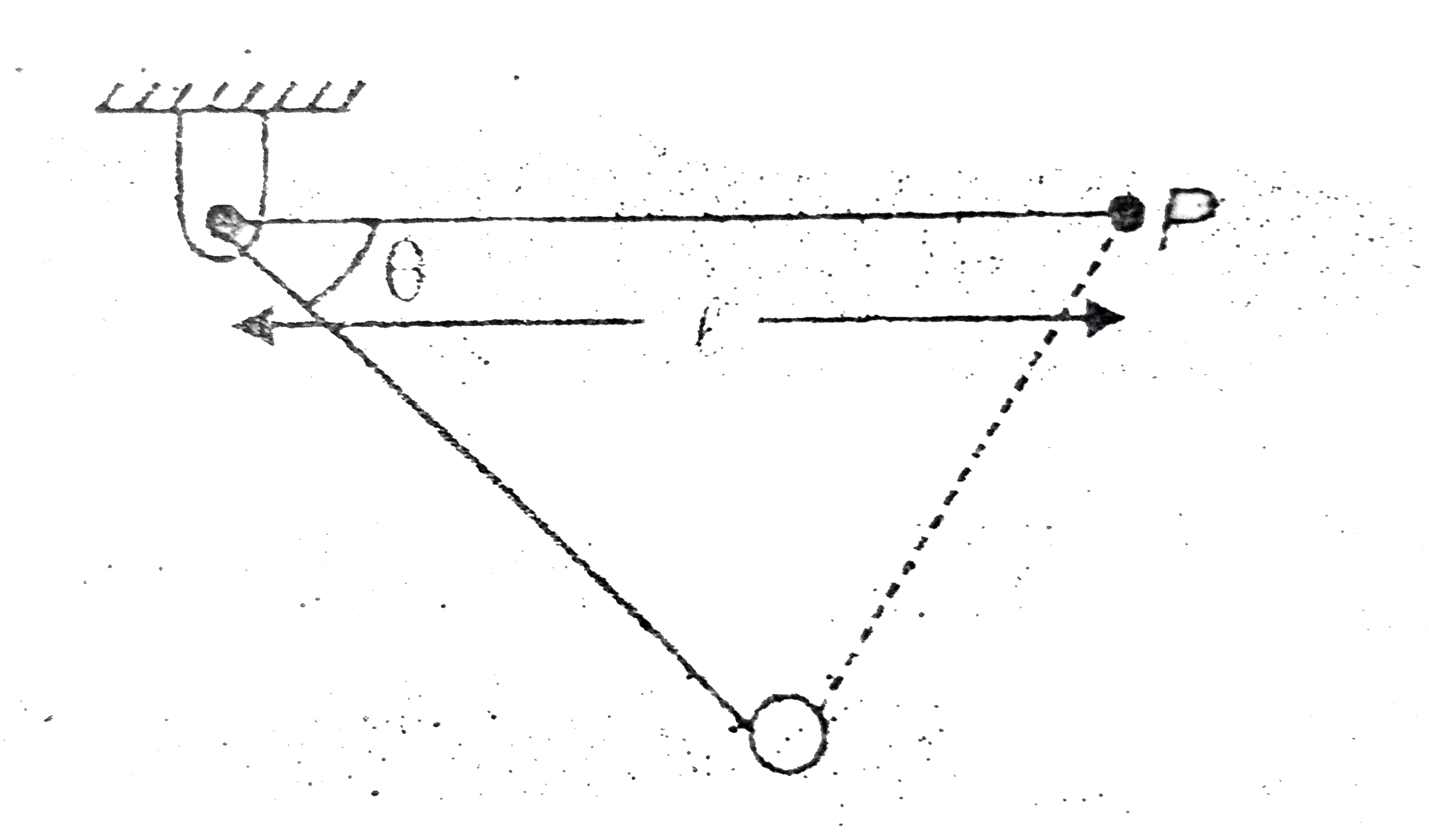
View SolutionA pendulum bob swing from the point P when the ideal string of length l is horizontal. Find the
(A) Speed
(B) Acceleration of the bob
(C ) Power delivered by gravity at an angular position θ
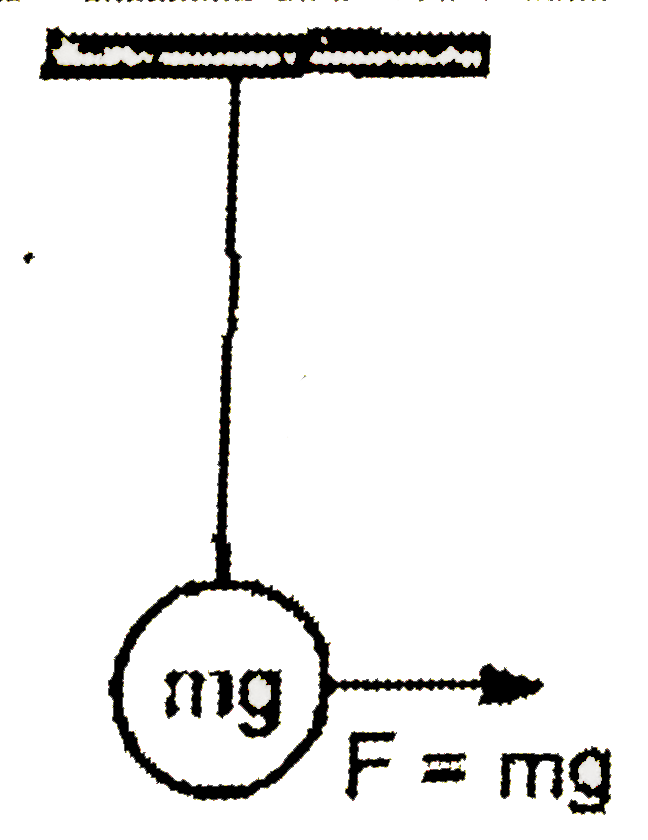
View SolutionA pendulum bob of mass m is suspended at rest. A constant horizontal force F=mg starts acting on it. Find
(a) the maximum angular defection of the string.
(b) the maximum possible tension in the string.
View SolutionA bob of mass 2 kg hangs from a string of length 5 m . It swings from its rest position to one of the sides so that the string makes an angle of 60∘ with the vertical Calculate the gain in potential energy of the bob .
View SolutionA pendulum bob is hanging from the roof of an elevator with the help of a light string. When the elevator moves up with uniform acceleration 'a' the tension in the string is T1. When the elevator moves down with the same acceleration, the tension in the string is T2. If the elevator were stationary, the tension in the string would be
View Solution
