A disc of mass m is connected with an ideal spring of stiffness k. If it is released from rest, it rolls without sliding on an inclination plane. The maximum elongation of the spring is:

mgsinθk
2mgsinθ3k
3mgsinθk
2mgsinθk
The correct Answer is:D
We use work enregy principale
mgsinθ×x−12kx2=0⇒x=2mgsinθk
Topper's Solved these Questions
- SYSTEM OF PARTICLESBook:NARAYNAChapter:SYSTEM OF PARTICLESExercise:Level-VI
Explore 78 Videos
- SYSTEM OF PARTICLESBook:NARAYNAChapter:SYSTEM OF PARTICLESExercise:Level -II(H.W)
Explore 47 Videos
- PHYSICAL WORLDBook:NARAYNAChapter:PHYSICAL WORLDExercise:C.U.Q
Explore 10 Videos
- SYSTEM OF PARTICLES AND ROTATIONAL MOTION Book:NARAYNAChapter:SYSTEM OF PARTICLES AND ROTATIONAL MOTION Exercise:EXERCISE - IV
Explore 39 Videos
Similar Questions
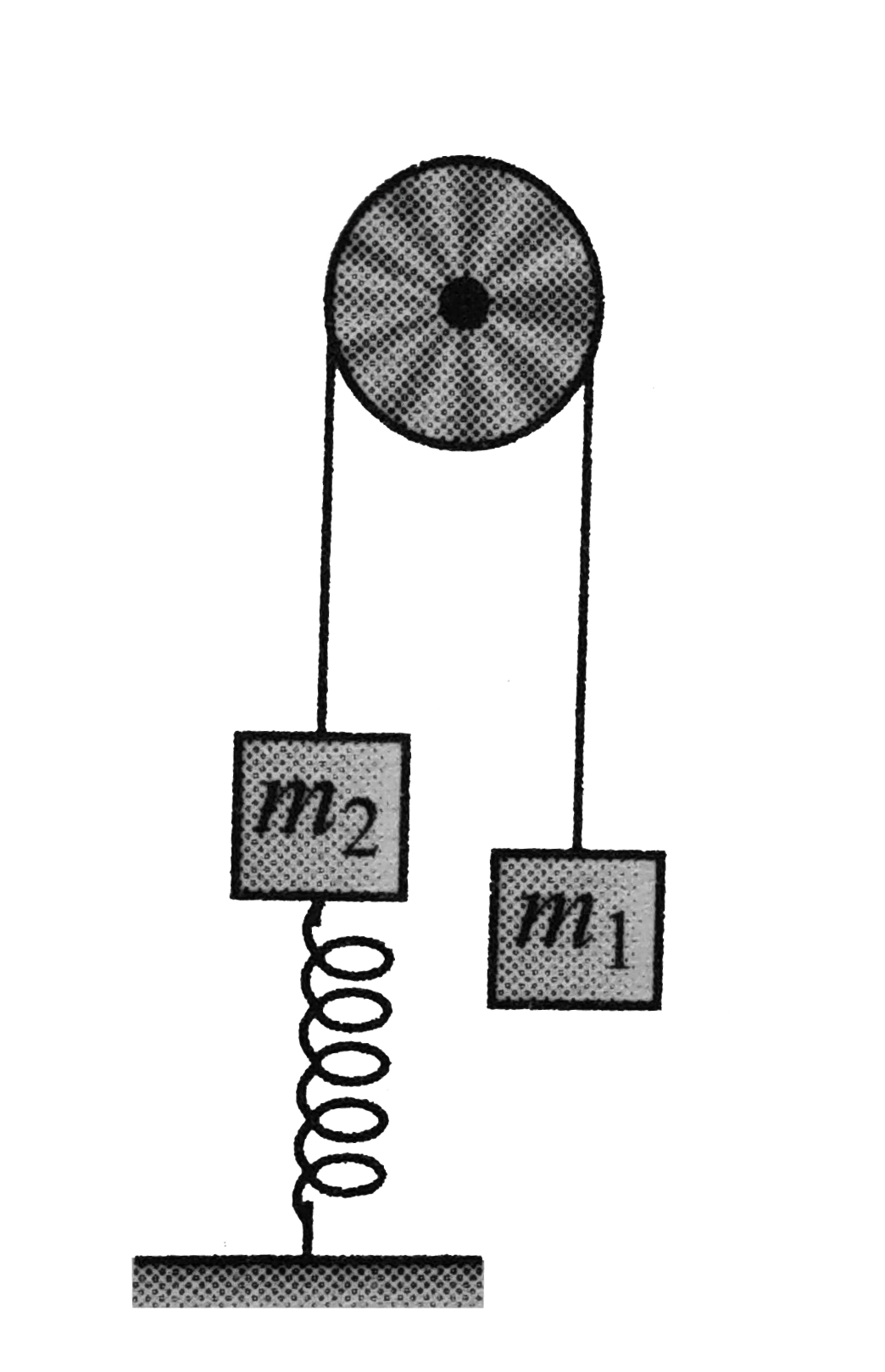
In an ideal pulley particle system, mass m2 is connected with a vertical spring of stiffness k. If mass m2 is released from rest, when the spring is underformed, find the maximum compression of the spring.
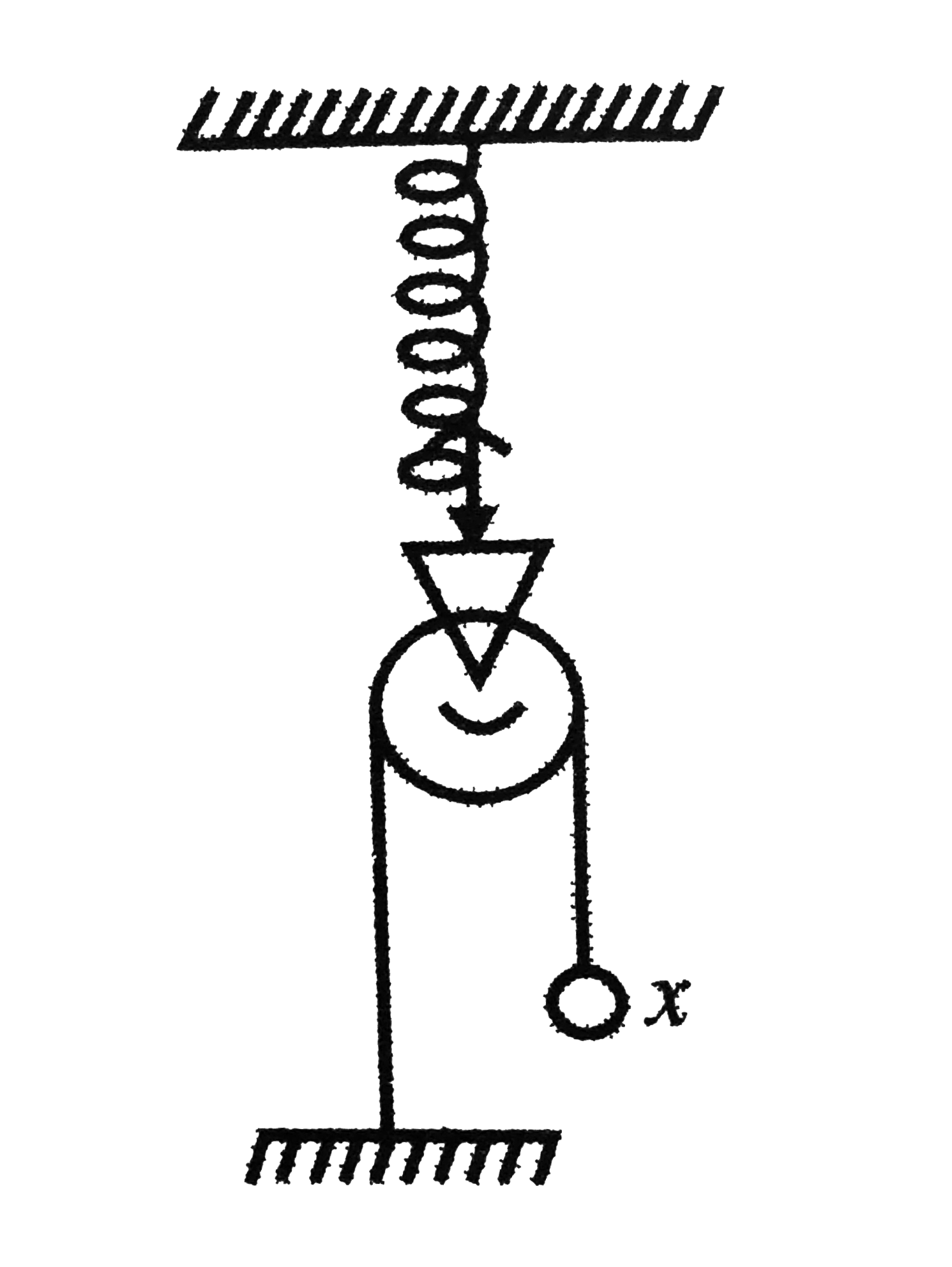
View SolutionA body of mass m hangs by an inextensible string that passes over a smooth mass less pulley that is fitted with a light spring of stiffness k as shown in figure. If the body is released from rest and the spring is released, calculate the maximum elongation of the spring.
View Solution
Knowledge Check
Two thin films of same liquid of surface tension 'T' are formed between a smooth rectangular wire frame and two thin uniform strainght wires each of mass 'm' and length 'I' connected to a massless non-deformed spring of stiffness 'k' and then the system is released form rest. The maximum elongation produced in the spring is
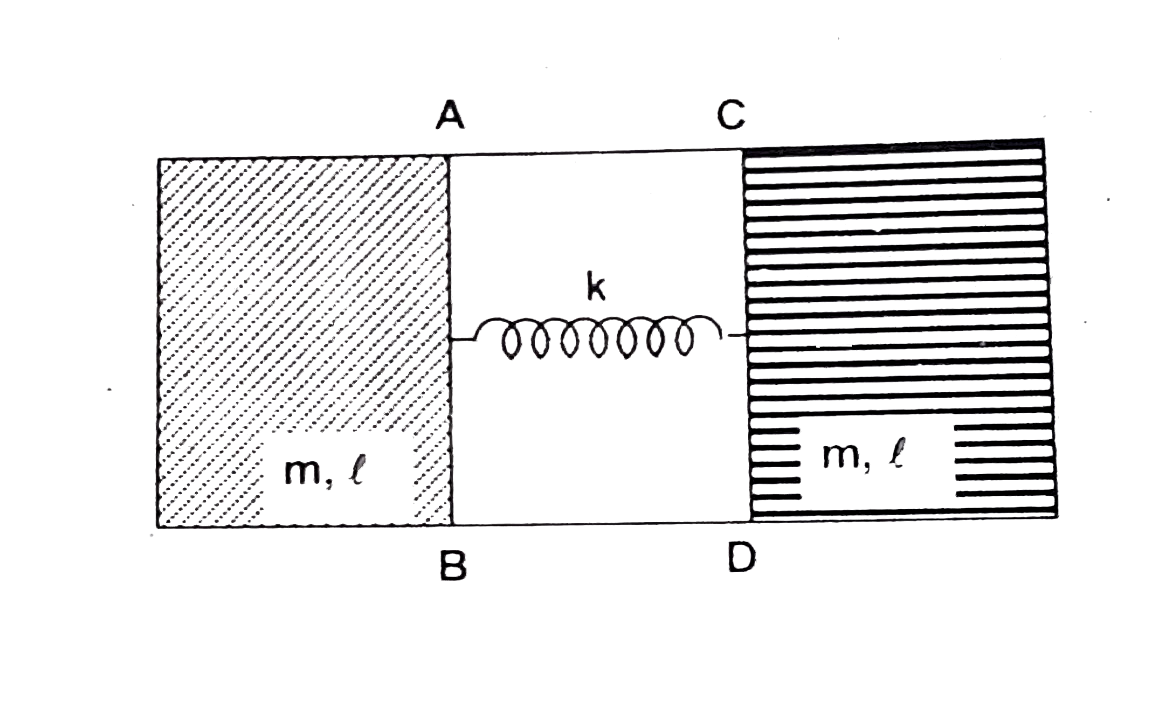 A2TlKB4TlKC6TlKD8TlK
A2TlKB4TlKC6TlKD8TlKA disc of mass m is connected with an ideal spring of spring constant k inside a lift as shown. The left starts moving with constant acceleration (aˆi−aˆj). At the same moment disc starts rolling without sliding on the wall of lift (from rest). If spring is in natural length initially then friction force acting on disc as a function of y is given by : (y is the displacement of the disc with respect to lift)
 A−kyB23(mg+ma−ky)C13(mg+ma−ky)Dmg+ma−2ky
A−kyB23(mg+ma−ky)C13(mg+ma−ky)Dmg+ma−2kyA disc of mass 3 m and a dise of mass m are connected by a massless sping of stiffness k. The heavier is disc placed on the ground with the spring vertical and lighter disc on top from its equilibrium position the upper disc is pushed down by a distance δ and released. Then.
Aif δ>3mgk the lower desc will bounce up.Bif δ=2mgk maximum normal reaction fron on lower disc = 6mg .Cif δ=2mgk maximum normal reaction from ground on lower disc = 4 mg.Dif δ>4mgk, the lower disc will bounce up
The block of mass m is released when the spring was in its natrual length. Spring constant is k. Find the maximum elongation of the spring.

View SolutionA block of mass m connected with a smooth prismatic wedge of mass m is released from rest when the spring is relaxed. Find the angular frequency of oscillation.

View SolutionThe system of the wedge and the block connected by a massless spring as shown in the figure is released with the spring in its natural length. Friction is absent. Maximum elongation in the spring will be
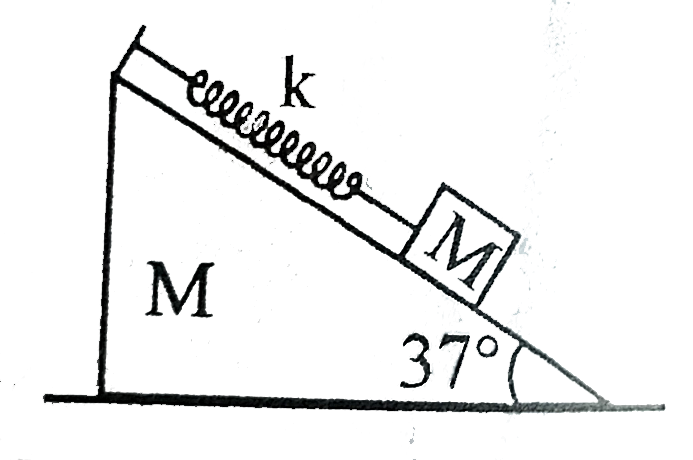
View SolutionA cube of mass m and density D is suspended from a point P by a spring of stiffness k. The system is kept inside a beaker filled with a liquid of density d. The elongation in the spring, assuming D>d, is :

View SolutionA rolling body is connected with a trolley car by a spring of stiffness k. It does not slide and remains in equilibrium relative to the acceleration trolley car. If the trolly car is stopped after a time t=t0. (the rolling body touches the trolly)
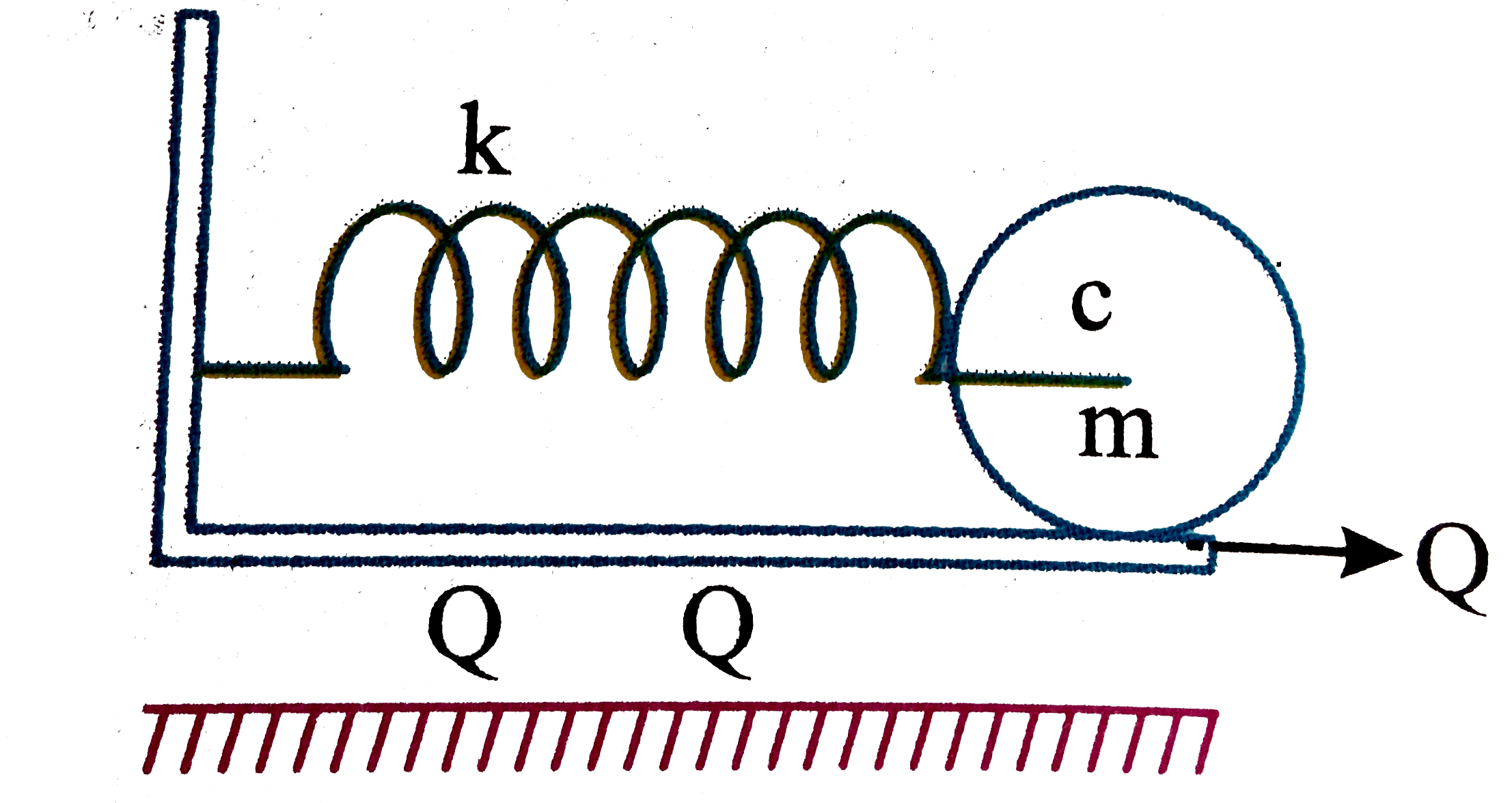
View Solution