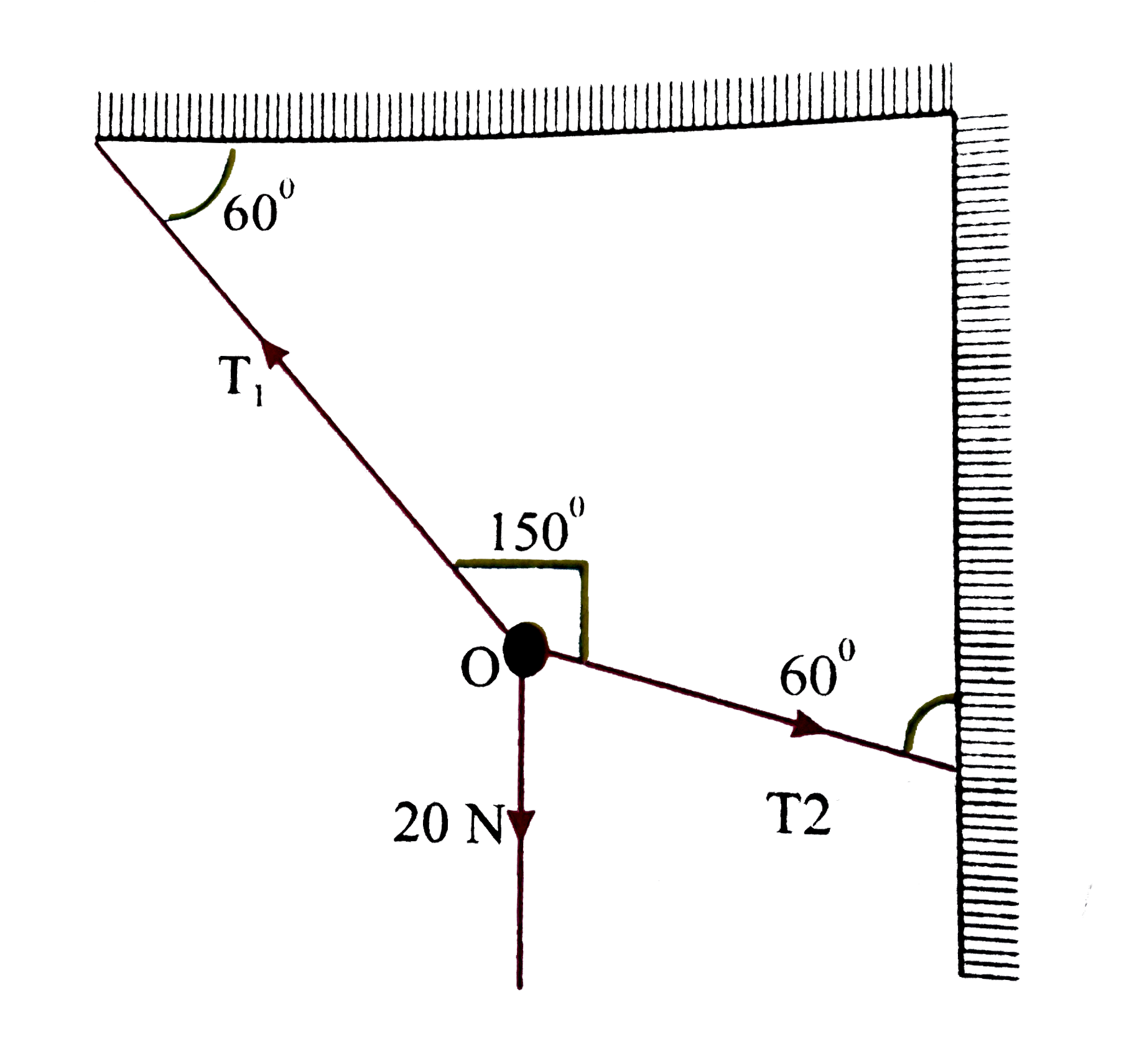
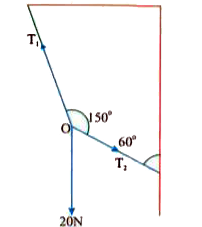
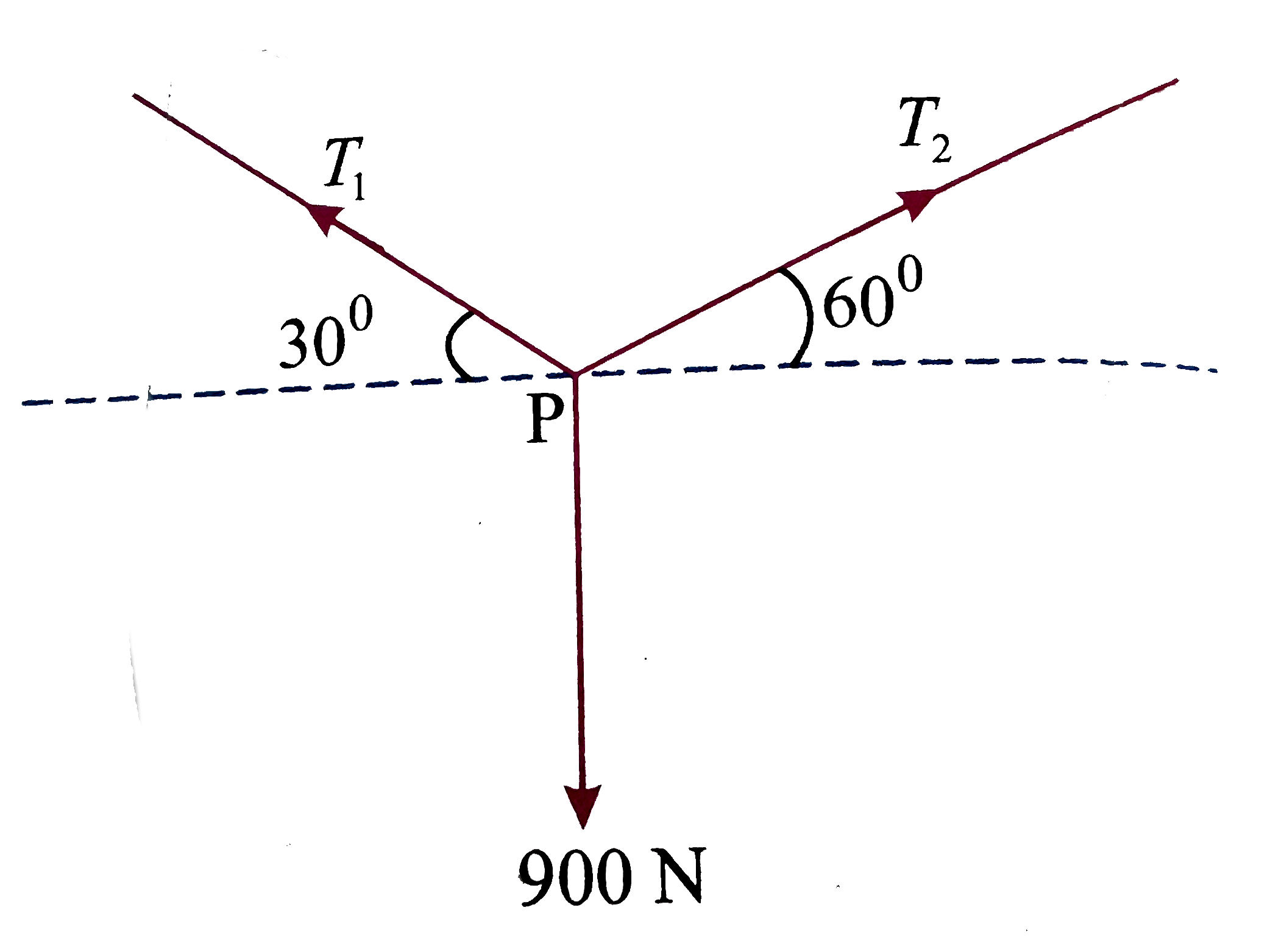
If O is at equilibrium then the values of the tension T1 and T2 are, (20N is acting vertically downwards at O).
20N,30N
20√3N,20N
20√3N,20√3N
10N,30N
The correct Answer is:B
T1sinθ1=T2cosθ2,T1cosθ1=T2sinθ2+mg .
Topper's Solved these Questions
- NEWTONS LAWS OF MOTIONBOOK - NARAYNACHAPTER - NEWTONS LAWS OF MOTIONEXERCISE - LEVEL -III
31 Videos
- NEWTONS LAWS OF MOTIONBOOK - NARAYNACHAPTER - NEWTONS LAWS OF MOTIONEXERCISE - NCERT BASED QUESTION
33 Videos
- NEWTONS LAWS OF MOTIONBOOK - NARAYNACHAPTER - NEWTONS LAWS OF MOTIONEXERCISE - LEVEL -I (C.W)
47 Videos
- MOTION IN A STRAIGHT LINEBOOK - NARAYNACHAPTER - MOTION IN A STRAIGHT LINEEXERCISE - Level 2 H.W
29 Videos
- OSCILLATIONSBOOK - NARAYNACHAPTER - OSCILLATIONSEXERCISE - EXERCISE - IV
41 Videos
Similar Questions
Knowledge Check
If 'O' is at equilibrium then the values of the tension T1 and T2 respectively.
 A20N, 30 NB20√3N,20NC20√3N,20√3ND10N, 30 N
A20N, 30 NB20√3N,20NC20√3N,20√3ND10N, 30 NIf P is in equilibrium then T1T2 is
 A√3B2C1√3D12
A√3B2C1√3D12If the arrangement in fig is given a downward acceleration then the ratio of tensions T1 and T2 is strings is-
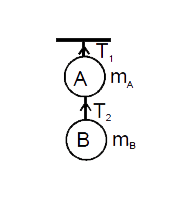 A(mA+mB)/mBB(mA+mB)/mACmA−mBmA+mBDNone of these
A(mA+mB)/mBB(mA+mB)/mACmA−mBmA+mBDNone of these
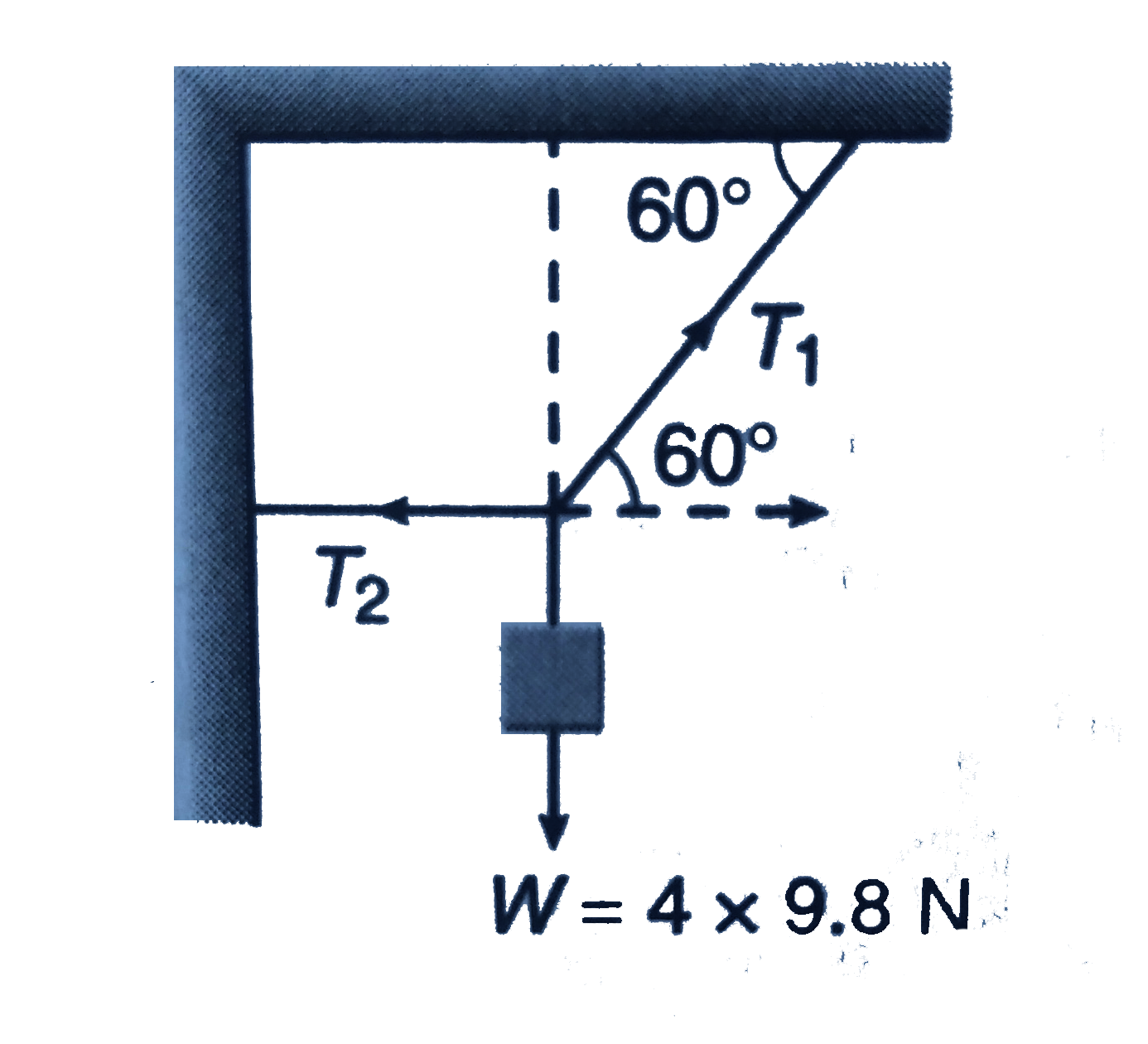
Determine the tension T1 and T2 in the strings shown in Fig.

View SolutionDetermine the tensions T1 and T2 in the string as shown in figure.
View SolutionThe value of tension in a long thin metal wire has been changed from T1 to T2. The lengths of the metal wire at two different values of tension T1 and T2 are l1andl2 respectively. The actual length of the metal wire is :
View SolutionA stone of mass m tied to one end of a string is revolved in a vertical circle of radius R the net forces at the lowest (FL and highest (FH) points of the circle directed vertically downwards are :
(a) FL=mg−T1FH=mg+T2
(b) FL=mg+T1,FH=mg−T2
(c) FL=mg+T1−mυ21R,FH=mg−T2+mυ21R
(d) FL=mg−T1−mυ21R,FH=mg+T2+mυ12R
Choose corret alternative T1,υ1 denote the tension and speed at the lowest point T2,υ2 denote corresponding valuse at the highest point .
View SolutionA body projected vertically upwords from the top of a tower reaches the ground in t1 second . If it projected vertically downwards from the some top with same velocity ,it reaches the ground in t2seconds . If it is just dropped from the top it reaches the ground in t second .prove that t=√t1t2
View Solution