A projectile is thrown into air so as to have the maximum possible range equal to 200. Taking projection point as origin, the coordinates of the point where the velocity of the projectile is minimum, are
(200,50)
(100,50)
(100,50)
(100,100)
The correct Answer is:B
Rmax=u2g=200m
Hmax=u2sin245∘2g=u24g=50m
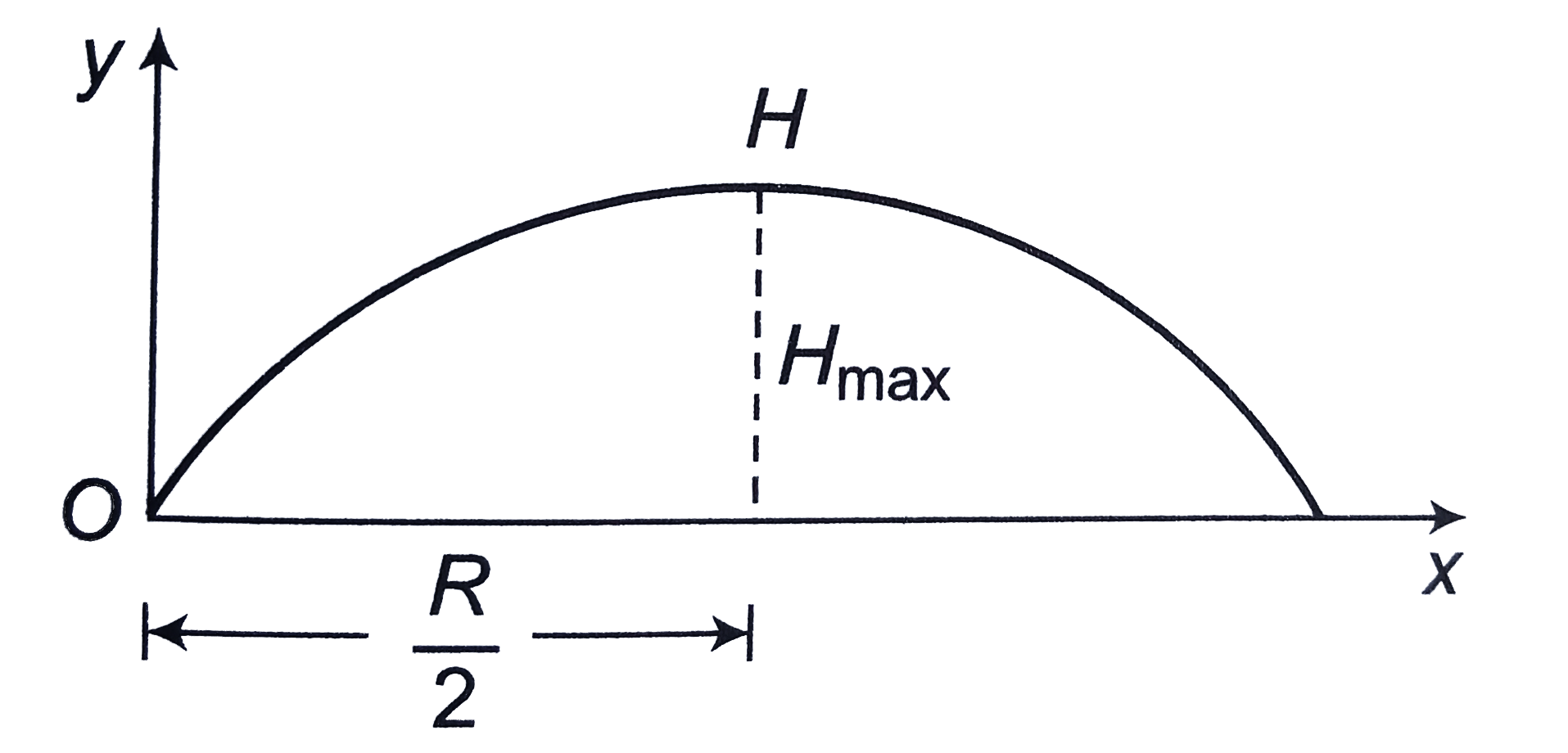
At the highest point H, the velocity is minimum.
Coordinates of H≡(R2,Hmax)
≡(100,50)
Topper's Solved these Questions
Similar Questions
A projectile is thrown into space so as to have maximum horizontal range R. Taking the point of projection as origin, find out the co-ordinates of the point where the speed of the particle is minimum.
View SolutionAt what point in its trajectory does a projectile have its minimum speed ?
View Solution
Knowledge Check
A projectile is thrwon into space so as to have the maximum possible horizontal range equal to 400m. Taking the point of projection as the origin, the coordinates of the point where the velocity of the projectile is minimum are:-
A-400100B-200100C-400200D-200200A projectile is projected with a kinetic energy K. If it has the maximum possible horizontal range, then its kinetic energy at the highest point will be
AK/4BK/2C3K/4DKA projectile is thrown with velocity v at an angle θ with the horizontal. When the projectile is at a height equal to half of the maximum height,.
The velocity of the projectile when it is at a height equal to half of the maximum height is.Av√cos2θ+sin2θ2B√2vcosθC√2vsinθDvtanθsecθ
If the horizontal range of a projectile be a and the maximum height attained by it is b, then prove that the velocity of projection is
View SolutionA projectile can have the same rangeR for two angle of projection . If t−1 and t2 be the terms of flight in the two cased then the initial velocity of projectile is?
View SolutionA javelin thrown into air at an angle with the horizontal has a range of 200 m. If the time of flight is 5 second, then the horizontal component of velocity of the projectile at the highest point of the trajectory is
View SolutionA projectile is thrown with velocity v at an angle θ with the horizontal. When the projectile is at a height equal to half of the maximum height,.
The vertical component of the velocity of projectile is.
View SolutionThe horizontal range and the maximum height of a projectile are equal. The angle of projection of the projectile is
View Solution