A number of indentical cells, n, each of emf ε, internal resistance r, connected in series are charged by a d.c. source of emf ε' , using a resistor R.(i) Draw the circuit arrangemnet. (ii) Deduce the expression for (a) the charging current and (b) the potential difference across the combination of the cells
(i) The circuit arrangment for charging the cells is shows in figure
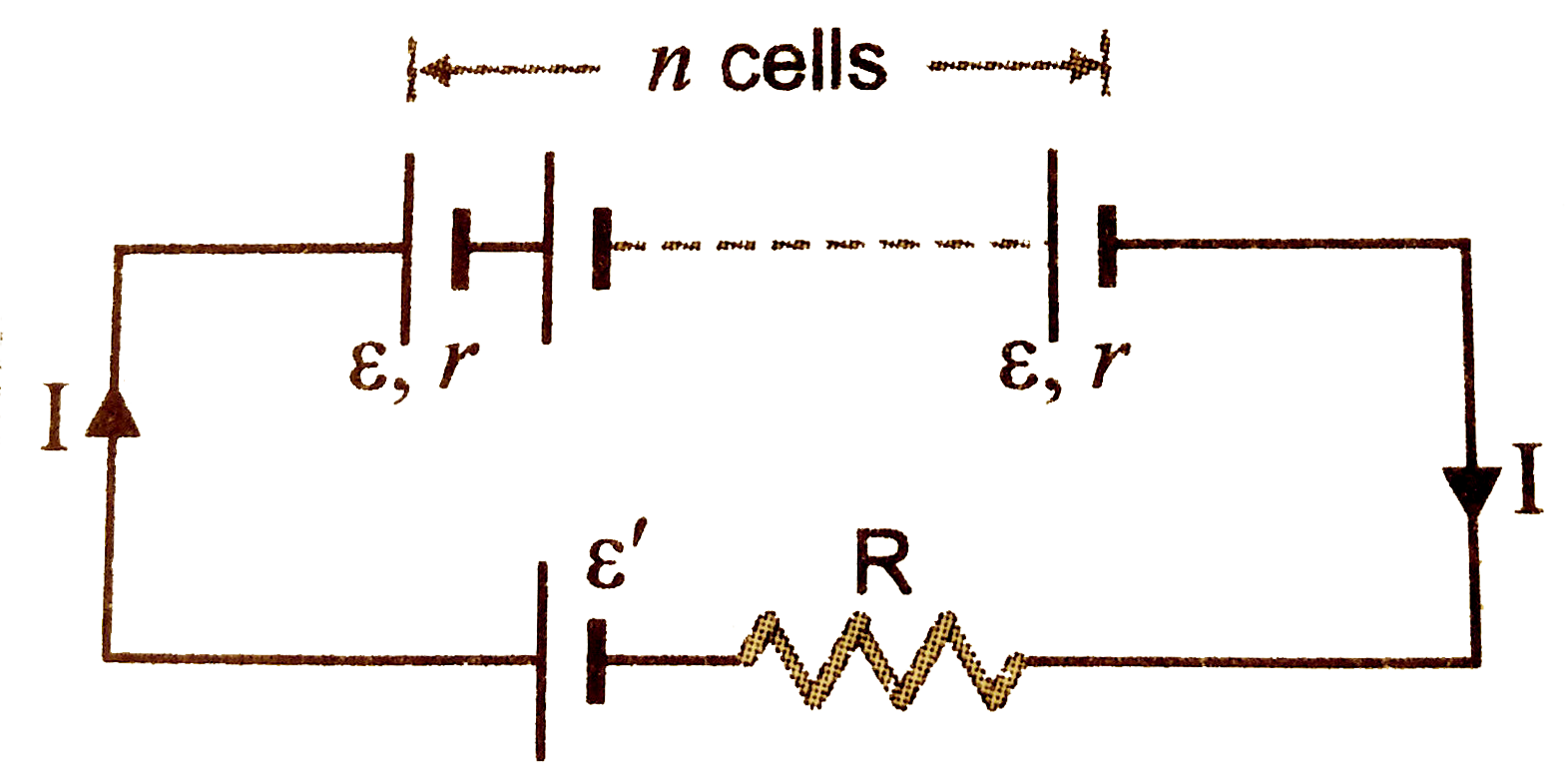
(ii) Effective emf of the circuit = ε'−nε
Total resistance =R+nr
Charging current I=effective emftotal resistnance=ε'−nεR+nr
(iii) Pot. diff. across each charging cell =ε+Ir
ε+(ε'−nεR+nr)r=εR+ε'rR+nr
Pot. diff. across the series combination of n cells
V=n(ε+Ir)=n(εR+ε'rR+nr)
Topper's Solved these Questions
- CURRENT ELECTRICITYBOOK - PRADEEPCHAPTER - CURRENT ELECTRICITYEXERCISE - Conceptual Problems
3 Videos
- CURRENT ELECTRICITYBOOK - PRADEEPCHAPTER - CURRENT ELECTRICITYEXERCISE - Very short Q/A
7 Videos
- COMMUNICATION SYSTEMSBOOK - PRADEEPCHAPTER - COMMUNICATION SYSTEMSEXERCISE - MODEL TEST PAPER-2
9 Videos
- DUAL NATURE OF RADIATION AND MATTERBOOK - PRADEEPCHAPTER - DUAL NATURE OF RADIATION AND MATTEREXERCISE - Exercise
191 Videos
Similar Questions
A number of identical cells, n, each of emf ε , internal resistance r connected in series are charged by a d.c. source of emf ε , using a resistor R.
(i) Draw the circuit arrangement.
(ii) Deduce the expressions for (a) the charging current and (b) the potential difference across the combination of the cells.
View Solutionn-identical cells each of emf E and internal resistance r are connected in series. Find the expression for current in the circuit given below

View Solution
Knowledge Check
A cell of emf ε and internal resistance r is charged by a current I, then
Athe cell stores chemical energy at the rate of εiBthe cell stores chemical energy at the rate of i2rCthe cell stores chemical energy at the rate of εI−i2rDthe storage of chemical energy rate cannot be calculatedn identical cells each of emfe and internal resistance r are joined in series so as to form a closed circuit. The P.D. across any one cell is
AzeroBεCε/nDn−1nεn identical cells, each of emf E and internal resistance r, are joined in series to form a closed circuit. Find the potential difference across any one cell.
AE/nBE/nrCnE/rD0
n identical cells, each of emf ε and internal resistance r, are joined in series to form a closed circuit. One cell A is joined with reversed polarity. The potential difference across any one cell is
View Solutionn identical cells each of e.m.f. E and internal resistance r are connected in series. An external resistance R is connected in series to this combination. The current through R is
View SolutionIn a arrangement, 3n cells of emf ε and internal resistance r are connected in series. Out of 3n cells polarity of n cells is reversed. Current in the circuit is
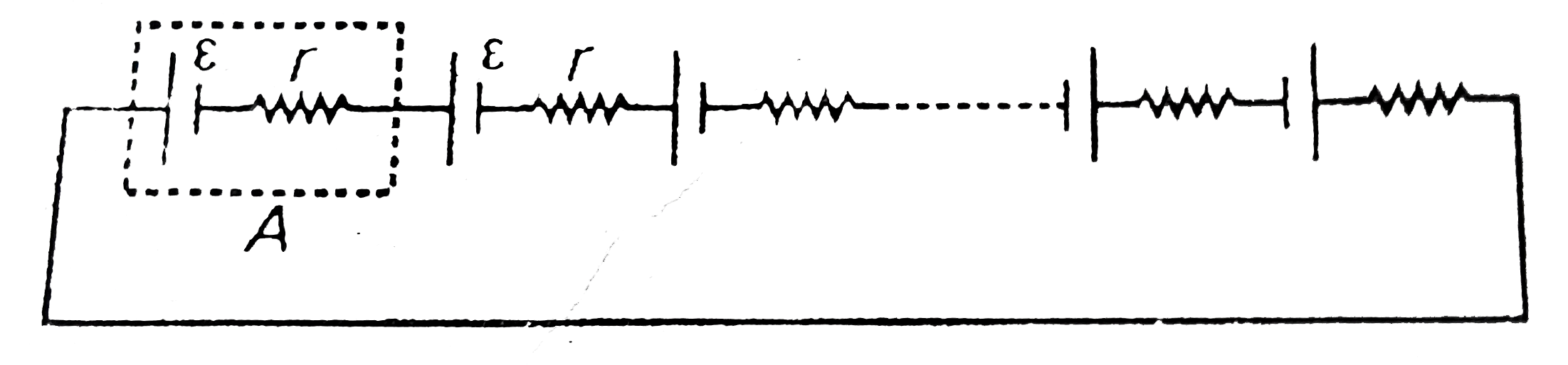
View SolutionFour cells, each of emf E and internal resistance r, are connected in series across an external resistance reverse. Then, the current in the external circuit is
View SolutionTwo cells , each of emfE and internal resistance r, are connected in parallel across a resistor R. The power delivered to the resistor is maximum if R is equal to
View Solution
