A cell of emf ε and internal resistance r is charged by a current I, then
the cell stores chemical energy at the rate of εi
the cell stores chemical energy at the rate of i2r
the cell stores chemical energy at the rate of εI−i2r
the storage of chemical energy rate cannot be calculated
The correct Answer is:C
c. From work-energy theorem, we get Δ=εi−i2r.
Similar Questions
A cell of emf ε and internal resistance r gives a current of 0.5 A with an external resistance of 12Ω and a current of 0.25 A with an external resistance of 25Ω. Calculate (a) internal resistance of the cell and (b) emf of the cell.
View SolutionA cell of emf ε and internal resistance (r) is connected in series with an external resistance (nr) then the ratio of the terminal potential difference to emf is:
View Solution
Knowledge Check
A cell of emf ε and internal resistance r drives a current i through an extermal resistance R
(i) The cell suppllied εi power
(ii) Heat is produced in R at the rate εi
(iii) Heat is produced in R at the rate εI(RR+r)
(iv) Heat is produced in the cell at the rate εi((r?R+r)A(i) , (ii)B(ii), (iii)C(i),(iii),(iv)DallA student measures the terminal potential difference (V) of a cell (of emf ε and internal resistance r) as a function of the current (I) flowing through it. The slope and intercept of the graph between V and I, then respectively, equal
Aε and −rB−r and εCr and −εD−ε and rAn accumulator battery (storage cell) B of emf ε and internal resistance r is being charged from DC supply whose terminals are T1 and T2
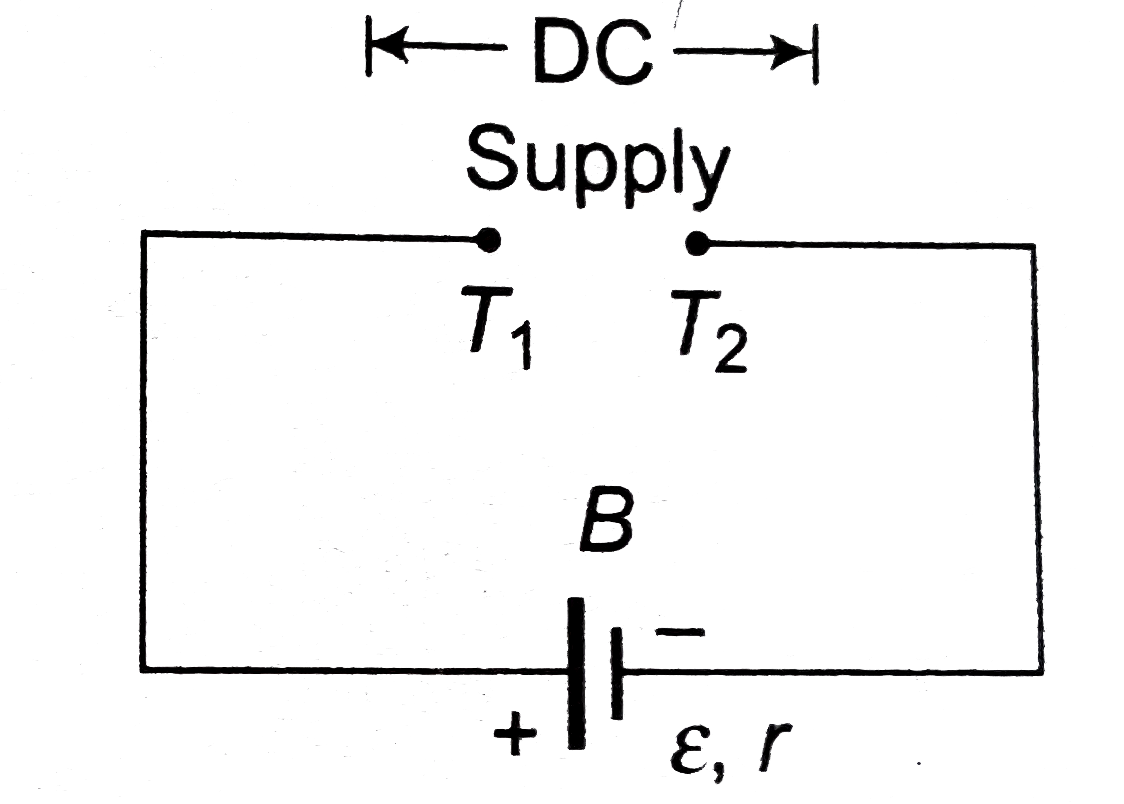 .APotential difference between T1 and T2 must be >εBT1 must be positive with respect to T2CIn the battery, current flows from the positive to the negative terminalDAll the above options are correct.
.APotential difference between T1 and T2 must be >εBT1 must be positive with respect to T2CIn the battery, current flows from the positive to the negative terminalDAll the above options are correct.
A battery of emf ε and internal resistance r sends currents I1 and I2, when connected to external resistance R1 and R2 respectively. Find the emf and internal resistance of the battery.
View SolutionA cell of emf ε and internal resistance r is connected across a variable load resistance R. It is found that when R=4Ω the current is 1A and when R is increased to 9Ω, the current reduces to 0.5A. Find the value of the emf ε and internal resistancer
View SolutionA cell of emf ε and internal resistance r is connected across a variable resistor R. Plot a graph showing variation of terminal voltage V of the cell versus the current I. Using the plot, show how the emf of the cell and its internal resistance can be determined.
View SolutionA cell of emf ε and internal resistance r is connected across a load resistance R
(i) Find the maximum power delivered at the load ?
(ii) Draw the power (P ) vs load resistance ( R ) graph.
View SolutionN cells, each of emf ε and internal resistance r, are arranged in a ring in series. Two points including n cells on one side and N-n cells on the other side are connected to a resistor R. Calcualte the current through R (figure)
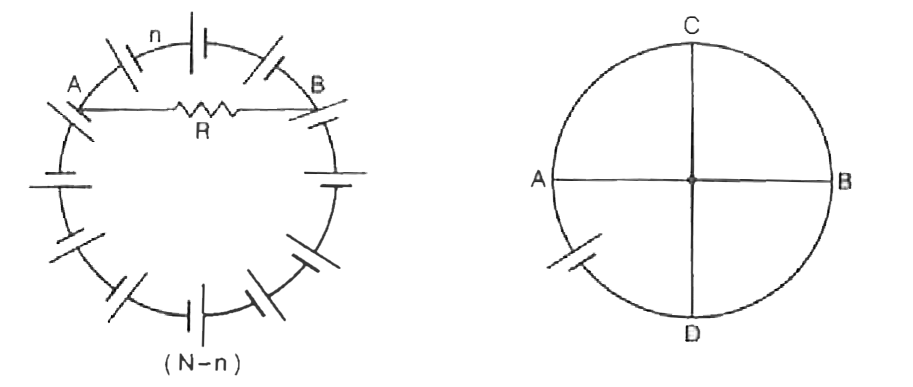
View Solution
