The space between the plates of a parallel plate capacitor is filled conseutively with two dielectric layers fo thickness d1andd2 having relative permittvities ∈1and∈2 respectively. If A is area of each plate, what is the capacity of the capacitor ?
As effective thickness of dielectric os 1/∈ times the actual thickness, therefore, we may write the capacity of condenser as C=∈0Ad1∈1+d2∈2
Similar Questions
The space between the plates of a parallel-plate capacitor is filled consecutively with two dielectric layers 1 and 2 having the thickness of d1 and d2 and the permittivities esπlon1 and esπlon2 respectively. The area of each plate is equal to S. Find :
View SolutionThe distance between the plates fo a parallel plate capacitor is d. A metal plate of thickness d/2 is placed between the plates, what will be the new capacity ?
View Solution
Knowledge Check
A parallel plate capacitor is filled with dielectrics. What is capacitance?
 A2K1K2K1+K2BK1K2K1+K2C2ε0Ad(K1K2K1+K2)D2ε0Ad(2K1+K22K1K2)
A2K1K2K1+K2BK1K2K1+K2C2ε0Ad(K1K2K1+K2)D2ε0Ad(2K1+K22K1K2)In figure half of the space between the plates of a parallel plate capacitor is filled with dielectric material of constant K. Then which of the plots are possible?
 A
A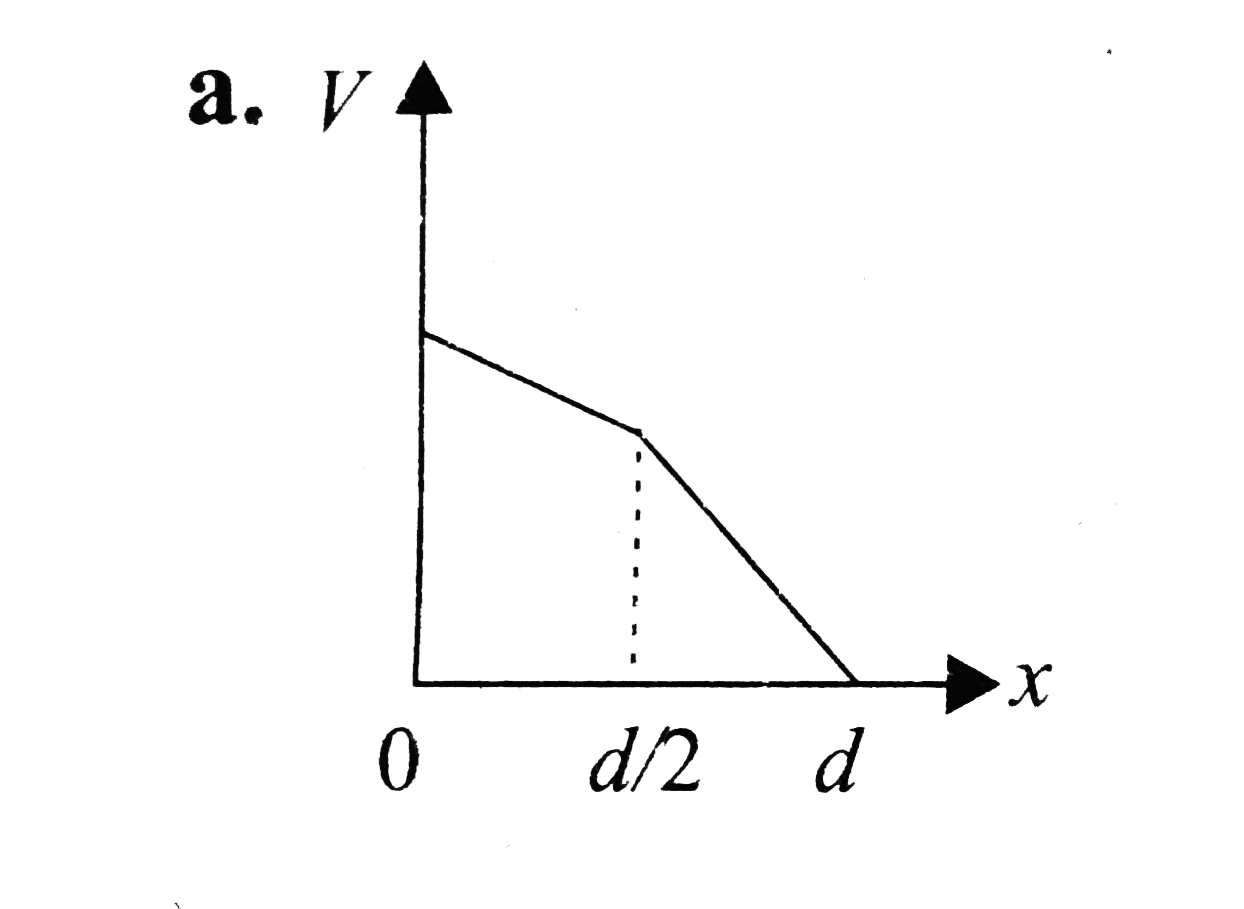 B
B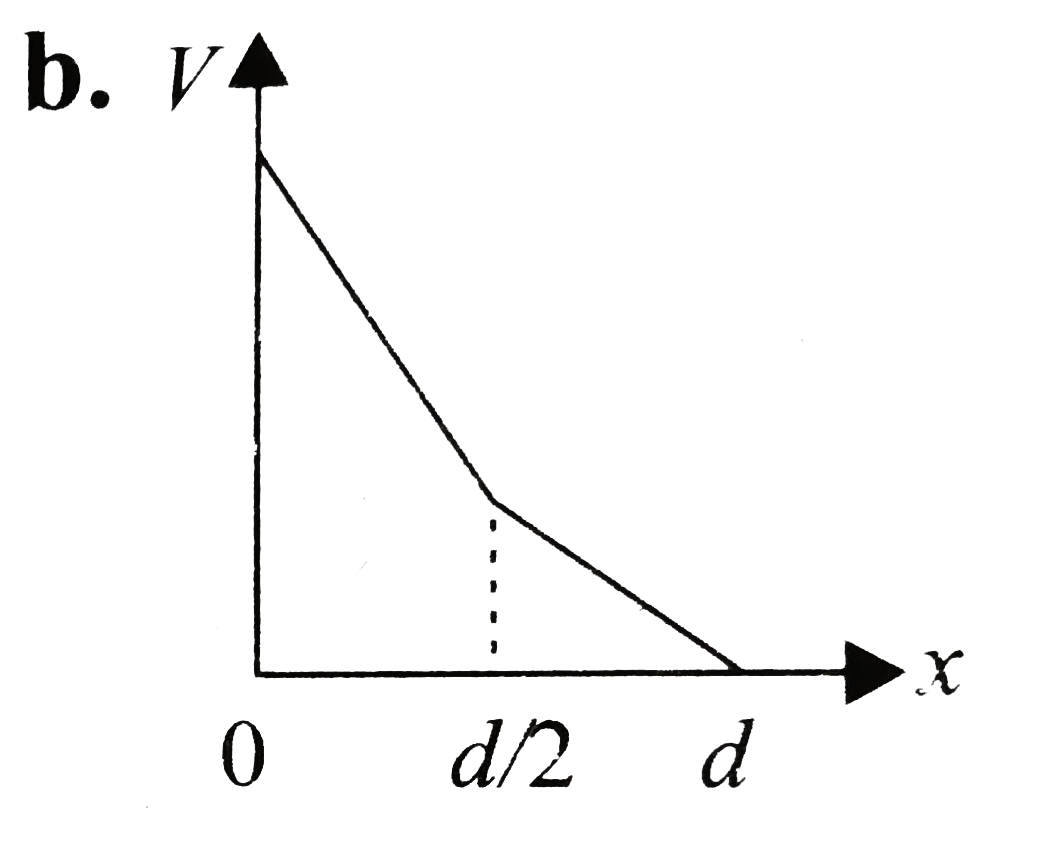 C
C D
D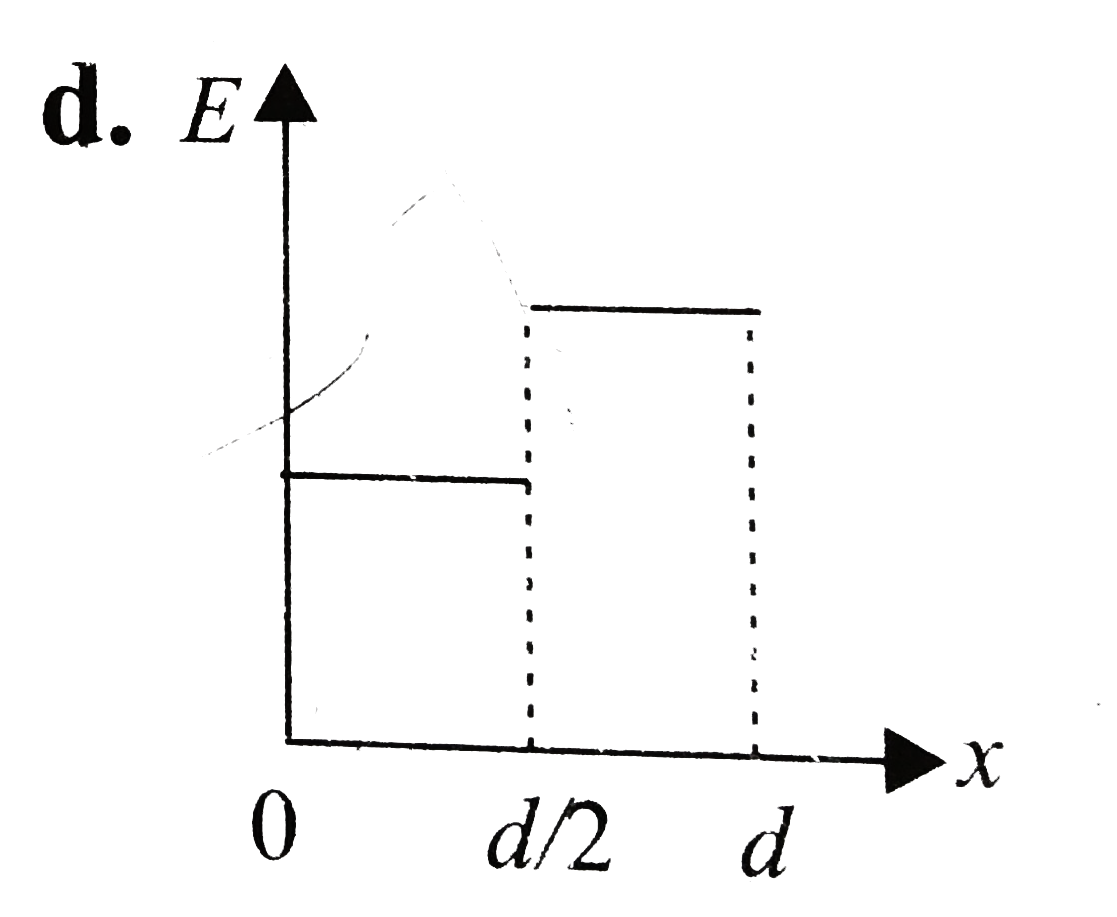
Space between the plates of a parallel plate capacitor is filled with a dielectric slab. The capacitor is charged and then the supply is disconnected to it. If the slab is now taken out then
Awork is not done to take out the slabBenergy stored in the capacitor reducesCpotential difference across the capacitor is decreasedDpotential difference across the capacitor is increased
The distance between the plates of a parallel plate capacitor is 'd'. The capacitance of the capacitor gets doubled when a metal plate of thicknessd2 is placed between the plates.
View SolutionThe' distance between the plates of a parallel plate capacitor is d. A metal plate of thickness d/2 is placed between the plates. What will e its effect on the capacitance.
View SolutionThe space between parallel plate capacitors is filled with four dielectrics of equal dimensions but of dielectric constants K1,K2,K3andK4 respectively. If K is the dielectric constant of a single dielectric that must be filled between capacitor plates to have the same capacitance between A and B. Then we must have
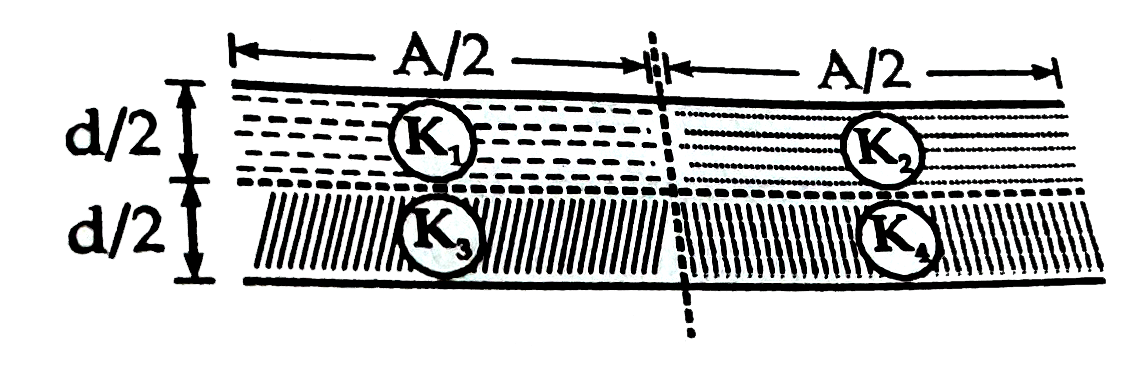
View SolutionA parallel plate capacitor of plate area A and separation d is filled with two materials each of thickness d2 and dielectric constants ∈1 and ∈2 respectively. The equivalent capacitance will be

View SolutionThe separation between the plates of a parallel plate capacitor is d and the area of each plate is A. iff a dielectric slab of thickness x and dielectric constant K is introduced between the plates, then the capacitance will be
View Solution