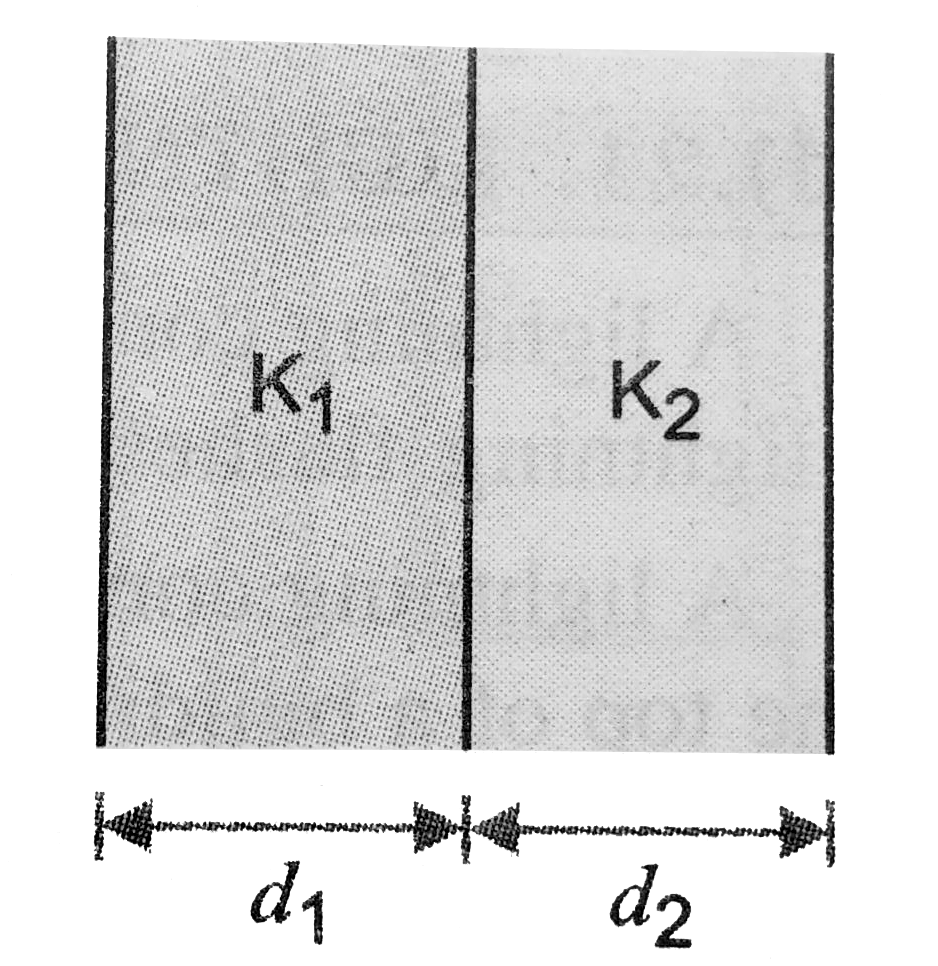
Find the capacitance of a system of three parallel plates, each of area A metre2 separated by distances d1andd2 metre respectively. The space between them is filled with dielectrics fo relatives dielectric constants K1andK2. The dielectric constant of free space is ∈0.
A system of three parallel plates is forming two capacitors of capacities C1andC2 connected in series as shown in Fig.
C1=K1∈0Ad1andC2=K2∈0Ad2
The equivalent capacity of the system is
1C=1C1+1C2orC=C1C2C1+C2
C=(K1∈0Ad1)(K2∈0Ad2)∈0A(K1d1+K2d2)=K1K2A(∈0d1)(∈0d2)d1d2∈0A(K1d2+K2d1)=K1K2∈0k1d2+K2d1
Similar Questions
Two parallel plate capacitors, each of capacitance 40 muF, are connected is series. The space between the plates of one capacitor is filled with a dielectric material of dielectric constant K =4. Find the equivalent capacitacne of the system.
View SolutionThe capacitance of a parallel plate capacitor with plate area A and separation d is C. The space between the plates in filled with two wedges of dielectric constants K1 and K2 respectively. Find the capacitance of resulting capacitor.

View Solution
Knowledge Check
A system of two parallel plates, each of area A, are separated by distance d1 and d2. The space between them is filled with dielectrices of permitivities ε1 and ε)2. The permittivity of free space is ε0. The equivalent capacitance of the system is
Aε1ε2Aε2d1+ε1d2Bε1ε2ε0Aε1d1+ε2d2Cε0Aε1d1+ε2d2Dε0Aε1d2+ε2d1A capacitor of plate area A and separation d is filled with two dielectrics of dielectric constant K1=6andK2=4. New capacitance will be
 A4Aε0dB4.8Aε0dC5Aε0dD2.4Aε0d
A4Aε0dB4.8Aε0dC5Aε0dD2.4Aε0dThe capacitance of a capacitor, filled with two dielectrics of same dimensions but of dielectric constants K1andK2 respectively as shown will be -
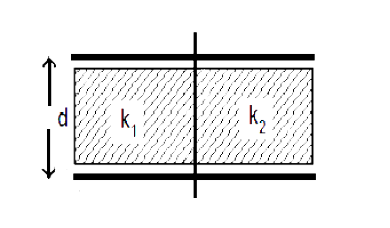 A∈0A2d(K1+K2)B∈0Ad(K1+K2)C∈0A2d(K1+K2K1+K2)D∈0Ad(K1K2K1+K2)
A∈0A2d(K1+K2)B∈0Ad(K1+K2)C∈0A2d(K1+K2K1+K2)D∈0Ad(K1K2K1+K2)
The capacitance of a parallel plate capacitor with plate area A and separation d is C. The space between the plates in filled with two wedges of dielectric constants K1 and K2 respectively. Find the capacitance of resulting capacitor.

View SolutionA capacitor is composed of three parallel conducting plates. All three plates are of the same area A. The first pair of plates are kept a distance d1 apart, and the space between them is filled with a medium of a dielectric K1 . The corresponding data of the second pair are d2 and K2, respectively. The middle plate is connected to the positive terminal of a constant voltage source V and the external plates are connected the other terminal of V.
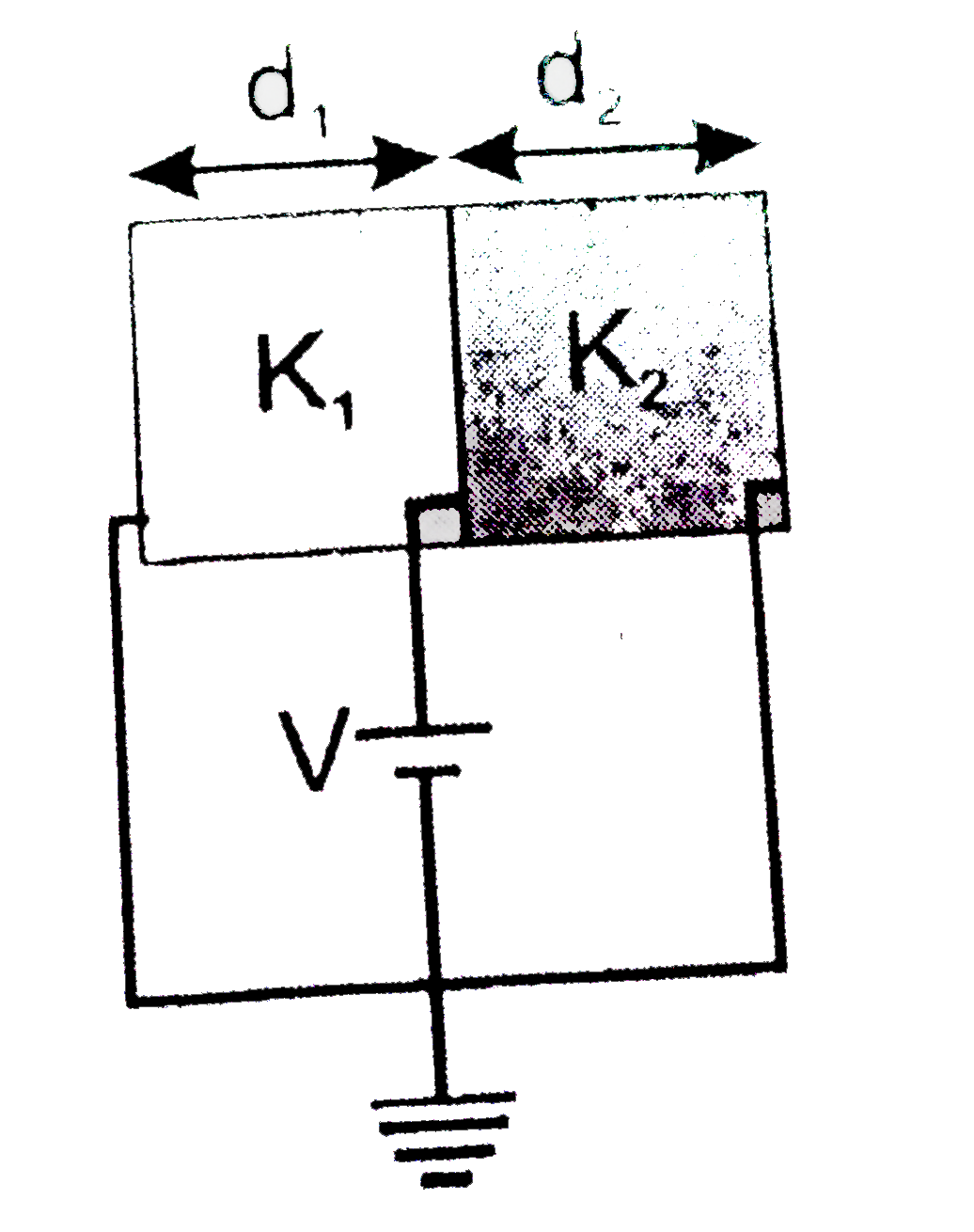
(a) Find the capacitance of the system .
(b) What is the surface charge density on the middle plate ?
(c) Compute the energy density in the medium K1
View SolutionA parallel plate capacitor with air between the plates has a capacitance of 9 pF. The separation between its plates is ‘d'. The space between the plates is now filled with two dielectrics. One of the dielectrics has dielectric constant K1=3 and thickness d3 while the other one has dielectric constant K2=6 and thickness 23d. Capacitance of the capacitor is now
View SolutionA parallel plate capacitor with air between the plates has a capacitance C. If the distance between the plates is doubled and the space between the plates is filled with a dielectric of dielectric constant 6, then the capacitance will become.
View SolutionA parallel plate capacitor with air between the plates has capacitance of 9pF. The separation between its plates is 'd'. The space between the plates is now filled with two dielectrics. One of the dielectrics has dielectric constant k1=3 and thickness d3 while the other one has dielectric constant k2=6 and thickness 2d3. Capacitance of the capacitor is now
View Solution
