A system of two parallel plates, each of area A, are separated by distance d1 and d2. The space between them is filled with dielectrices of permitivities ε1 and ε)2. The permittivity of free space is ε0. The equivalent capacitance of the system is
ε1ε2Aε2d1+ε1d2
ε1ε2ε0Aε1d1+ε2d2
ε0Aε1d1+ε2d2
ε0Aε1d2+ε2d1
The correct Answer is:A
To find the equivalent capacitance of a system of two parallel plates filled with dielectrics, we can follow these steps:
Step 1: Understand the Configuration
We have two parallel plates, each with an area A, separated by two different distances d1 and d2. The space between the plates is filled with two different dielectrics with permittivities ϵ1 and ϵ2.
Step 2: Identify the Capacitance of Each Section
The capacitance of a parallel plate capacitor filled with a dielectric is given by the formula:
C=ϵAd
For the first section with dielectric ϵ1 and distance d1:
C1=ϵ1Ad1
For the second section with dielectric ϵ2 and distance d2:
C2=ϵ2Ad2
Step 3: Determine the Equivalent Capacitance
Since the two capacitors C1 and C2 are in series, the equivalent capacitance C can be calculated using the formula for capacitors in series:
1C=1C1+1C2
Substituting the expressions for C1 and C2:
1C=d1ϵ1A+d2ϵ2A
Step 4: Combine the Terms
To combine the terms, we can find a common denominator:
1C=d1ϵ2+d2ϵ1ϵ1ϵ2A
Step 5: Invert to Find C
Now, we can invert both sides to find C:
C=ϵ1ϵ2Ad1ϵ2+d2ϵ1
Final Answer
Thus, the equivalent capacitance of the system is:
C=ϵ1ϵ2Ad1ϵ2+d2ϵ1
---
We have two parallel plates, each with an area A, separated by two different distances d1 and d2. The space between the plates is filled with two different dielectrics with permittivities ϵ1 and ϵ2.
The capacitance of a parallel plate capacitor filled with a dielectric is given by the formula:
C=ϵAd
For the first section with dielectric ϵ1 and distance d1:
C1=ϵ1Ad1
For the second section with dielectric ϵ2 and distance d2:
C2=ϵ2Ad2
Since the two capacitors C1 and C2 are in series, the equivalent capacitance C can be calculated using the formula for capacitors in series:
1C=1C1+1C2
Substituting the expressions for C1 and C2:
1C=d1ϵ1A+d2ϵ2A
To combine the terms, we can find a common denominator:
1C=d1ϵ2+d2ϵ1ϵ1ϵ2A
Now, we can invert both sides to find C:
C=ϵ1ϵ2Ad1ϵ2+d2ϵ1
Thus, the equivalent capacitance of the system is:
C=ϵ1ϵ2Ad1ϵ2+d2ϵ1
Topper's Solved these Questions
- ELECTROSTATIC POTENTIAL AND CAPACITANCEBOOK - DISHA PUBLICATIONCHAPTER - ELECTROSTATIC POTENTIAL AND CAPACITANCEEXERCISE - EXERCISE -1: CONCEPT BUILDER
58 Videos
- ELECTROMAGNETIC WAVESBOOK - DISHA PUBLICATIONCHAPTER - ELECTROMAGNETIC WAVESEXERCISE - Exercise - 2 : Concept Applicator
30 Videos
- GRAVITATIONBOOK - DISHA PUBLICATIONCHAPTER - GRAVITATIONEXERCISE - EXERCISE-2
30 Videos
Similar Questions
Find the capacitance of a system of three parallel plates, each of area A metre2 separated by distances d1andd2 metre respectively. The space between them is filled with dielectrics fo relatives dielectric constants K1andK2. The dielectric constant of free space is ∈0.
View SolutionThe plates of a parallel plate capacitance 1.0 F are separated by a distance d =1 cm. Find the plate area .
View Solution
Knowledge Check
The dimensions of 12ε0E2(ε0= permittivity of free space, E= electric field) is
A[MLT−1]B[ML−1T−2]C[MLT−2]D[ML2T−1]The dimension of (12)ε0E2 (ε0 : permittivity of free space, E electric field
A(a) MLT−1B(b) ML2T2C( c ) ML−1T−2D( d ) ML2T−1The dimensions of 12∈0E2, where ∈0 is permittivity of free space and E is electric field, is :-
A[M1L1T−2A1]B[M1L0T−1]C[M1L−1T−2A1]D[M1L−1T−2]
The capacitance of a parallel plate capacitor with plate area A and separation d is C. The space between the plates in filled with two wedges of dielectric constants K1 and K2 respectively. Find the capacitance of resulting capacitor.

View SolutionA parallel plate capacitor has square plates of area A separated by distance ‘d’ between them. It is filled with a dielectric which has a dielectric constant that varies as k(y)=K0(1+βy) where 'y' is the distance measured from bottom of capacitor. The total capacitance of the system is given by the expression:
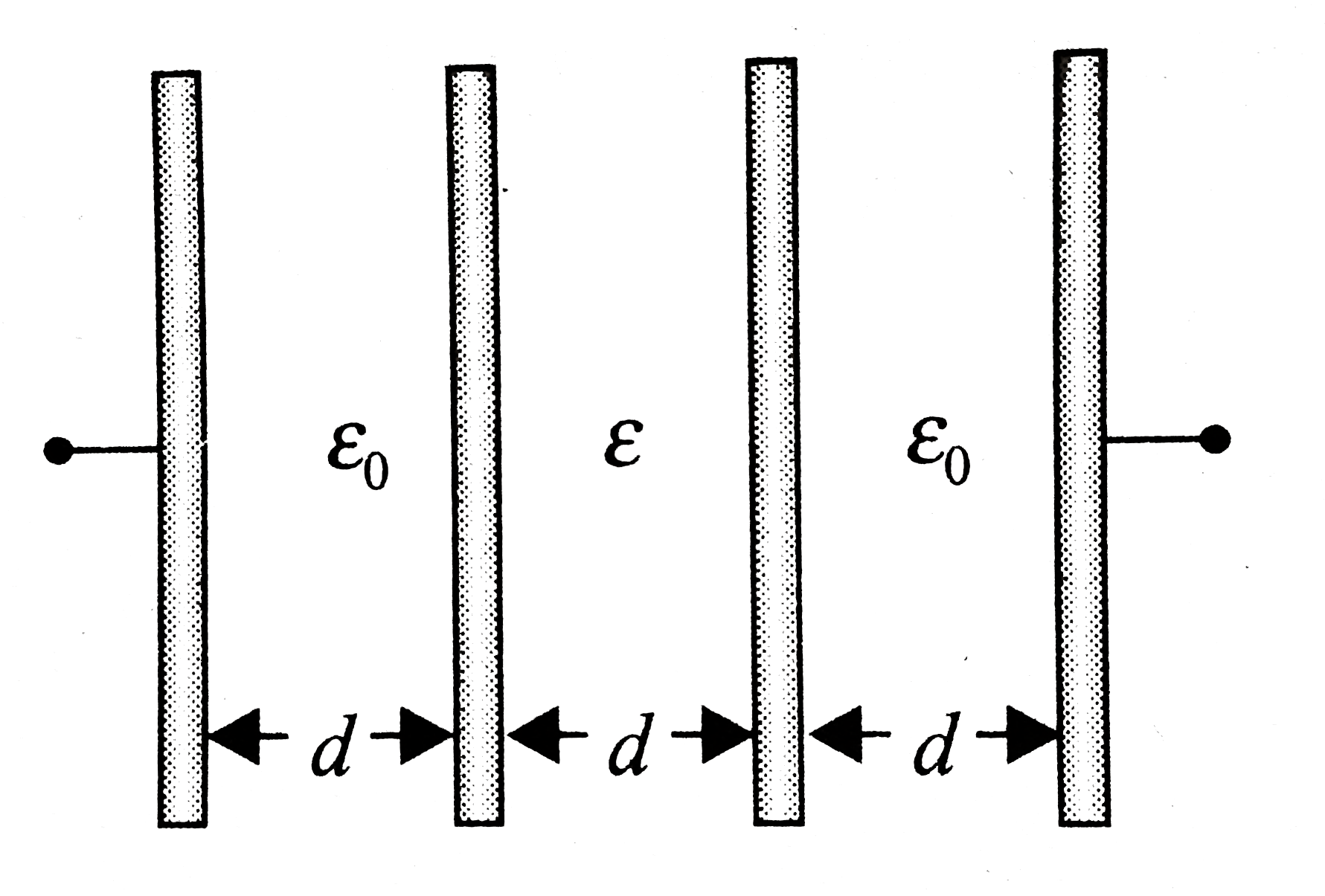
View SolutionFor the congiuration of mede of permittiveties epslon0,ε, and ε0 between parallel plates each of area A, as shown in the equivalent capacitance is .
 .
.
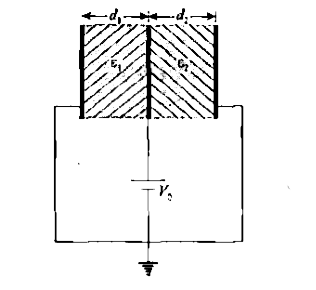
View SolutionA capacitor is composed of three parallel conducting plates. All three plates are of same area A. The first pair of plates are kept a distance d1 apart and the space between them is filled with a medium of a dielectric ε1. The corresponding values for the second pair of plates are d2. and ε2 respectively. What is the surface charge density on the middle plate?
View SolutionIf C is capacitance, Vis potential, ρ is specific resistance and ε0 is permittivity of free space, then the dimensions of CVρε0 are same as that of
View Solution
