A particle starts from a point P at a distance of A/2 from the mean position O& travels towards left as shown in the figure. If the time period of SHM, executed about O is T and amplitude A then the equation of motion of particle is :
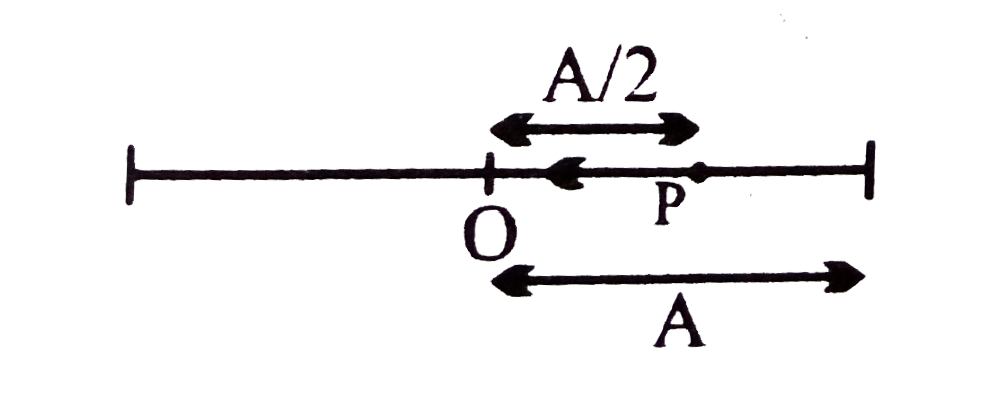
X=Asin(2πTt+π6)
X=Asin(2πTt+5π6)
X=Acos(2πTt+π6)
X=Acos(2πTt+π3)
The correct Answer is:B, D
Figure
Similar Questions
A particle start form mean position and moves towards positive extreme as shown. Find the equation of the SHM. Amplitude of SHM is A.
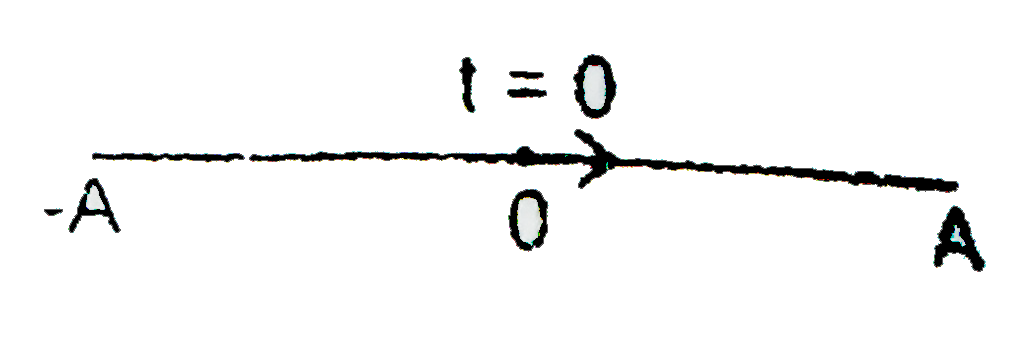
View SolutionA particle starts from mean position and moves towards positive extreme as show below. Find the equation of the SHM, Amplitude of SHM is A.
_S01_003_Q01.png)
View Solution
Knowledge Check
A particle starts from a point P at a distance of A/2 from the mean position O and travels towards left as shown in the figure. If the time period of SHM, executed about O is T and amplitude A then the equation of the motion of particle is
 Ax=Asin(2πTt+π6)Bx=Asin(2πTt+5π6)Cx=Acos(2πTt+π6)Dx=Acos(2πTt+π3)
Ax=Asin(2πTt+π6)Bx=Asin(2πTt+5π6)Cx=Acos(2πTt+π6)Dx=Acos(2πTt+π3)A particle executes SHM of amplitude A. At what distance from the mean position is its KE equal to its PE ?
A0.71 AB0.61 AC0.65 AD0.8 AThe displacement from mean position of a particle in SHM at 3 seconds is √3 /2 of the amplitude. Its time period will be :
A18 sec.B6√3sec.C9sec.D3√3sec.
The figure shows the displacement-time graph of a particle executing SHM. If the time period of oscillation is 2s, then the equation of motion is given by
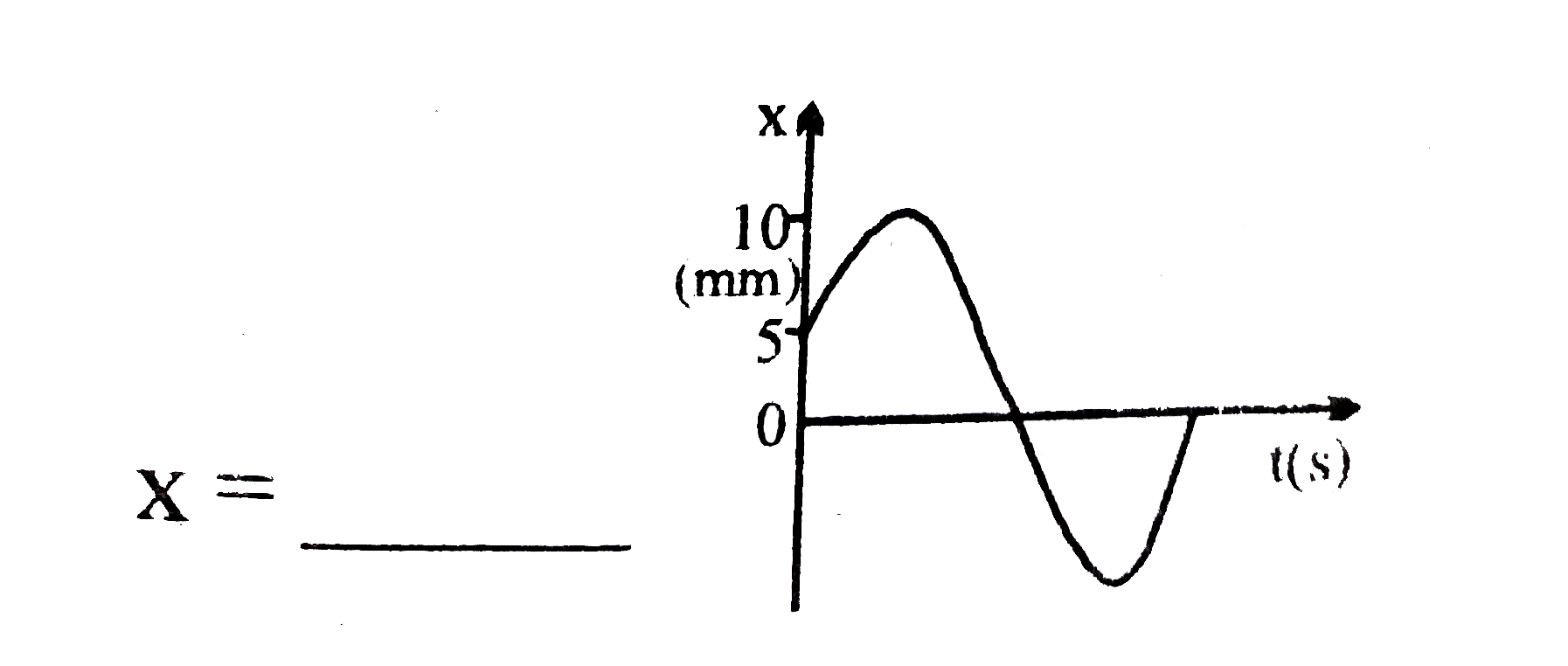
View SolutionThe period of a particle executing SHM is T. There is a point P at a distance 'x' from the mean position 'O'. When the particle passes P towards OP, it has speed v. Find the time in which it returns to P again.
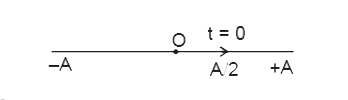
View SolutionA particle starts from A/2 and moves towards positive extreme as shown below. Find the equation of the SHM. Given amplitude of SHM is A.
View SolutionThe displacement (from intial position) of a particle executing SHM with amplitude A in half the time period is always
View SolutionA particle executing S.H.M. starts from the mean position. Its phase, when it reaches the extreme position, is
View Solution