A liquid flows through a horizontal tube as shown in figure. The velocities of the liquid in the two sections, which have areas of cross-section and and and respectively. The difference in the levels of the liquid in the two vertical tubes is , then

The correct Answer is:A
Let be density of liquid flowing in the tube.
According to Bernoulli's equation for horizontal of liquid,
)
.
Topper's Solved these Questions
- FLUID MECHANICSBOOK - A2ZCHAPTER - FLUID MECHANICSEXERCISE - Application Of Bernoulli'S Theorem And Velocity Of Efflux
19 Videos
- FLUID MECHANICSBOOK - A2ZCHAPTER - FLUID MECHANICSEXERCISE - Problems Based On Mixed Concepts
29 Videos
- FLUID MECHANICSBOOK - A2ZCHAPTER - FLUID MECHANICSEXERCISE - Buoyancy And Floatation
40 Videos
- GENERAL KINEMATICS AND MOTION IN ONE DIMENSIONBOOK - A2ZCHAPTER - GENERAL KINEMATICS AND MOTION IN ONE DIMENSIONEXERCISE - Chapter Test
30 Videos
Similar Questions
Water flows through a horizontal tube as shown in figure. If the difference of height of water column in the vertical tubes in 2cm and the areas of corss-section at A and B are 4cm2 respectively. Find the rate of flow of water across any section.
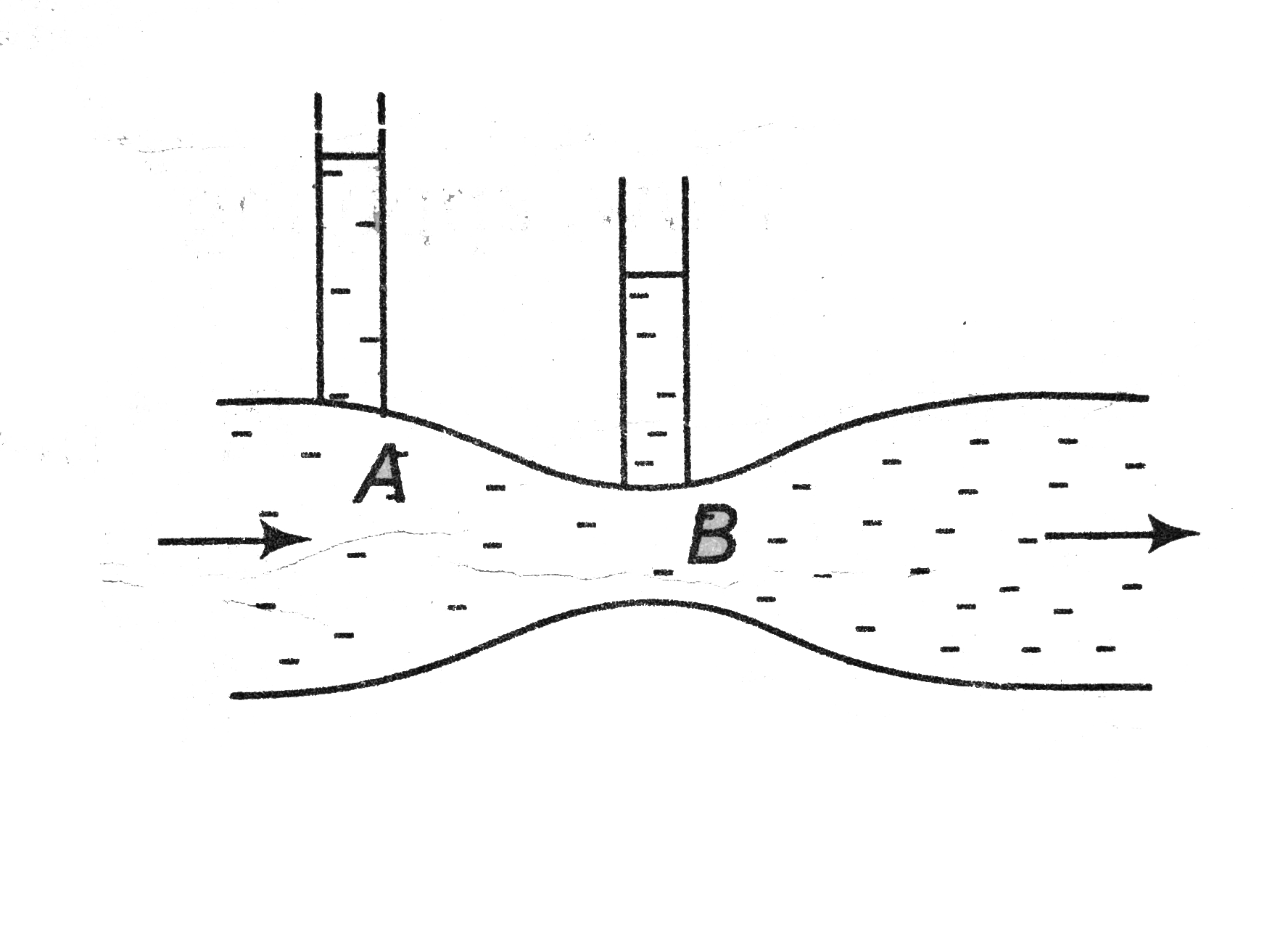 .
.
View SolutionWater flows through a horizontal tube as shown in figure. If the difference of heights of water colun in the vertical tubes is 2 cm and the area of cross section at A and B are 4cm2and2cm2 respectively, find the rate of flow of water across any section.

View Solution
Knowledge Check
A liquid flows through a horizontal tube. The velocities of the liquid in the two sections, which have areas of cross section A1 and A2 are v1 and v2 respectively. The difference in the levels of the liquid in the two vertical tubes is h. Then
 Athe volume of the liquid flowing through the tube in unit time is A1v1Bv2−v1=√2ghCv22−v21=2ghDthe energy per unit mass of the liquid is the same in both sections of the tube
Athe volume of the liquid flowing through the tube in unit time is A1v1Bv2−v1=√2ghCv22−v21=2ghDthe energy per unit mass of the liquid is the same in both sections of the tubeAn incompressible liquid flows through a horizontal tube as shown in the figure. Then the velocity 'v' of the fluid is:
 A3.0 m/sB1.5 m/sC1.0 m/sD2.25 m/s
A3.0 m/sB1.5 m/sC1.0 m/sD2.25 m/s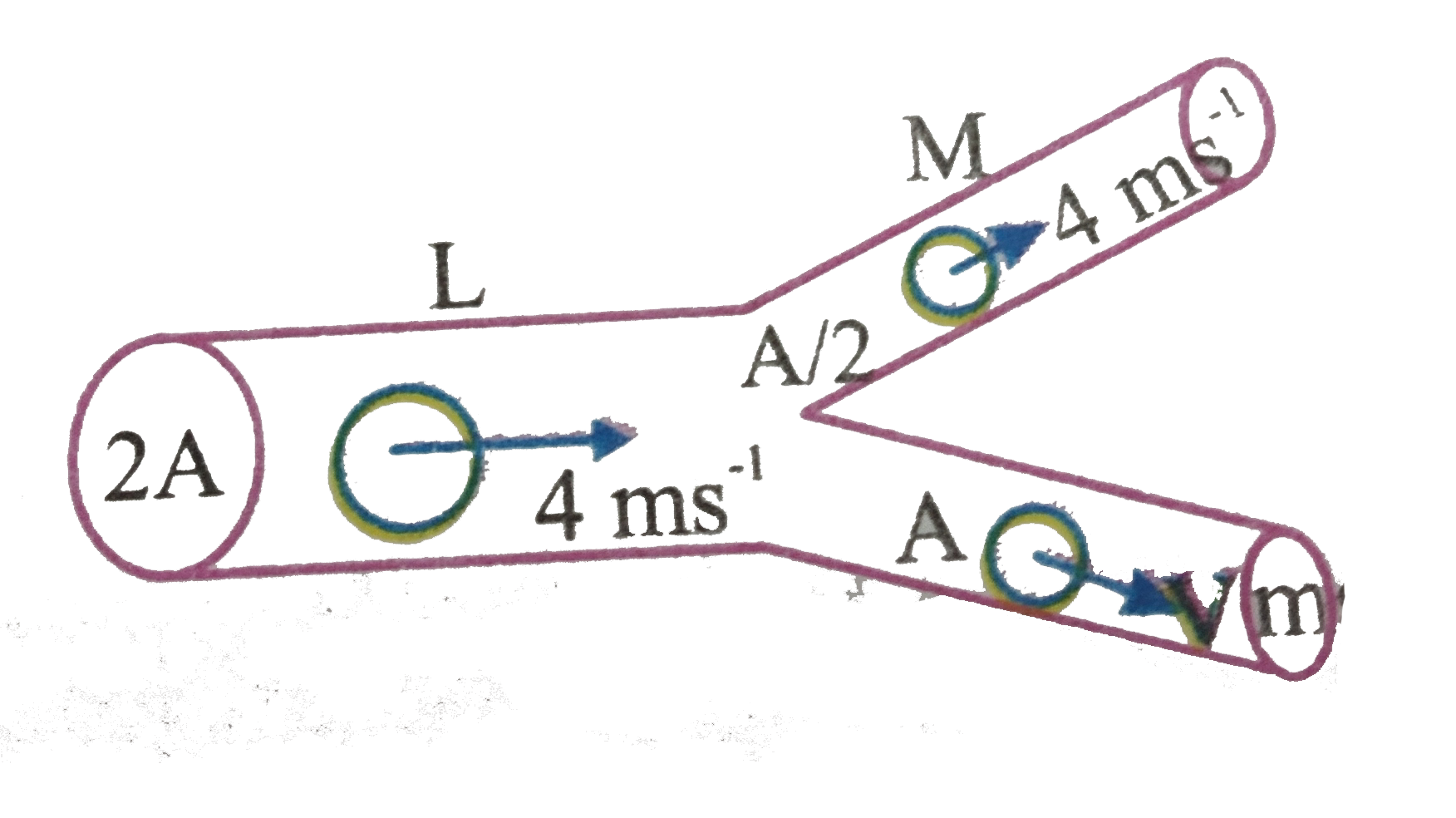
An incompressible liquid flows through a horizontal tube LMN as shown in the figure. Then the velocity V of the liquid throught he tube N is:A1ms−1B2ms−1C4.5ms−1D6ms−1
An incompressible, non-viscous liquis of density r flows through a horizontal tube as shown below. The flow condition is steady. Area of cross section at A and B are a1anda2 respectively. The height difference of liquid in two tubes inserted at A and B is h.
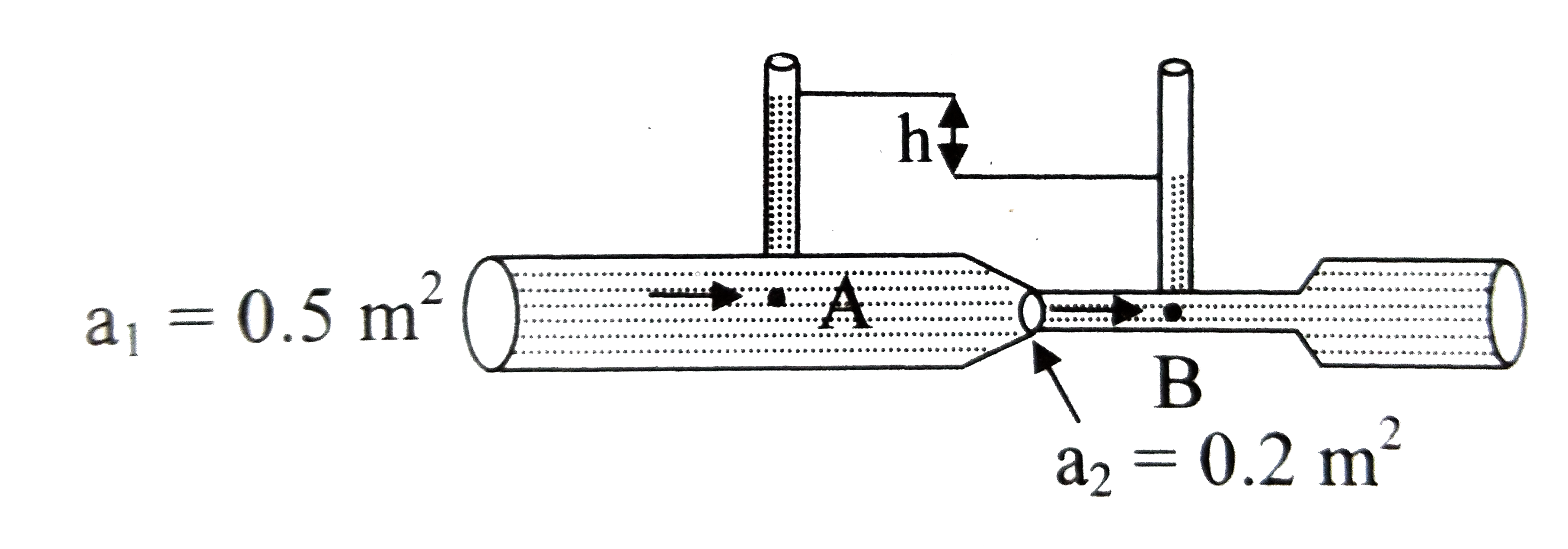
View SolutionFrom a horizontal tube with area of cross-section A1 and A2 as shosn in fiugre liquid is flowing in the level of the liauid in the two veritcal tunes is h.

View SolutionFig, shows a U-tube of uniform cross-sectional area A accelerated with acceleration a as shown. If d is the seperation between the limbs. Then the difference in the levels of the liquid in the U−tube is

View SolutionThe mass of the liquid flowing per second per unit area of cross section of the tube is proportional to Pxandvy , where P is the pressure difference and v is the velocity , then the relation between x and Y is
View SolutionA liquid flows through a pipe of non-uniform cross-section. If A1andA2 are the cross-sectional areas of the pipe at two points, the ratio of velocities of the liquid at these points will be
View Solution
