A spherical ball of radius r and mass m is rolling without slipping on a horizontal table. Calculate the percentage of KE, which is translational.
Total KE=KE of translation +KE of rotation
E=12mv2+12Iω2
Now, for the ball, I=25mr2
∴E=12mv2+12×25mr2ω2
E=12mv2+12mv2=710mv2
EtranslationalEtotal=12mv2710mv2=1014=57
=57×100%=71.4%
Topper's Solved these Questions
- SYSTEMS OF PARTICLES AND ROTATIONAL MOTIONBOOK - PRADEEPCHAPTER - SYSTEMS OF PARTICLES AND ROTATIONAL MOTIONEXERCISE - SOLVED EXAMPLES TYPE B
2 Videos
- SYSTEMS OF PARTICLES AND ROTATIONAL MOTIONBOOK - PRADEEPCHAPTER - SYSTEMS OF PARTICLES AND ROTATIONAL MOTIONEXERCISE - SOLVED EXAMPLES TYPE C
2 Videos
- SYSTEMS OF PARTICLES AND ROTATIONAL MOTIONBOOK - PRADEEPCHAPTER - SYSTEMS OF PARTICLES AND ROTATIONAL MOTIONEXERCISE - SOLVED EXAMPLES TYPE A
2 Videos
- RAY OPTICSBOOK - PRADEEPCHAPTER - RAY OPTICSEXERCISE - Problem For Practice(a)
25 Videos
- THERMODYNAMICSBOOK - PRADEEPCHAPTER - THERMODYNAMICSEXERCISE - Assertion- Reason Type Questions
19 Videos
Similar Questions
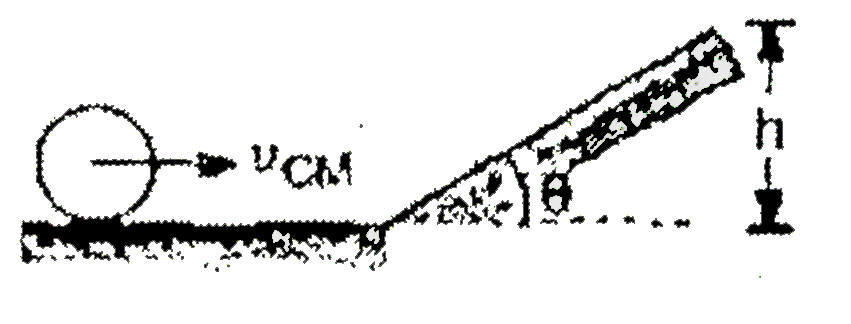
A ball of radius R and mass m is rolling without slipping on a horizontal surface with velocity of its centre of mass vCM. It then rolls wilthout slippig up a hill to a height h before momentarily coming to rest. Find h
View SolutionRolling (with and without slipping) on horizontal and inclined surface
View Solution
Knowledge Check
When a hollow sphere is rolling without slipping on a rough horizontal surface then the percentage of its total K.E. which is translational is
A72%B28%C60%D40%A symmetrical body of mass M and radius R is rolling without slipping on a horizontal surface with linear speed v. Then its angular speed is
Av/RBContinuously increasingCDependent on mass MDIndependent of radius (R)A solid sphere of mass m and radius R rolls without slipping on a horizontal surface such that vc.m.=v0.
AThe kinetic energy of rotation is 15mv20.BThe total kinetic energy is 710mv20.CThe mechanical energy (assume the ground as reference) is mgR+710mv20.DAll options are correct.
A circular disc of radius r is rolling without slipping on a horizontal surface. What is the ratio of the translational KE and rotational KE of disc ?
View SolutionA disc of mass 100 g is rolling without slipping on a horizontal surface with a velocity of 10cms−1. Calculate its total energy.
View SolutionA drum of radius R and mass M rolls down without slipping along an inclined plane of angle θ. The frictional force
View SolutionA drum of radius R and mass M, rolls down without slipping along an inclined plane of angle θ. The frictional force-
View SolutionA drum of radius R and mass M, rolls down without slipping along an inclined plane of angle θ. The frictional force-
View Solution
