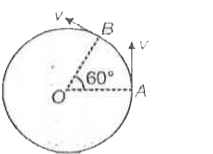
A particle is moving in a circle of radius r centred at O with constant speed v. What is the change in velocity in moving from AtoB(∠AOB=40∘) ?
2vcos40∘
2vsin20∘
2vcosec40∘
2vsec20∘
The correct Answer is:B
Here, ∣∣→A∣∣=|→–––v|=v
Change in velocity <br> Delta vec v = vec _B - vec v_A = vec v_B + (- verc v_A)<br><imga≤ocr_imageload∈g=lazysrc=https://d10lpgp6xz60nq.cloudfront.net/physics_images/PR_XI_V01_C02_E01_420_S01.pngwh=80%mx-autosty≤=background:white;>.<br>Ang≤between vec v _Band (-vec v_A)<br> = 80^@ - 40^@ = 140^@<br>Δv=√v2+v2+2v.vcos140∘
=√v2+v2+2v2cos(180∘)−40∘
= sqrt ( v^2 xx 2 sin ^2 20^@) = 2 v sin 20 .
Topper's Solved these Questions
- KINEMATICSBOOK - PRADEEPCHAPTER - KINEMATICSEXERCISE - 4 NCERT multiple Choice
10 Videos
- KINEMATICSBOOK - PRADEEPCHAPTER - KINEMATICSEXERCISE - 4 NCERT Integer type
4 Videos
- KINEMATICSBOOK - PRADEEPCHAPTER - KINEMATICSEXERCISE - 2 NCERT multiple
15 Videos
- GRAVIATIONBOOK - PRADEEPCHAPTER - GRAVIATIONEXERCISE - Assertion-Reason Type Questions
19 Videos
- LAWS OF MOTIONBOOK - PRADEEPCHAPTER - LAWS OF MOTIONEXERCISE - Assertion- Reason Type Questions
17 Videos
Similar Questions
In Fig. a particle is moving in a circle of radius r centred at O with constant speed v. what is the change in velocity in moving from A to B ? Given ∠AOB=40∘.
View SolutionA particle is moving in a circle of radius having centre at 0. with a constant speed V. The magnitude at change in velocity in moving from A to B is 60°
View Solution
Knowledge Check
A particle is moving in a circle of radius r having centre at O with a constant speed v . The magnitude of change in velocity in moving from A to B is
 A2 vBZeroC√3vDv
A2 vBZeroC√3vDvA particle is moving in a circle of radius r with speed v as shown in the figure. The magnitude of change in velocity in moving from P to Q is
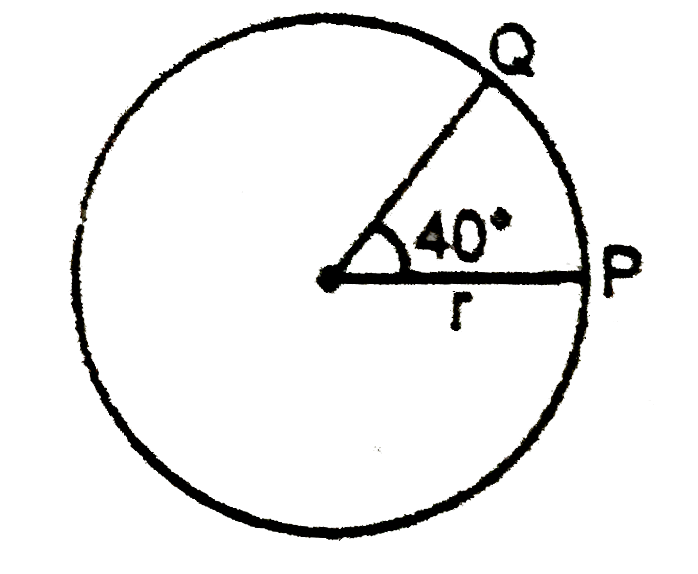 A2vcos40∘B2vsin40∘C2vcos20∘D2vsin20∘
A2vcos40∘B2vsin40∘C2vcos20∘D2vsin20∘A particle is moving an a cirle of radius 'r' and centre O with constant speed . What is the change in velocity in moving from A to B ?
(If any angle between two velocity vector is θ.)A2vsin2θ/2B2vsinθ/2C2vsin2θ/2D2vcosθ/2
A particle is moving in a circle of radius r with speed v as shown in the figure. The magnitude of change in velocity in moving from P to Q is
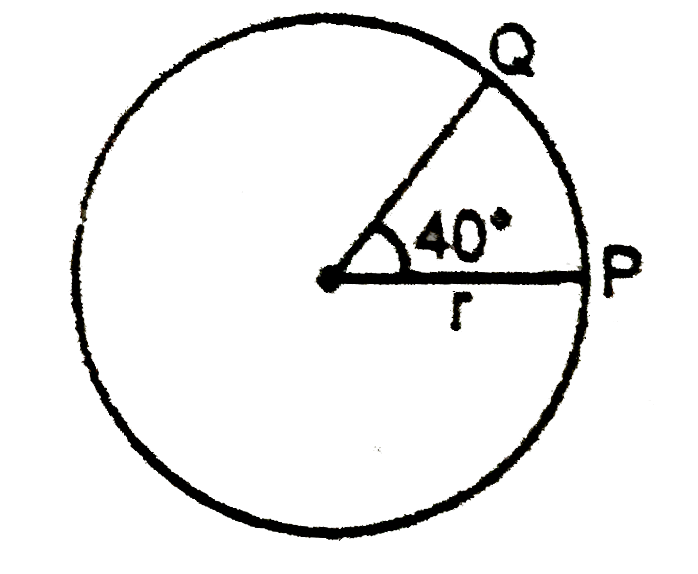
View SolutionIf the body is moving in a circle of radius r with a constant speed v , its angular velocity is
View SolutionA particle is moving on a circular path with a constant speed 'v'. Its change of velocity as it moves from A to B is:

View SolutionA particle is moving in a circle with a constant speed. Its moving is
View SolutionA particle is moving on a circular path of radius R with constant speed v. During motion of the particle form point A to point B

View Solution
