The phase difference between the instantaneous Velocity and acceleration of a particle executing simple harmonic motion is

A

$0.5 π$

B

$π$

C

$0.707 π$

D

Zero

Video Solution

Text Solution

Verified by Experts

The correct Answer is:A

The displacement equation of particle executing SHM is
$x = a cos (ω t + ϕ)$ …(i)
Velocity, $v = \frac{d x}{d t} = - a ω sin (ω + ϕ)$ ….(ii)
Acceleration,

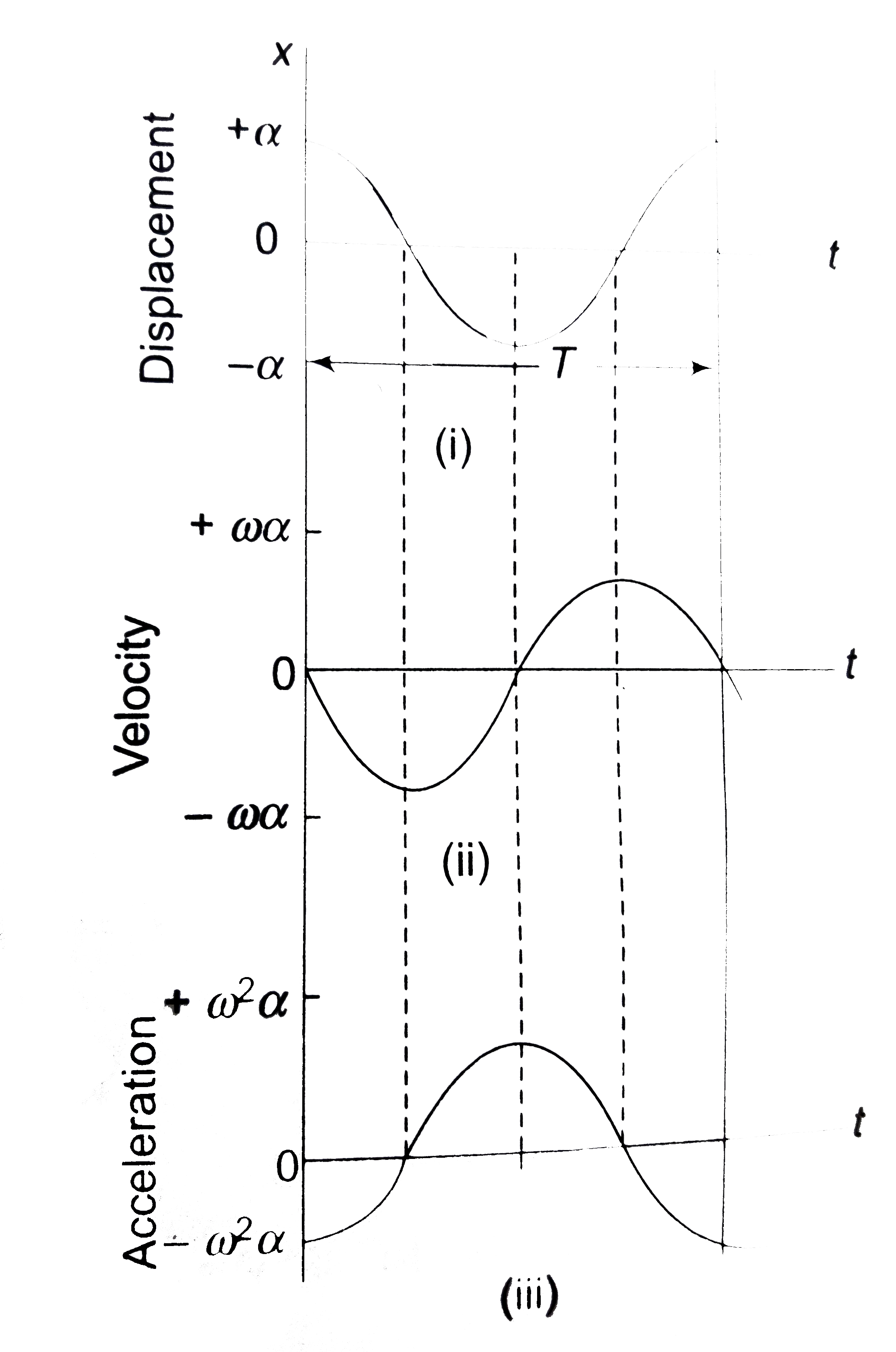
$A = \frac{d v}{d t} = - a ω^{2} cos (ω t + ϕ)$ ….(iii)
Fig is a plot of Eq.(i) with $ϕ = 0$ . Fig.shows Eq.(ii) also with $ϕ = 0$ . Fig(iii) is a plot of Eq.(iii). It should be noted that in the figures the curve of $v$ is shifted (to the left) from the curve of x by one-quarter period $(\frac{1}{4} T)$ . Similarly, the acceleration curve of A is shifted (to the left) by $\frac{1}{4} T$ relative to thevelocity curve $v$ . This implies that Velocity is $90^{\circ} (0.5 π)$ out phase with the displacement and the acceleration is $90^{\circ} (0.5 π)$ out phase with the velocity but $180^{\circ} (π)$ out of phase with displacement.

Updated on:21/07/2023

|

Class 11 PHYSICS MOCK TEST

Topper's Solved these Questions

Similar Questions

Knowledge Check

The pahse difference between the instantaneous veliocity and acceleration of a particle executing simple harmonic motion is
A $0.5 π$
B $π$
C $0.707 π$
Dzero
The phase difference between the displacement and acceleration of a particle executing simple harmonic motion is
Azero
B $π / 2$
C $π$
D $2 π$
The velocity of a particle executing simple harmonic motion is
A $ω^{2} \sqrt{A^{2} + x^{2}}$
B $ω \sqrt{A^{2} - x^{2}}$
C $ω \sqrt{A^{2} + x^{2}}$
D $ω^{2} \sqrt{A^{2} - x^{2}}$

A2Z-MOCK TEST-Motion With Constant Acceleration

Home
Ask Doubt
Profile