The phase of a particle executing simple harmonic motion is π2 when it has
Maximum velocity
Maximum acceleration
Maximum energy
Maximum displacement
The correct Answer is:B, D
To solve the problem regarding the phase of a particle executing simple harmonic motion (SHM) when it is at π2, we can follow these steps:
Step 1: Understand the Equation of SHM
The general equation for the displacement x of a particle in SHM is given by:
x(t)=Asin(ωt+ϕ)
where:
- A is the amplitude,
- ω is the angular frequency,
- t is the time,
- ϕ is the phase constant.
Step 2: Set the Phase to π/2
Given that the phase is π/2, we can set:
ωt+ϕ=π2
Assuming the phase constant ϕ=0 for simplicity, we have:
ωt=π2
Step 3: Solve for Time t
From the equation ωt=π2, we can solve for t:
t=π2ω
Step 4: Relate Angular Frequency to Time Period
The angular frequency ω is related to the time period T by the equation:
ω=2πT
Substituting this into our equation for t:
t=π2⋅2πT=T4
Step 5: Interpret the Result
The result t=T4 indicates that the particle has traveled for one-fourth of its time period. In the context of SHM, this means that at t=T4, the particle is at its maximum displacement (amplitude A).
Step 6: Conclusion
Thus, when the phase of a particle executing SHM is π/2, it is at its maximum displacement (amplitude).
Final Answer
The phase of a particle executing simple harmonic motion is π/2 when it has reached its maximum displacement (amplitude).
---
The general equation for the displacement x of a particle in SHM is given by:
x(t)=Asin(ωt+ϕ)
where:
- A is the amplitude,
- ω is the angular frequency,
- t is the time,
- ϕ is the phase constant.
Given that the phase is π/2, we can set:
ωt+ϕ=π2
Assuming the phase constant ϕ=0 for simplicity, we have:
ωt=π2
From the equation ωt=π2, we can solve for t:
t=π2ω
The angular frequency ω is related to the time period T by the equation:
ω=2πT
Substituting this into our equation for t:
t=π2⋅2πT=T4
The result t=T4 indicates that the particle has traveled for one-fourth of its time period. In the context of SHM, this means that at t=T4, the particle is at its maximum displacement (amplitude A).
Thus, when the phase of a particle executing SHM is π/2, it is at its maximum displacement (amplitude).
The phase of a particle executing simple harmonic motion is π/2 when it has reached its maximum displacement (amplitude).
Topper's Solved these Questions
- SIMPLE HARMONIC MOTIONBook:ERRORLESS Chapter:SIMPLE HARMONIC MOTIONExercise:velocity of simple Harmonic motion
Explore 27 Videos
- SIMPLE HARMONIC MOTIONBook:ERRORLESS Chapter:SIMPLE HARMONIC MOTIONExercise:Acceleration of simple Harmonic motion
Explore 21 Videos
- ROTATIONAL MOTIONBook:ERRORLESS Chapter:ROTATIONAL MOTIONExercise:Practice Problems (Problems based on motion of connected mass)
Explore 10 Videos
- SURFACE TENSIONBook:ERRORLESS Chapter:SURFACE TENSIONExercise:Exercise
Explore 214 Videos
Similar Questions
Knowledge Check
The velocity of a particle executing simple harmonic motion is
Aω2√A2+x2Bω√A2−x2Cω√A2+x2Dω2√A2−x2While a particle executes linear simple harmonic motion
Aits linear velocity and acceleration pass through their maximum and minimum values once in each oscillation.BIts linear velocity and acceleration pass through their maximum and minimum values twice in each oscillation.Cits linear velocity and acceleration pass through their maximum and minimum values four times in each oscillation.Dits linear velocity and acceleration always attain their peak values simlataneaously.The displacement of a particle executing simple harmonic motion is given by
y=A0+Asinωt+Bcosωt
Then the amplitude of its oscillation is given by :AA0+√A2+B2B√A2+B2C√A20+(A+B)2DA+B
Draw displacement-time, velocity-time and acceleration-time graphs for a particle executing simple harmonic motion. Discuss their phase relationship.
View SolutionThe displacement-time graph of a particle executing simple harmonic motion is shown in the figure
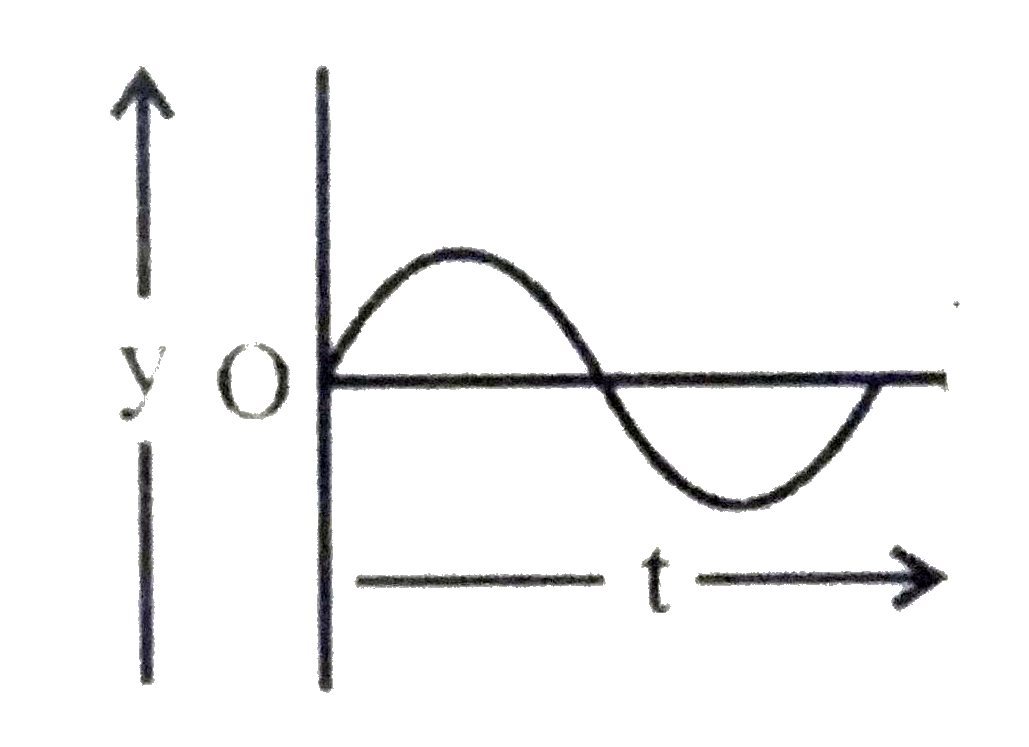
Which one of the following graphs is the correct forcetime graph for the motion of the particle ?
View SolutionThe displacement of a particle executing simple harmonic motion is given by
x=3sin(2πt+π4)
where x is in metres and t is in seconds. The amplitude and maximum speed of the particle is
View SolutionThe total mechanical energy of a particle executing simple harmonic motion is E when the displacement is half the amplitude of its kinetic energy will be
View SolutionWhen the displacement of a particle executing simple harmonic motion is half its amplitude, the ratio of its kinetic energy to potential energy is
View Solution